If the wind is strong, kites are flown.
If the sky is not clear, kites are not flown.
If the temperature is high, kites are flown. assume the statement above to be true ,if kites are flown . which of the following statement definitely true
I. The wind is strong.
II. The sky is clear.
III. The temperature is not high.

A

I only

B

II only

C

III only

D

I and III only

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

This is a problem involving deductive arguments. Assume that the initial statements are true. Then test each choice. I is not necessarily true. For example, even though it is true that when it is raining, there are clouds in the sky, you cannot conclude from the fact that there are clouds in the sky that it is raining. III makes the same error. II, however, is inferable. It.s like reasoning as follows: When there are no clouds in the sky, it is not raining. It is raining, therefore, there are clouds in the sky.

Updated on:21/07/2023
|
Class 14ENGLISHEVALUATING INFERENCES

Similar Questions

Knowledge Check

  • (i)M is the brother of N
    (ii) B is the brother of N,and
    (iii)M is the brother of D, which of the following statements is definitely true?

    AN is the brother of B
    BN is the brother of D
    CM is the brother of B
    DD is the brother of M

  • In the above Maxwellian plot at two different temperatures which of the following statements may be true?
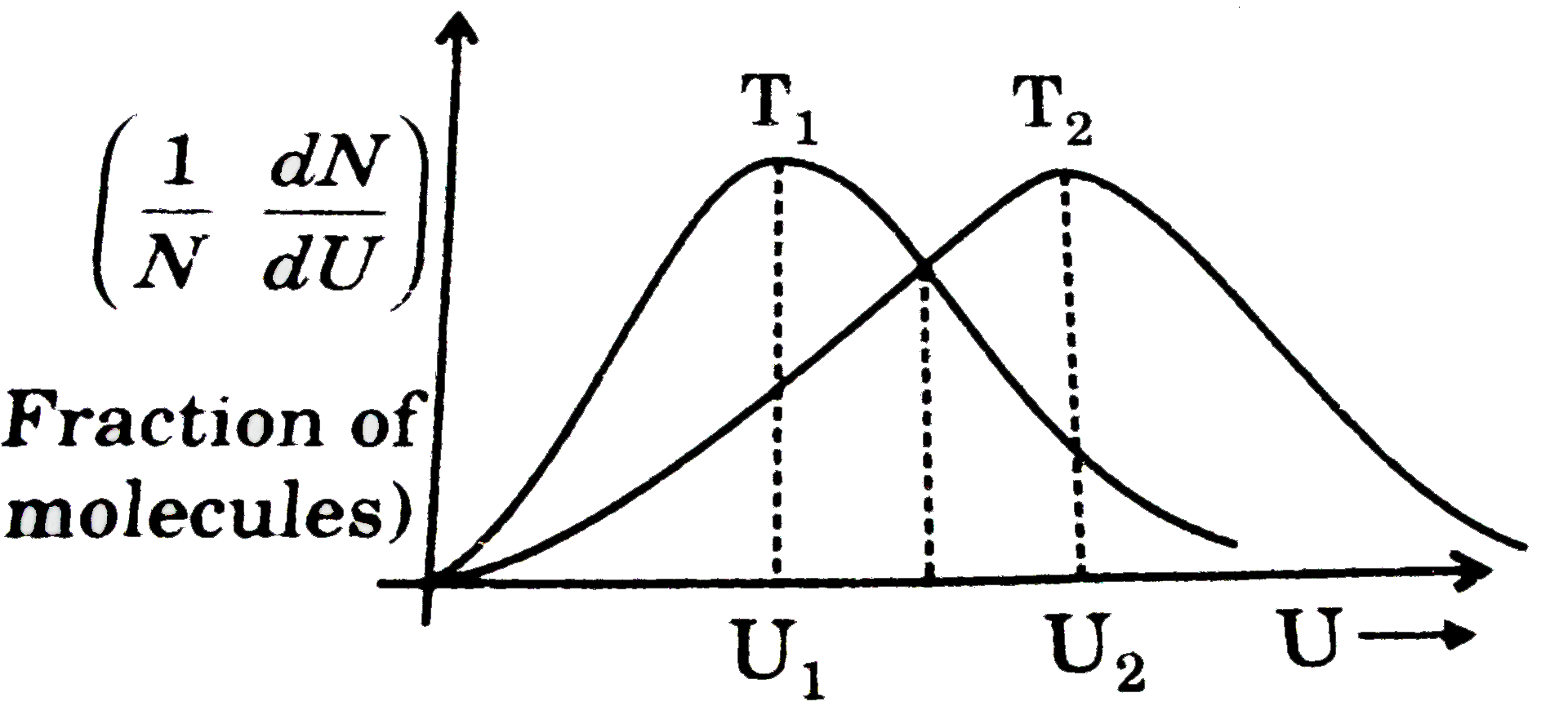    ocr_image

    AArea under the two plots is the same
    BFraction of molecules with speed U1>U2
    CU2>U1andT2>T1
    DUMPS at T1<URMS at T2,URMS at T1<URMS at T2

  • In the given questions assuming the given statements to be true, find which of the following conclusion I and II given below is/are definitely true and give your answer accordingly.
    Statements:
    M < D, D = K, K ≤ R, R= F
    Conclusions:
    I. F < K
    II. D = F

    AIf, I is true
    BIf, II is true
    CIf, either I or II is true
    DIf, neither I nor II is true