Will the median class and modal class of grouped data always be different? Justify your answer.
To determine whether the median class and modal class of grouped data are always different, we can analyze the definitions and properties of both concepts and provide an example to illustrate our findings.
1. Understanding Median Class and Modal Class:
- The median class is the class interval where the median lies. It is determined by finding the cumulative frequency and identifying the class where the cumulative frequency exceeds N/2, where N is the total frequency.
- The modal class is the class interval with the highest frequency. It is simply the class that contains the most data points.
2. Analyzing the Relationship:
- The median class and modal class can be different or the same depending on the distribution of the data. This means that it is not a strict rule that they must always differ.
3. Example:
- Consider the following frequency distribution:
| Class Interval | Frequency (F) |
|----------------|---------------|
| 100 - 120 | 12 |
| 120 - 140 | 14 |
| 140 - 160 | 8 |
| 160 - 180 | 6 |
| 180 - 200 | 10 |
4. Calculating Cumulative Frequency:
- Cumulative Frequency (CF):
- For 100 - 120: CF = 12
- For 120 - 140: CF = 12 + 14 = 26
- For 140 - 160: CF = 26 + 8 = 34
- For 160 - 180: CF = 34 + 6 = 40
- For 180 - 200: CF = 40 + 10 = 50
5. Finding the Median Class:
- Total frequency N=50
- N/2=25
- The median class is the first class where the cumulative frequency exceeds 25, which is the class interval 120 - 140.
6. Finding the Modal Class:
- The highest frequency is 14, which corresponds to the class interval 120 - 140.
- Therefore, the modal class is also 120 - 140.
7. Conclusion:
- In this example, both the median class and modal class are the same (120 - 140). This shows that the statement "the median class and modal class of grouped data will always be different" is incorrect.
Final Justification:
The median class and modal class can be the same in certain distributions, as demonstrated in the example above. Therefore, it is not accurate to say they will always be different.
---
- The median class is the class interval where the median lies. It is determined by finding the cumulative frequency and identifying the class where the cumulative frequency exceeds N/2, where N is the total frequency.
- The modal class is the class interval with the highest frequency. It is simply the class that contains the most data points.
- The median class and modal class can be different or the same depending on the distribution of the data. This means that it is not a strict rule that they must always differ.
- Consider the following frequency distribution:
|----------------|---------------|
| 100 - 120 | 12 |
| 120 - 140 | 14 |
| 140 - 160 | 8 |
| 160 - 180 | 6 |
| 180 - 200 | 10 |
- Cumulative Frequency (CF):
- For 100 - 120: CF = 12
- For 120 - 140: CF = 12 + 14 = 26
- For 140 - 160: CF = 26 + 8 = 34
- For 160 - 180: CF = 34 + 6 = 40
- For 180 - 200: CF = 40 + 10 = 50
- Total frequency N=50
- N/2=25
- The median class is the first class where the cumulative frequency exceeds 25, which is the class interval 120 - 140.
- The highest frequency is 14, which corresponds to the class interval 120 - 140.
- Therefore, the modal class is also 120 - 140.
- In this example, both the median class and modal class are the same (120 - 140). This shows that the statement "the median class and modal class of grouped data will always be different" is incorrect.
The median class and modal class can be the same in certain distributions, as demonstrated in the example above. Therefore, it is not accurate to say they will always be different.
Topper's Solved these Questions
- BOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - NCERT EXEMPLAR (Exercise - 13.3)19videos
STATISTICS
- BOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - NCERT EXEMPLAR (Exercise - 13.4)14videos
STATISTICS
- BOOK - OSWAL PUBLICATIONCHAPTER - STATISTICSEXERCISE - NCERT EXEMPLAR (Exercise - 14.1)11videos
STATISTICS
- BOOK - OSWAL PUBLICATIONCHAPTER - SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES) EXERCISE - CASE-BASED MCQs15videos
SOME APPLICATIONS OF TRIGONOMETRY (HEIGHTS AND DISTANCES)
- BOOK - OSWAL PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - BOARD CORNER (LONG ANSWER TYPE QUESTIONS)9videos
SURFACE AREAS AND VOLUMES
Similar Questions
Will the median class and modal class of grouped data always by different? Justify your answer.
View SolutionIt is true to say that the mean, mode and median of grouped data always be different? Justify your answer.
View SolutionIs it trun to say that the mean, mode and median of group data will always be differnet? Justify your answer.
View SolutionThe median and modal classes of the following data :
 View Solution
View SolutionIs inclusion of a subset in another, in the context of a universal set, an equivalence relation in the class of subsets of the sets? Justify your answer.
View SolutionThe Median class of the data given below is ________.
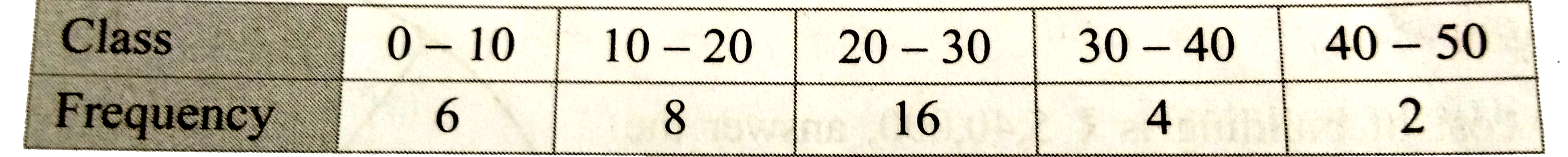 View Solution
View SolutionWrite the median class of the following distribution:
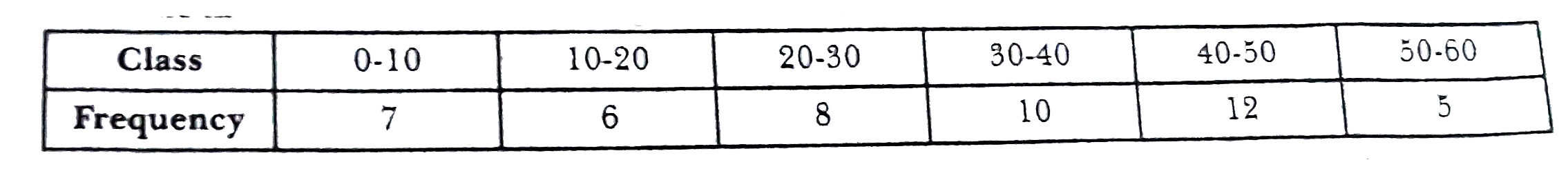 View Solution
View SolutionWill 35Cl and 37Cl have different valencies? Justify your answer.
View SolutionWill 35Cl and 37Cl have different valencies? Justify your answer.
View SolutionIn Calculating the mean of grouped data, grouped in classes of equal width, we may use the formula
¯x=a+∑fidi∑fi
Where, a is the assumed mean, a must be one of the mid point of the classes. Is the last statement correct? Justify your answer.View Solution

