The height and radius of the cone of which the frustum is a part are h1 units and r1 units respectively. Height of the frustum is h2 units and radius of the smaller base is r2 units. If h2:h1=1:2 then r2:r1 is
1:3
1:2
None of these
3:1
The correct Answer is:B
Topper's Solved these Questions
- MENSURATIONBOOK - NCERT TAMILCHAPTER - MENSURATIONEXERCISE - Unit Exercise - 7
10 Videos
- MENSURATIONBOOK - NCERT TAMILCHAPTER - MENSURATIONEXERCISE - Exercise 7.4
8 Videos
- GEOMETRYBOOK - NCERT TAMILCHAPTER - GEOMETRYEXERCISE - UNIT EXERCISE - 4
5 Videos
- NUMBERS AND SEQUENCES BOOK - NCERT TAMILCHAPTER - NUMBERS AND SEQUENCES EXERCISE - Unit Exercise - 2
10 Videos
Similar Questions
The height and radius of the cone of which the frustum is a part are h1 and r1 respectively. If h2 and r2 are the heights and radius of the smaller base of the frustum respectively and h2 :h1=1 :2 , then r2 :r1 is equal to (a) 1 :3 (b) 1 :2 (c) 2 :1 (d) 3 :1
View SolutionThe height and radius of the cone of which the frustum is a part are h1 and r1 respectively. If h2 and r2 are the heights and radius of the smaller base of the frustum respectively and h2 :h1=1 :2 , then r2 :r1 is equal to (a) 1 :3 (b) 1 :2 (c) 2 :1 (d) 3 :1
View Solution
Knowledge Check
The radius of base and curved surface area of a right cylinder is 'r' units and 4πrh square units respectively. The height of the cylinder is :
Ah2 unitsBh unitsC2 h unitsD4 h unitsIf h is the height, I the slant height and r1,r2 radii of the circular bases of the frustum of a cone, then slant height of the frustum = √(r1−r2)2+h2. Find the height of the cone of which the frustum is a part = (hr1/(r1−r2).We have a bucket in the form of frustum of a cone in which h = 8 cm, r1 = 9 cm and r2 = 3 cm.
A12 cmB9 cmC11 cmD8 cmIf h is the height, I the slant height and r1,r2 radii of the circular bases of the frustum of a cone, then slant height of the frustum = √(r1−r2)2+h2. Height of the cone of which the frustum is a part = (hr1/(r1−r2).We have a bucket in the form of frustum of a cone in which h = 8 cm, r1 = 9 cm and r2 = 3 cm. Find its volume.
A321πcm3B312πcm3C108πcm3D324πcm3
The volume of the frustum of a cone is 13πh[r21+r22−r1r2], where h is vertical height of the frustum and r1,r2 are the radii of the ends.
View SolutionThe volume of the frustum of a cone is 13πh[r21+r22−r1r2], where h is vertical height of the frustum and r1,r2 are the radii of the ends.
View SolutionWhat is volume of the frustum of a cone with height h and radii r1,r2 ?
View SolutionA frustum is cut from a right circular cone. The two circular faces have radii R and 2R and their centers are at O1 and O2 respectively. Height of the frustum is h=3R. When a point charge Q is placed at O1, the flux of electric field through the circular face of radius 2R is ϕ1 and when the charge Q is placed at O2, the flux through the other circular face is ϕ2. find the ratio ph1ϕ2
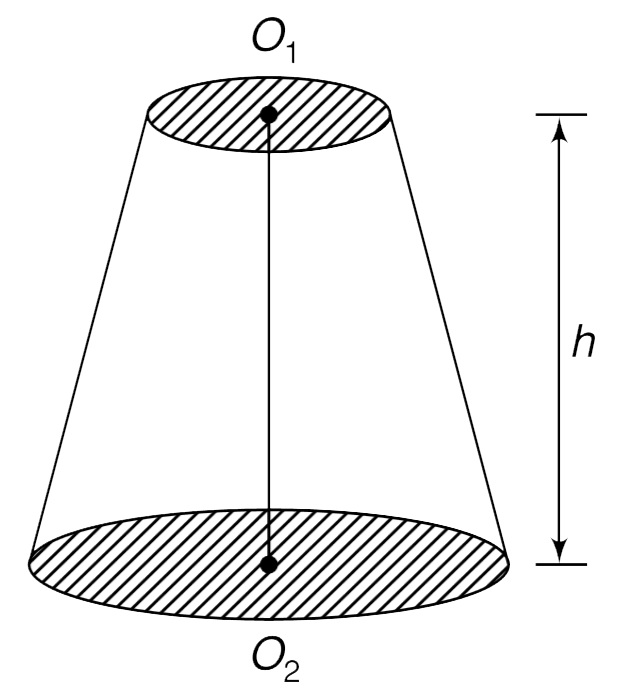
View SolutionIf h is the height, I the slant height and r1,r2 radii of the circular bases of the frustum of a cone, then slant height of the frustum = √(r1−r2)2+h2. Height of the cone of which the frustum is a part = (hr1/(r1−r2).We have a bucket in the form of frustum of a cone in which h = 8 cm, r1 = 9 cm and r2 = 3 cm.
View Solution
