The shadow of a candle fell on the table and made a right angled triange with an angle of elevtion of 60∘ from the surface of the table to the upper tip of the flame of the candle. If the length of the candle is 21 cm, how long is the tip of the shadow on the table away from the base of candle?
2√3m
4√3m
√32m
7√3m
To solve the problem step-by-step, we can follow these steps:
Step 1: Understand the Problem
We have a candle that is 21 cm tall, and the shadow it casts on the table forms a right-angled triangle with the angle of elevation from the tip of the shadow to the top of the candle being 60 degrees. We need to find the length of the shadow on the table.
Step 2: Identify the Triangle Components
In the right triangle formed:
- The height of the candle (21 cm) is the opposite side.
- The length of the shadow on the table is the adjacent side.
- The angle of elevation is 60 degrees.
Step 3: Use the Tangent Function
The tangent of an angle in a right triangle is defined as the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(60∘)=Height of the candleLength of the shadow
Substituting the known values:
tan(60∘)=21Length of the shadow
Step 4: Find the Value of tan(60∘)
From trigonometric values, we know:
tan(60∘)=√3
So, we can rewrite the equation:
√3=21Length of the shadow
Step 5: Solve for the Length of the Shadow
Rearranging the equation to find the length of the shadow:
Length of the shadow=21√3
Step 6: Rationalize the Denominator
To simplify 21√3, we multiply the numerator and denominator by √3:
Length of the shadow=21√33=7√3 cm
Final Answer
The length of the shadow on the table is 7√3 cm.
---
We have a candle that is 21 cm tall, and the shadow it casts on the table forms a right-angled triangle with the angle of elevation from the tip of the shadow to the top of the candle being 60 degrees. We need to find the length of the shadow on the table.
In the right triangle formed:
- The height of the candle (21 cm) is the opposite side.
- The length of the shadow on the table is the adjacent side.
- The angle of elevation is 60 degrees.
The tangent of an angle in a right triangle is defined as the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(60∘)=Height of the candleLength of the shadow
Substituting the known values:
tan(60∘)=21Length of the shadow
From trigonometric values, we know:
tan(60∘)=√3
So, we can rewrite the equation:
√3=21Length of the shadow
Rearranging the equation to find the length of the shadow:
Length of the shadow=21√3
To simplify 21√3, we multiply the numerator and denominator by √3:
Length of the shadow=21√33=7√3 cm
The length of the shadow on the table is 7√3 cm.
Similar Questions
If a candle of length 10 cm is placed 2 m away from the front of a plane mirror, find the length of the image of the candle formed in the mirror. Also, find the distance of the image from the object (candle).
View SolutionA candle flame 1.6 cm high is imaged in a ball bearing of diameter 0.4 cm. If the ball bearing is 20 cm away from the flame, find the location and the height of the image.
View Solution
Knowledge Check
- Question 1 - Select One
The angle of elevation of the tip of a tower from a point on the ground is 45∘.Moving 21 m directly towards the base of the tower,the angle of elevation changes to 60∘. What is the height of the tower, to the nearest metre ?
A48 mB49 mC50 mD51m - Question 2 - Select One
A student performs an experiment on finding the focal length of a convex lens by keeping a lighted candle on one end of laboratory table, a screen on its other end and the lens between them as shown in the figure. The positions of the three are adjusted to get a sharp image of the candle flame on the screen.
If now the candle flame were to be replaced by a distant lamp on a far away electric pole, the student would be able to get a sharp image of this distant lamp on the screen by moving :
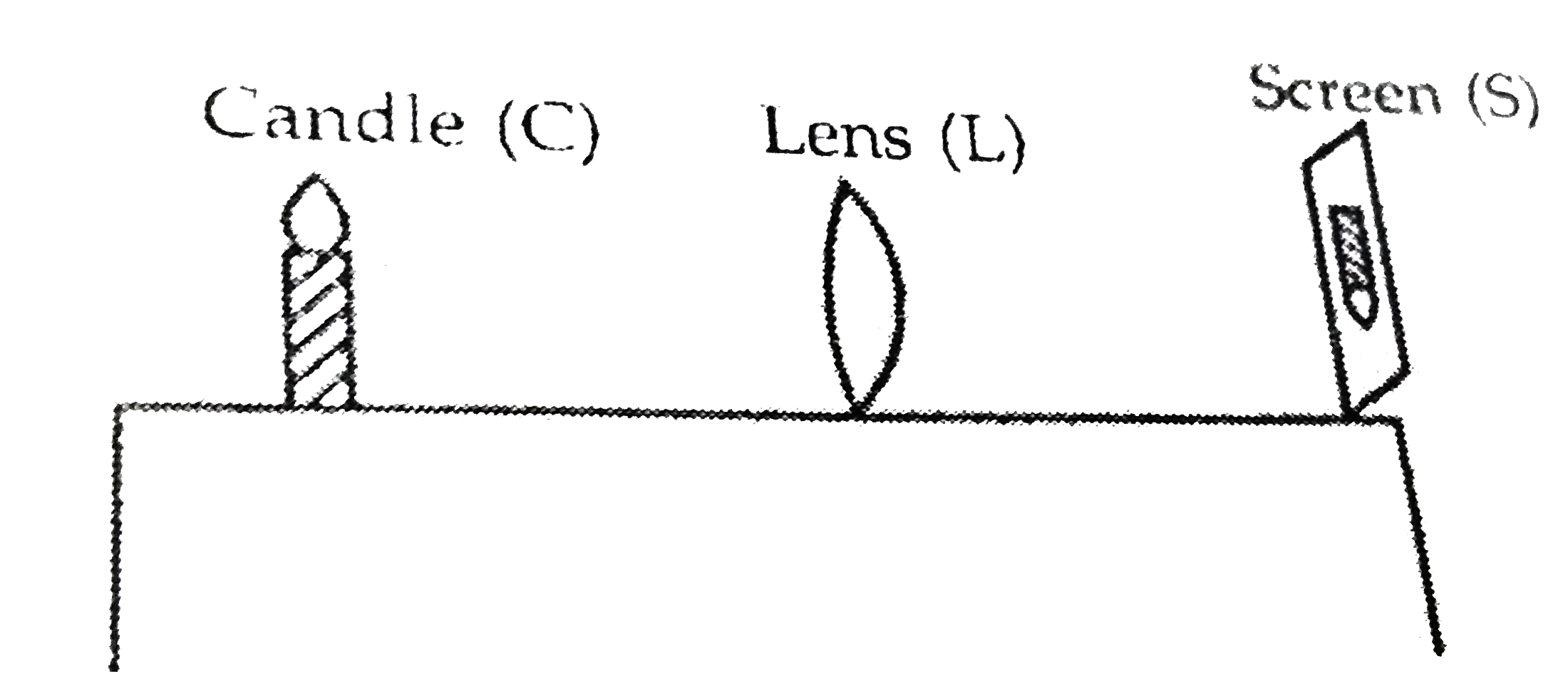 Athe screen towards of the lens or the lens towards the screen.Bthe screen towards of the lens or the lens away from the screen.Cthe screen away from the lens or the lens towards the screen.Dneither the screen nor the lens
Athe screen towards of the lens or the lens towards the screen.Bthe screen towards of the lens or the lens away from the screen.Cthe screen away from the lens or the lens towards the screen.Dneither the screen nor the lens
The image of a candle flame placed at a distance of 45 cm from a spherical lens is formed on a screen placed at a distance of 90 cm from the lens. Identify the type of lens and calculate its focal length. If the height of the flame is 2 cm, find the height of its image.
View SolutionA man 180 cm tall walks at a rate of 2m/sec. away, from a source of light that is 9 m above the ground. How fast is the length of his shadow increasing when he is 3 m away from the base of light?
View SolutionA 2 cm high candle flame is placed at a distance of 80 cm from a white screen. On placing a convex lens exactly at the mid point of the candle and the screen, a distant image of the flame is seen on the screen. What is the focal length of the lens and the size of the candle flame image formed? Draw a ray diagram to show the formation of the image in this case.
View SolutionThe image of a candle flame placed at a distance of 30 cm from a spherical lens is formed on a screen placed on the other side of the lens at a distance of 60 cm from the optical centre of the lens. Identify the type of lens and calculate its focal length. If the height of the flame is 3 cm, find the height of its image.
View SolutionThe image of a candle flame placed at a distance of 30 cm from a mirror is formed on a screen placed in front of the mirror at a distance of 60 cm from its pole. What is the nature of the mirror ? Find its focal length. If the height of the flame is 2.4 cm, find the height of its image. State whether the image formed is erect or inverted.
View SolutionA person of height 1.6m is walking away from a lamp post of height 4 m along a straight path on the flat ground. The lamp post and the person are always perpendicular to the ground. If the speed of the person is 60cms−1, the speed of the tip of the person’s shadow on the ground with respect to the person is _______ cms−1 .
View Solution

