2 moles of pure KClO3 is decomposed to an extent of 66.6%. How many moles of O2 is released?
The correct Answer is:2
To solve the problem of how many moles of O2 are released when 2 moles of pure KClO3 are decomposed to an extent of 66.6%, we can follow these steps:
Step 1: Write the decomposition reaction
The decomposition reaction of potassium chlorate (KClO3) can be represented as:
2KClO3→2KCl+3O2
Step 2: Determine the moles of KClO3 decomposed
Given that 2 moles of KClO3 are decomposed to an extent of 66.6%, we can calculate the moles decomposed:
Moles decomposed=Total moles×Extent of decomposition
Moles decomposed=2 moles×0.666=1.332 moles
Step 3: Use the stoichiometry of the reaction
From the balanced equation, we see that 2 moles of KClO3 produce 3 moles of O2. Therefore, we can set up a ratio to find out how many moles of O2 are produced from the decomposed moles of KClO3:
Moles of O2=(3 moles O22 moles KClO3)×Moles decomposed
Moles of O2=(32)×1.332=1.998 moles
Step 4: Round to appropriate significant figures
Since we started with 2 moles of KClO3, we can round our answer to 2 significant figures:
Moles of O2≈2 moles
Final Answer
The number of moles of O2 released is approximately 2 moles.
---
The decomposition reaction of potassium chlorate (KClO3) can be represented as:
2KClO3→2KCl+3O2
Given that 2 moles of KClO3 are decomposed to an extent of 66.6%, we can calculate the moles decomposed:
Moles decomposed=Total moles×Extent of decomposition
Moles decomposed=2 moles×0.666=1.332 moles
From the balanced equation, we see that 2 moles of KClO3 produce 3 moles of O2. Therefore, we can set up a ratio to find out how many moles of O2 are produced from the decomposed moles of KClO3:
Moles of O2=(3 moles O22 moles KClO3)×Moles decomposed
Moles of O2=(32)×1.332=1.998 moles
Since we started with 2 moles of KClO3, we can round our answer to 2 significant figures:
Moles of O2≈2 moles
The number of moles of O2 released is approximately 2 moles.
Similar Questions
2 moles of KClO3 is completely decomposed to produce O2. How many moles of butane can be completely burnt by the O2 gas produced
View SolutionHow many moles of 'HI' will react with
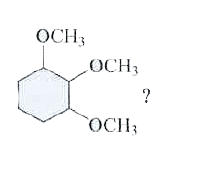
View SolutionWhen one mole of the given compond reacts with sodium metal then how many moles of H2 gas will release ?
View SolutionWhen a mixture of 10 moles of SO2 and 15 moles of O2 was passed over catalyst, 8 moles of SO3 was formed. How many moles of SO2 and O2 did not enter into combination?
View SolutionHow many moles of PbCO3 are present in whitelead ?
View SolutionA mixture of SO3,SO2 and O2 gases is maintained in a 10L flask at a temperature at which the equilibrium constant for the reaction is 100:
2SO2(g)+O2(g)⇔2SO3(g)
a. If the number of moles of SO2 and SO3 in the flask are equal. How many moles of O2 are present?
b. If the number of moles of SO3 in flask is twice the number of moles of SO2, how many moles of oxygen are present?
View Solution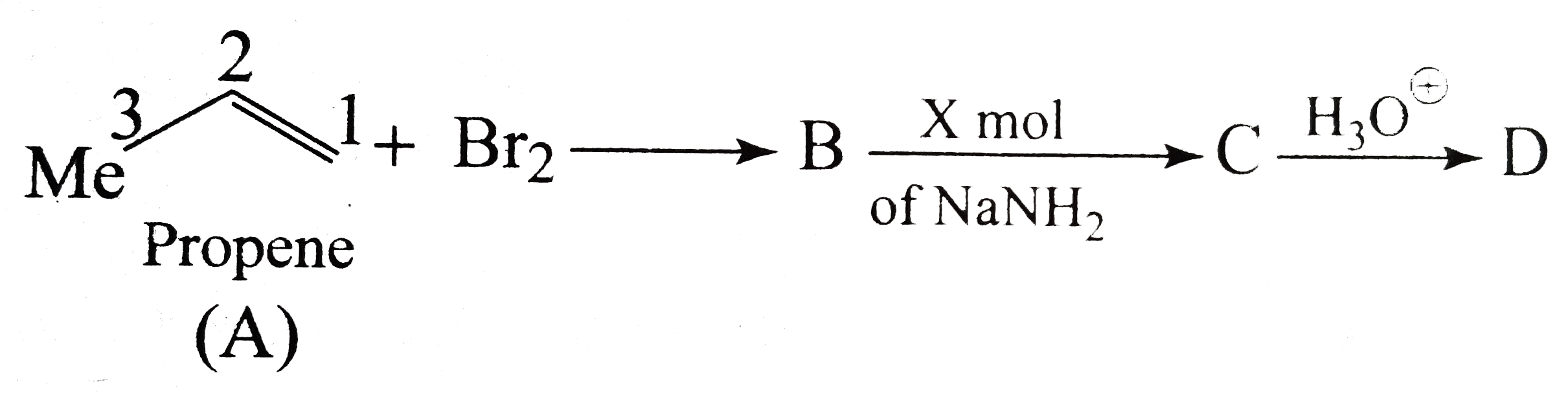
In the conversion of (B) to (C), how many moles of NaNH2 are used?
View Solution
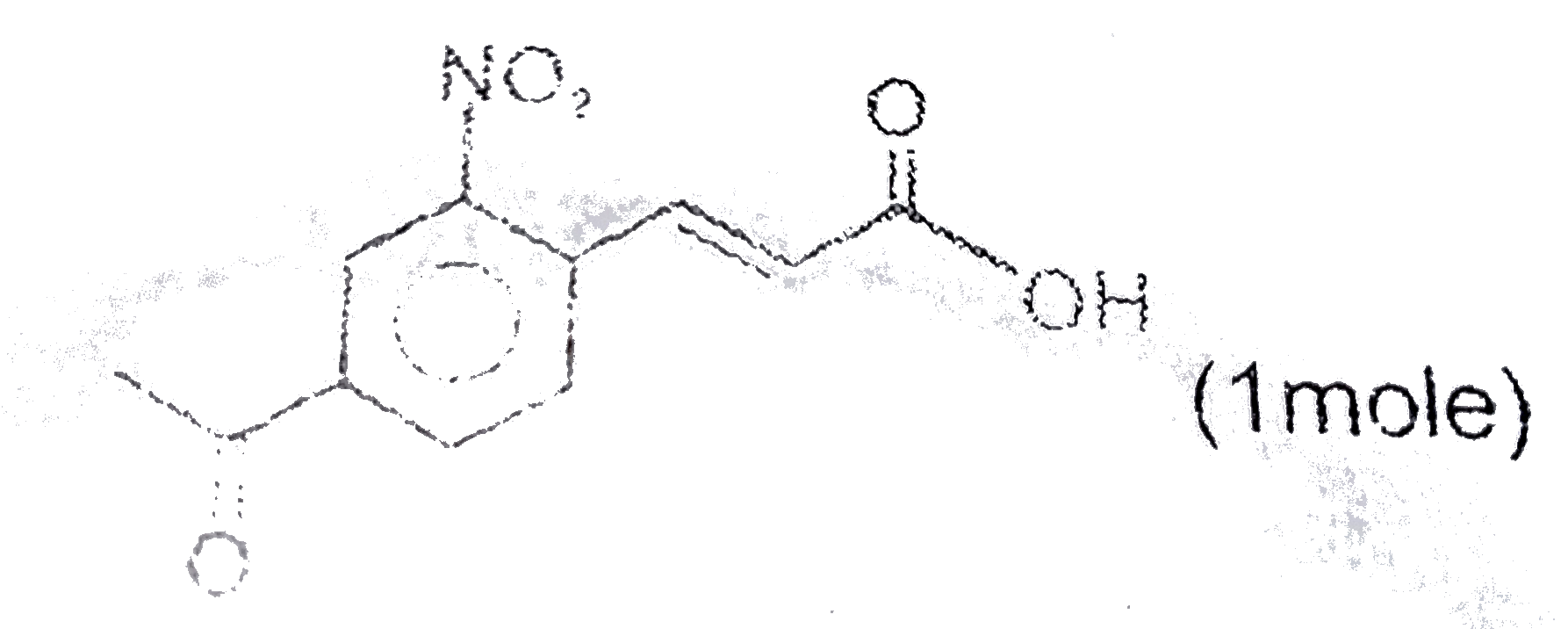
How many moles of PhNHNH2 react with 1mol(A)?
View SolutionHow many atoms are present in one mole of H2SO4 ?
View SolutionAt STP, a container has 1 mole of Ar, 2 mole of CO2, 3 moles of O2 and 4 moles of N2. Without changing the total pressure if one mole of O2 is removed, the partial pressure of O2
View Solution
