2 Its flask contains.an ideal gas at 8 atm pressure at 27°C. If some of the gas is pumped into a 5 Its flask at 127°C where 2 atm pressure developed. What is the resultant pressure in the first flask 1
4.29 atm
16.2 atm
4 atm
3.02 atm
To solve the problem step by step, we will use the ideal gas law, which is given by the equation:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles
- R = Ideal gas constant (0.0821 L·atm/(K·mol))
- T = Temperature in Kelvin
Step 1: Calculate the number of moles in the first flask.
Given:
- Pressure P1=8atm
- Volume V1=2L
- Temperature T1=27∘C=300K
Using the ideal gas law:
n1=P1V1RT1=8atm×2L0.0821L⋅atm/(K⋅mol)×300K
Calculating n1:
n1=1624.63≈0.649moles
Step 2: Calculate the number of moles in the second flask.
Given:
- Pressure P2=2atm
- Volume V2=5L
- Temperature T2=127∘C=400K
Using the ideal gas law:
n2=P2V2RT2=2atm×5L0.0821L⋅atm/(K⋅mol)×400K
Calculating n2:
n2=1032.84≈0.304moles
Step 3: Calculate the remaining moles in the first flask after gas is transferred.
The number of moles remaining in the first flask nremaining:
nremaining=n1−n2=0.649−0.304=0.345moles
Step 4: Calculate the resultant pressure in the first flask.
Using the ideal gas law again for the first flask:
Presultant=nremainingRT1V1=0.345moles×0.0821L⋅atm/(K⋅mol)×300K2L
Calculating Presultant:
Presultant=8.4872≈4.2435atm
Final Result
The resultant pressure in the first flask is approximately 4.29 atm.
---
- P = Pressure
- V = Volume
- n = Number of moles
- R = Ideal gas constant (0.0821 L·atm/(K·mol))
- T = Temperature in Kelvin
- Pressure P1=8atm
- Volume V1=2L
- Temperature T1=27∘C=300K
- Pressure P2=2atm
- Volume V2=5L
- Temperature T2=127∘C=400K
Similar Questions
Knowledge Check
2g of H2 and 17g of NH3 are placed in a 8.21 litre flask at 27∘C. The total pressure of the gas mixture is?
A4 atmB5 atmC6 atmD3 atm.What is the density of CO2 at 27.∘C and 2.5 atm pressure ?
A5.2gL−1B6.2gL−1C7.3gL−1D4.46gL−1
At 27∘C and 8.0 atm pressure, the density of propene gas is :
View SolutionA 10 liter flask contains 0.2 mole of methane, 0.3 mole of hydrogen and 0.4 mole of nitrogen at 25∘C. What is the partial pressure of each component and what is the pressure inside the flask ?
View Solution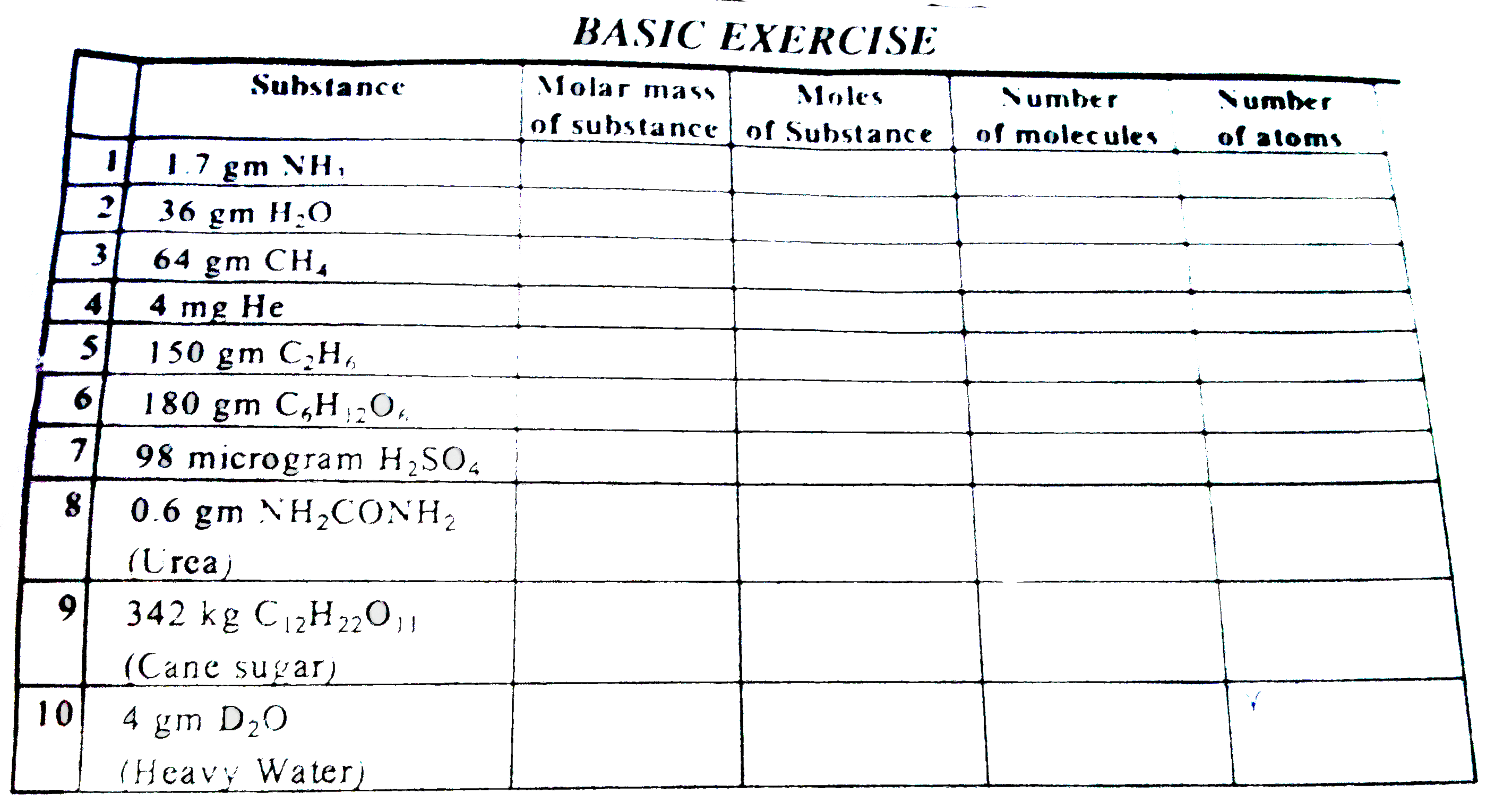
A flask of 8.21 litre contains CH4 gas at a pressure of 2 atm. Find moles of CH4 gas at 400 K?
View SolutionAn ideal gas at a pressure of 1 atm and temperature of 27∘C is compressed adiabatically until its pressure becomes 8 times the initial pressure , then final temperature is (γ=32)
View SolutionFor a given gas at 1 atm pressure, rms speed of the molecules is 200m/s at 127∘C. At 2 atm pressure and at 227∘C, the speed of the molecules will be :
View SolutionA 2 L flask containing nitrogen at 60 cm pressure is connected to a 4 L flask containing carbon monoxide at 48 cm pressure. If the temperature is kept constant, calculate the final pressure of the mixture.
View Solution