Change in enthalpy and change in internal energy are state functions. The value of ΔH,ΔU can be determined by using Kirchoff's equation.
Calculate ΔH when 10dm3 of helium at NTP is heated in a cylinder to 100∘C, assuming that the gas behave ideally.
927.9J
279.2J
729.3J
999J
The correct Answer is:A
To calculate the change in enthalpy (ΔH) when heating 10 dm³ of helium at normal temperature and pressure (NTP) to 100°C, we can follow these steps:
Step 1: Determine the number of moles of helium (N)
At NTP, the molar volume of an ideal gas is approximately 22.4 dm³/mol.
N=VolumeMolar Volume=10dm322.4dm3/mol≈0.4464mol
Step 2: Calculate the heat capacity at constant pressure (Cₚ)
For a monoatomic ideal gas like helium, the specific heat at constant volume (Cᵥ) is given by:
Cᵥ = \frac{3}{2} R
Where R is the universal gas constant (8.314 J/(mol·K)). Therefore,
Cᵥ=32×8.314≈12.471J/(mol⋅K)
Now, using the relation Cₚ = Cᵥ + R:
Cₚ=Cᵥ+R=32R+R=52R
Calculating Cₚ:
Cₚ=52×8.314≈20.785J/(mol⋅K)
Step 3: Calculate the change in temperature (ΔT)
The initial temperature (T₁) is 0°C (or 273.15 K) and the final temperature (T₂) is 100°C (or 373.15 K).
\Delta T = T₂ - T₁ = 373.15 \, \text{K} - 273.15 \, \text{K} = 100 \, \text{K}
Step 4: Calculate the change in enthalpy (ΔH)
Using the formula for enthalpy change:
ΔH=N⋅Cₚ⋅ΔT
Substituting the values we calculated:
ΔH=0.4464mol⋅20.785J/(mol⋅K)⋅100K
Calculating ΔH:
ΔH≈0.4464⋅20.785⋅100≈927.90J
Final Answer:
The change in enthalpy (ΔH) when heating 10 dm³ of helium to 100°C is approximately 927.90 J.
---
At NTP, the molar volume of an ideal gas is approximately 22.4 dm³/mol.
For a monoatomic ideal gas like helium, the specific heat at constant volume (Cᵥ) is given by:
Cᵥ = \frac{3}{2} R
The initial temperature (T₁) is 0°C (or 273.15 K) and the final temperature (T₂) is 100°C (or 373.15 K).
\Delta T = T₂ - T₁ = 373.15 \, \text{K} - 273.15 \, \text{K} = 100 \, \text{K}
Using the formula for enthalpy change:
The change in enthalpy (ΔH) when heating 10 dm³ of helium to 100°C is approximately 927.90 J.
Similar Questions
Change in enthalpy and change in internal energy are state functions. The value of ΔH,ΔU can be determined by using Kirchoff's equation.
Calculate the heat of formation of methane, given that heat of formation of water =−286kJmol−1, heat of combustion of methane =−890kJmol−1 heat of combustion of carbon =−393.5kJmol−1
View SolutionChange in enthalpy and change in internal energy are state functions. The value of ΔH,ΔU can be determined by using Kirchoff's equation.
I mole of naphthalene (C10H8) was burnt is oxygen gas at 25∘C at constant volume. The heat evolved was found to be 5138.8kJ. Calculate the heat of reaction at constant pressure
View Solution
Knowledge Check
The entropy change involved in the isothermal reversible expansion of 2 moles of an ideal gas from a volume of 10 dm3 a volume of 100 dm3 at 27∘C is
A38.3Jmol−1k−1B35.8Jmol−1K−1C32.3Jmol−1K−1D42.3Jmol−1K−1
calculate the change in internal energy of a gas kept in a rigid container when 100 J of heat is supplied to it.
View SolutionCalculate the change in internal energy of 3.0 mol of helium gas when its temperature is increased by 2.0K.
View SolutionChange in internal energy of an ideal gas is given by ΔU=nCVΔT. This is applicable for (CV=molar heat capacity at constant volume)
View SolutionThe enthalpy change for chemical reaction is denoted aas ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal energy is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal energy Δn= change in number of moles, R= gas constant.
Which of the following equations corresponds to the definition of enthalpy of formation at 298K?
View SolutionIn the given figure, an ideal gas changes it state from A to state C by two paths ABC and AC.
(i) Find the path along which work done is the least.
(ii) The internal energy of the gas at A to 10J and the amount of heat supplied to change its state to C through the path AC is 200J. Calculate the internal energy at C.
(iii) The internal energy of the gas at state B is 30J. Find the amount of heat supplied to the gas to go from A→B.

View SolutionThe enthalpy change for chemical reaction is denoted aas ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal enegry is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal enegry Δn= change in number of moles, R= gas constant.
For a reaction, 2X(s)+2Y(s)→2C(l)+D(g),ΔH at 27∘C is −28kcalmol−1.ΔU is .....kcalmol−1
View SolutionIn the given graph, an ideal gas changes its state from A to C by two paths ABC and AC.
(a) Find the path along which work done is less.
(b) The internal energy of gas at A is 10J and the amount of heat supplied in path AC is 200J. Calculate the internal energy of gas at C.
(c) The internal energy of gas at state B is 20J. Find the amount of heat supplied to the gas to go from A to B.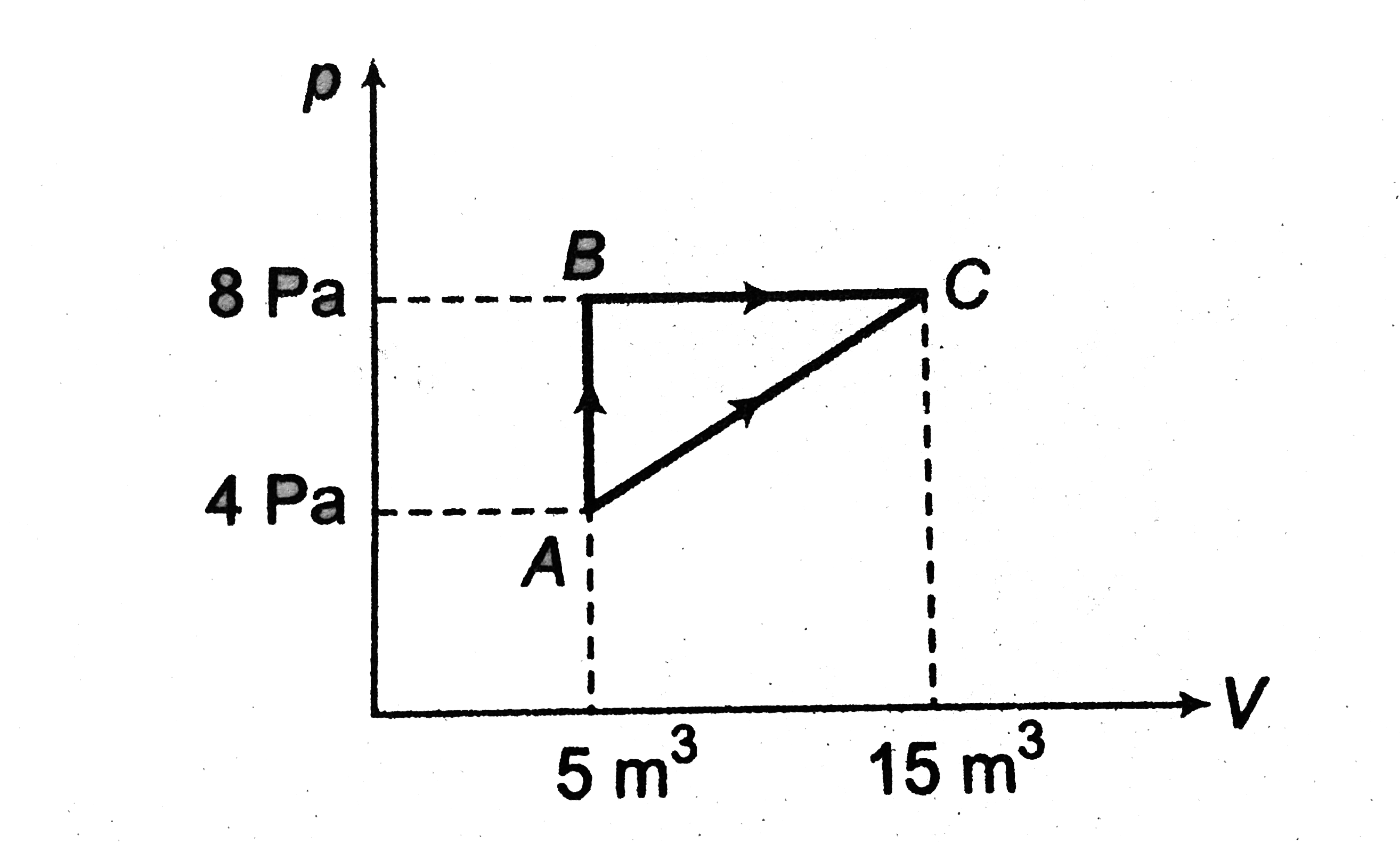
View Solution
