The driver of a car moving towards a rocket launching with a speed of 6 ms−1 observed that the rocket is moving with speed of 10 ms−1 The upward speed of the rocket as seen by the stationary observer is nearly
4ms−1
6ms−1
8ms−1
11ms−1
The correct Answer is:C
To solve the problem, we need to find the upward speed of the rocket as seen by a stationary observer, given the speed of the car and the speed of the rocket as observed by the driver in the car.
1. Identify the Given Information:
- Speed of the car (Vc) = 6 m/s (moving towards the rocket)
- Speed of the rocket as observed by the driver in the car (Vrc) = 10 m/s (upward)
2. Understand the Vector Relationship:
- The rocket is moving upwards with some speed (Vr) which we need to find.
- The car is moving towards the rocket, which means we can visualize this as a right triangle where:
- One leg represents the upward speed of the rocket (Vr).
- The other leg represents the speed of the car (Vc).
- The hypotenuse represents the speed of the rocket as observed from the car (Vrc).
3. Apply the Pythagorean Theorem:
- According to the Pythagorean theorem, we can write:
Vrc2=Vr2+Vc2
- Substituting the known values:
102=Vr2+62
4. Calculate the Squares:
- Calculate 102 and 62:
100=Vr2+36
5. Rearrange the Equation:
- To find Vr2, rearrange the equation:
Vr2=100−36
Vr2=64
6. Find the Upward Speed of the Rocket:
- Taking the square root of both sides:
Vr=√64=8 m/s
7. Conclusion:
- The upward speed of the rocket as seen by a stationary observer is 8 m/s.
Final Answer:
The upward speed of the rocket as seen by a stationary observer is 8 m/s.
- Speed of the car (Vc) = 6 m/s (moving towards the rocket)
- Speed of the rocket as observed by the driver in the car (Vrc) = 10 m/s (upward)
- The rocket is moving upwards with some speed (Vr) which we need to find.
- The car is moving towards the rocket, which means we can visualize this as a right triangle where:
- One leg represents the upward speed of the rocket (Vr).
- The other leg represents the speed of the car (Vc).
- The hypotenuse represents the speed of the rocket as observed from the car (Vrc).
- According to the Pythagorean theorem, we can write:
Vrc2=Vr2+Vc2
- Substituting the known values:
102=Vr2+62
- Calculate 102 and 62:
100=Vr2+36
- To find Vr2, rearrange the equation:
Vr2=100−36
Vr2=64
- Taking the square root of both sides:
Vr=√64=8 m/s
- The upward speed of the rocket as seen by a stationary observer is 8 m/s.
The upward speed of the rocket as seen by a stationary observer is 8 m/s.
Similar Questions
A body is projected horizontally with speed 20ms−1 . The speed of the body after 5s is nearly
View SolutionThere is a source of sound (a tuning fork) moving towards a reflecting wall will a speed of 30ms−1. The velocity of the sound in air is 330ms−1and the frequency of the tuning fork is 600 Hz. The observer is between the source and the wall and is moving with some velocity V1 towards the wall. The beat frequency heard by the observer is 4 Hz. If the tuning fork is waxed, the frequency beats heard by the observer becomes 3 Hz. If the new frequency of tuning fork is k then k/50 is
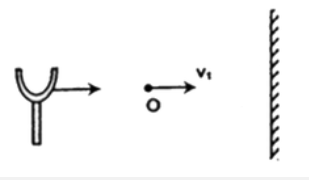
View Solution
Knowledge Check
The driver of a car moving towards a rocket launching pad with a speed of 6 m s−1 observed that the rocket is moving with speed of 10 m s−1. The upward speed of the rocket as seen by the stationery observer is nearly
A4 m s−1B6 m s−1C8 m s−1D11 m s−1
Find the de Broglie wavelength of electrons moving with a speed of 7×106ms−1
View SolutionA rocket is moving at a speed of 220 ms−1 towards a stationary target. While moving, it emits a wave of frequency 500 Hz. What is the frequency of the sound as detected by the target?
View SolutionThe driver of a car moving with a speed of 10ms−1 sees a red light ahead, applies brakes and stops after covering 10 m distance. If the same car were moving with a speed of 20ms−1 the same driver would have stopped the car after covering 30 m distance. Within what distance the car can be stopped if traveling with a velocity of 15ms−1 Assume the same reaction time and the same deceleration in each case
View SolutionA car is moving towards east with a speed of 25kmh−1. To the driver of the car, a bus appears to move towards north with a speed of 25√3kmh−1. What is the actual velocity of the bus ?
View SolutionA fish rising up vertically toward the surface of water with speed 3ms−1 observes a bird diving down vertically towards it with speed 9ms−1 . The actual velocity of bird is
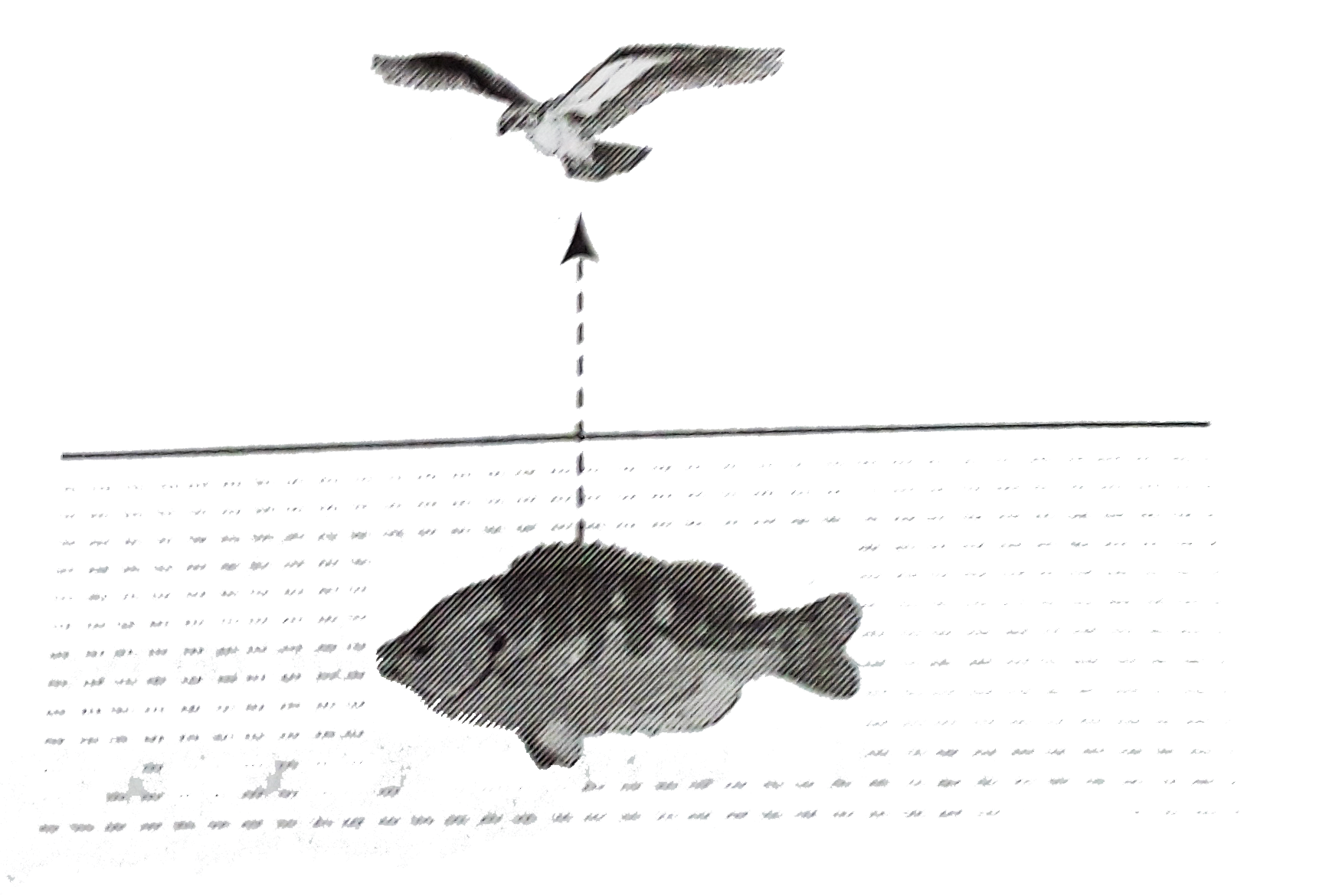
View SolutionA fish rising up vertically toward the surface of water with speed 3ms−1 observes a bird diving down vertically towards it with speed 9ms−1 . The actual velocity of bird is

View SolutionA car is moving with a speed of 30 ms−1 on a circular path of radius 500 m. If its speed is increasing at the rate of 2 ms−2 , the net acceleration of the car is
View Solution
