Two cliff of heights 120m and 100.4m are separated by a horizontal distance of 16m. If a car has to reach from the first cliff to the second, the horizontal velocity of the car should be
16ms−1
4ms−
2ms−1
8ms−1
The correct Answer is:D
To solve the problem of determining the horizontal velocity required for a car to travel from one cliff to another, we can follow these steps:
Step 1: Determine the height difference between the two cliffs
The heights of the cliffs are given as:
- Height of the first cliff (h1) = 120 m
- Height of the second cliff (h2) = 100.4 m
The height difference (Δh) is calculated as:
Δh=h1−h2=120m−100.4m=19.6m
Step 2: Calculate the time of flight
The time of flight (t) for an object falling from a height can be calculated using the formula:
t=√2hg
where:
- h is the height difference (19.6 m)
- g is the acceleration due to gravity (approximately 9.8m/s2)
Substituting the values:
t=√2×19.69.8=√39.29.8=√4=2s
Step 3: Calculate the horizontal velocity
The horizontal velocity (v) can be calculated using the formula:
v=dt
where:
- d is the horizontal distance (16 m)
- t is the time of flight (2 s)
Substituting the values:
v=16m2s=8m/s
Final Answer
The horizontal velocity of the car should be 8 m/s.
---
The heights of the cliffs are given as:
- Height of the first cliff (h1) = 120 m
- Height of the second cliff (h2) = 100.4 m
Δh=h1−h2=120m−100.4m=19.6m
The time of flight (t) for an object falling from a height can be calculated using the formula:
t=√2hg
where:
- h is the height difference (19.6 m)
- g is the acceleration due to gravity (approximately 9.8m/s2)
t=√2×19.69.8=√39.29.8=√4=2s
The horizontal velocity (v) can be calculated using the formula:
v=dt
where:
- d is the horizontal distance (16 m)
- t is the time of flight (2 s)
v=16m2s=8m/s
The horizontal velocity of the car should be 8 m/s.
Similar Questions
A body of mass m is projected horizontally with a velocity v from the top of a tower of height h and it reaches the ground at a distance x from the foot of the tower. If a second body of mass 2m is projected horizontally from the top of a tower of height 2h, it reaches the ground at a distance 2x from the foot of the tower. The horizontal veloctiy of the second body is.
View SolutionTwo vertical poles AL and BM of height 4 m and 16 m respectively stand apart on a horizontal plane. If A, B be the feet of the poles and AM and BL intersect at P, then the height of P from the horizontal plane is equal to
View Solution
Knowledge Check
A boy aims a gun at a bird from a point, at a horizontal distance of 100 m. If the gun can impart a velocity of 500 m/sec to the bullet, at what height above the bird must be aim his gun in order to hit it ?
A400 m/sB200 m/sC800 m/sD256 m/sa 100kg gun fires a ball of 1kg horizontally from a cliff of height 500m. If falls on the ground at a distance of 400m from the bottom of the cliff. The recoil velocity of the gun is (Take g: 10ms−2
A0.2ms−1B0.4ms−1C0.6ms−1D0.8ms−1A man stands in between two vertical cliffs X and Y such that he is at a distance of 660 m from X. When he explodes cracker, he hears the first echo after 4 sec and second echo 6 second later. Calculate the speed of sound in air and the distance of cliff y from the man.
A330ms−1,1650mB320ms−1 ,1400mC340ms−1, 1700mDnone of these
a 100kg gun fires a ball of 1kg horizontally from a cliff of height 500m. If falls on the ground at a distance of 400m from the bottom of the cliff. The recoil velocity of the gun is (Take g: 10ms−2
View SolutionFrom a height of 100 m, a ball is thrown horizontally with an initial speed of 15m/s. How far does it travel horizontally in the first 2 seconds ?
View SolutionShots are fired simultaneously from the top and bottom of a vertical cliff with the elevation α=30∘, beta =60∘, respectively. The shots strike an object simultaneously at the same point. If a=10(√3) m is the horizontal distance of the object from the cliff, then the height h of the cliff is
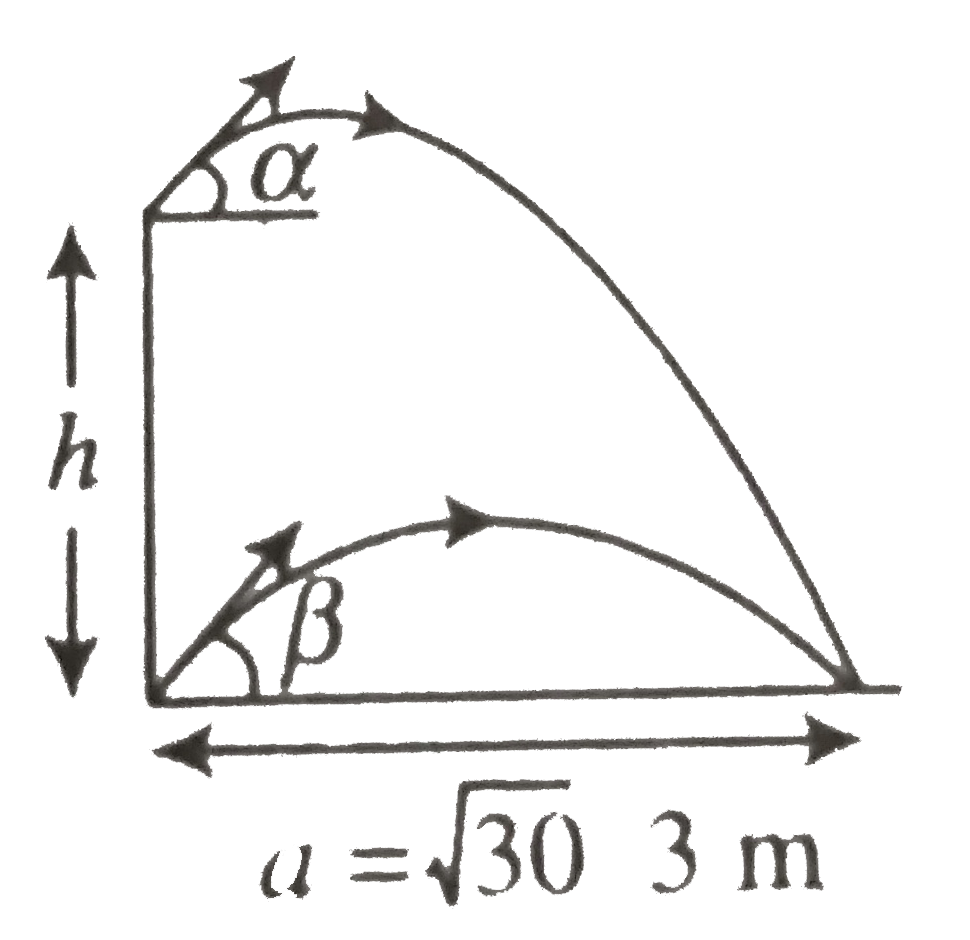
View SolutionTwo cars moving in opposite direction approach each other with speed of 22m/s and 16.5m/s respectively . The driver of the first car blows a horn having a frequency 400Hz. The frequency heard by the driver of the second car is [ velocity of the sound 340m/s]
View SolutionThe angle of elevation of the of a tower at a distance of 120 m from its foot on a horizontal plane is found to be 30∘ . Find the height of the tower.
View Solution
