If P represents radiation pressure, C speed of light, and Q radiation energy striking unit area per second and x,y,z are non zero integers, then P^x Q^y C^z is dimensionless. The values of X,y and z are respectively
1,1,-1
1,-1,1
-1,1,1
1,1,1
The correct Answer is:B
To solve the problem, we need to find the values of x, y, and z such that the expression PxQyCz is dimensionless. We will start by determining the dimensions of the quantities involved.
Step 1: Determine the dimensions of each quantity.
1. Radiation Pressure (P):
- The dimension of pressure is given by:
(P)=(F)(A)=(M)(L)(T−2)(L2)=(M)(L−1)(T−2)
2. Radiation Energy per unit area per unit time (Q):
- The dimension of energy is (M)(L2)(T−2).
- Therefore, the dimension of Q is:
(Q)=(M)(L2)(T−2)(L2)(T)=(M)(T−3)
3. Speed of Light (C):
- The dimension of speed is:
(C)=(L)(T−1)
Step 2: Write the expression for PxQyCz.
Now we can express the dimensions of PxQyCz:
(Px)=((M)(L−1)(T−2))x=(Mx)(L−x)(T−2x)
(Qy)=((M)(T−3))y=(My)(T−3y)
(Cz)=((L)(T−1))z=(Lz)(T−z)
Combining these, we have:
(PxQyCz)=(Mx+y)(L−x+z)(T−2x−3y−z)
Step 3: Set the dimensions equal to zero for a dimensionless quantity.
For the expression to be dimensionless, the exponents of M, L, and T must all equal zero:
1. x+y=0 (1)
2. −x+z=0 (2)
3. −2x−3y−z=0 (3)
Step 4: Solve the equations.
From equation (1):
y=−x
Substituting y=−x into equation (2):
−x+z=0⟹z=x
Now substituting y=−x and z=x into equation (3):
−2x−3(−x)−x=0⟹−2x+3x−x=0⟹0=0
This equation is satisfied for any x.
Step 5: Choose a value for x.
Let’s choose x=1:
- From y=−x, we get y=−1.
- From z=x, we get z=1.
Thus, the values of x, y, and z are:
x=1,y=−1,z=1
Final Answer:
The values of x, y, and z are respectively:
1,−1,1
- The dimension of pressure is given by:
(P)=(F)(A)=(M)(L)(T−2)(L2)=(M)(L−1)(T−2)
- The dimension of energy is (M)(L2)(T−2).
- Therefore, the dimension of Q is:
(Q)=(M)(L2)(T−2)(L2)(T)=(M)(T−3)
- The dimension of speed is:
(C)=(L)(T−1)
(Px)=((M)(L−1)(T−2))x=(Mx)(L−x)(T−2x)
(Qy)=((M)(T−3))y=(My)(T−3y)
(Cz)=((L)(T−1))z=(Lz)(T−z)
(PxQyCz)=(Mx+y)(L−x+z)(T−2x−3y−z)
1. x+y=0 (1)
2. −x+z=0 (2)
3. −2x−3y−z=0 (3)
y=−x
−x+z=0⟹z=x
−2x−3(−x)−x=0⟹−2x+3x−x=0⟹0=0
This equation is satisfied for any x.
- From y=−x, we get y=−1.
- From z=x, we get z=1.
x=1,y=−1,z=1
The values of x, y, and z are respectively:
1,−1,1
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -2
56 Videos
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE
45 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (INTEGER TYPE QUESTIONS)
10 Videos
Similar Questions
If P represents radiation pressure , C represents the speed of light , and Q represents radiation energy striking a unit area per second , then non - zero integers x,y,z such that PxQyCz is dimensionless , find the values of x,y,andz.
View SolutionIf P represents radiation pressure , C represents the speed of light , and Q represents radiation energy striking a unit area per second , then non - zero integers x,y,z such that PxQyCz is dimensionless , find the values of x,y,andz.
View Solution
Knowledge Check
If x,y,z are positive integers, then the value of the expression (x+y)(y+z)(z+x) is
Aa)=8xyzBb) >8xyzCc) <8xyzDd) =4xyz
If P represents radiation pressure , C represents the speed of light , and Q represents radiation energy striking a unit area per second , then non - zero integers x,y,z such that PxQyCz is dimensionless , find the values of x,y,andz.
View SolutionLet P represent radiation pressure, c represent speed of light and l represents radiation energy striking a unit area per second, then PxIycz will be dimensionless for
View SolutionIf p and q vary inversely, find the values of x,y and z.

View SolutionIf p and q vary inversely, find the values of x,y and z.

View SolutionIf x,y,z are in G.P and ax=by=cz,then
View SolutionIf x,yandz are positive integers such that x<y<zandx+y+z=6, then what is the value of z?
View SolutionIF p and q are in direct variation of x, y and z in the table given below:
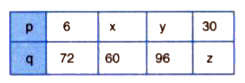
View Solution
