A force produces an acceleration of a1 in a body and the same force produces an acceleration of a2 in another body. If the two bodies are combined and the same force is applied on the combination, the acceleration produced in it is
a1+a2
a1+a2a1a2
a1a2a1+a2
√a1a2
The correct Answer is:C
To solve the problem, we need to find the acceleration produced when two bodies are combined and the same force is applied to them. Let's break it down step by step.
Step 1: Understand the relationship between force, mass, and acceleration
According to Newton's second law of motion, the force acting on an object is equal to the mass of the object multiplied by its acceleration. This can be expressed as:
F=m⋅a
Step 2: Define the forces acting on the individual bodies
Let:
- m1 be the mass of the first body,
- a1 be the acceleration of the first body when force F is applied,
- m2 be the mass of the second body,
- a2 be the acceleration of the second body when the same force F is applied.
From the definition of force, we can write:
F=m1⋅a1(1)
F=m2⋅a2(2)
Step 3: Express the masses in terms of force and acceleration
From equations (1) and (2), we can express the masses as:
m1=Fa1(3)
m2=Fa2(4)
Step 4: Combine the masses of the two bodies
When the two bodies are combined, the total mass M is:
M=m1+m2
Substituting equations (3) and (4) into this, we get:
M=Fa1+Fa2
Step 5: Factor out the force
Factoring out F, we have:
M=F(1a1+1a2)
Step 6: Apply the same force to the combined mass
Now, when the same force F is applied to the combined mass M, the acceleration a produced can be expressed as:
F=M⋅a
Substituting for M:
F=(Fa1+Fa2)⋅a
Step 7: Simplify the equation
Dividing both sides by F (assuming F≠0):
1=(1a1+1a2)⋅a
Step 8: Solve for acceleration a
Rearranging the equation gives:
a=1(1a1+1a2)
This can be simplified to:
a=a1⋅a2a1+a2
Final Answer
Thus, the acceleration produced in the combined body when the same force is applied is:
a=a1⋅a2a1+a2
---
According to Newton's second law of motion, the force acting on an object is equal to the mass of the object multiplied by its acceleration. This can be expressed as:
F=m⋅a
Let:
- m1 be the mass of the first body,
- a1 be the acceleration of the first body when force F is applied,
- m2 be the mass of the second body,
- a2 be the acceleration of the second body when the same force F is applied.
F=m1⋅a1(1)
F=m2⋅a2(2)
From equations (1) and (2), we can express the masses as:
m1=Fa1(3)
m2=Fa2(4)
When the two bodies are combined, the total mass M is:
M=m1+m2
Substituting equations (3) and (4) into this, we get:
M=Fa1+Fa2
Factoring out F, we have:
M=F(1a1+1a2)
Now, when the same force F is applied to the combined mass M, the acceleration a produced can be expressed as:
F=M⋅a
Substituting for M:
F=(Fa1+Fa2)⋅a
Dividing both sides by F (assuming F≠0):
1=(1a1+1a2)⋅a
Rearranging the equation gives:
a=1(1a1+1a2)
This can be simplified to:
a=a1⋅a2a1+a2
Thus, the acceleration produced in the combined body when the same force is applied is:
a=a1⋅a2a1+a2
Similar Questions
A force produces an acceleration of 4ms−2 in a body of mass m1 and the same force produces an acceleration of 6ms−2 in another body oa mass m2. If the same force is applied to (m1+m2), then the acceleration will be
View SolutionFind the force exerted on a mass of 20 g if the acceleration produced in it is 8m s−2
View SolutionIf a tangential force mg is applied to a disc of mass m and radius r, the angular acceleration produced in it is?
View SolutionA 10 N force is applied on a body produces an acceleration of 1 m/s2 . The mass of the body is
View SolutionA constant force of 5 N can produce an acceleration of 1 m/s2 on the body of mass m1 and 2m/s2 on the body of mass m2 . If both the masses are tied together and same force is applied then what will be the acceleration of the system ?
View SolutionWhen a same force is applied on two different objects. It produces accelerations of 4msec2 and 6msec2. In these objects, if the same force is applied on the combination of these objects, calculate acceleration of combination.
View SolutionHow much force is required to produce an acceleration of 2ms−2 in a body of mass 0.8 kg ?
View SolutionA force of 10 N acts on a body of mass 5 kg. Find the acceleration produced.
View SolutionIf 100 N force is applied to 10 kg block as shown is diagram, then the acceleration produced in lower block is
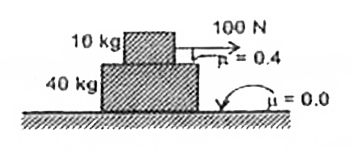
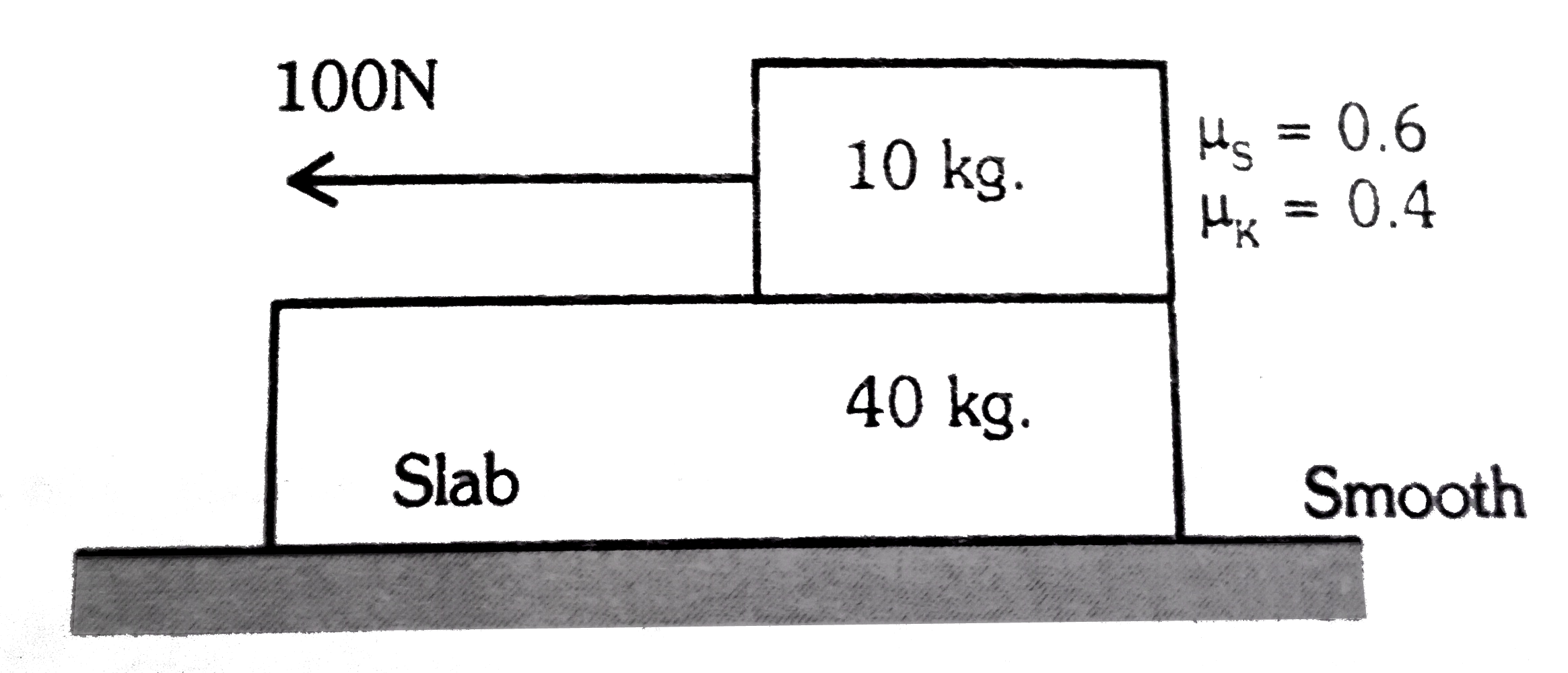
View SolutionIf 100N force is applied to 10kg. block as shown in diagram then acceleration produced for slab-
View Solution
