A block of mass'm' is resting on the floor of a lift. The coefficient of friction between the block and the floor is μ. When the lift is falling freely, the limiting frictional force between block and surface is
μ mg
μmgs
mg
zero
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the block when the lift is falling freely.
1. Understanding the Situation:
- The block of mass m is resting on the floor of a lift.
- The lift is falling freely, which means it is in free fall under the influence of gravity.
2. Identifying Forces:
- When the lift is in free fall, both the lift and the block experience the same acceleration, which is equal to g (acceleration due to gravity).
- The forces acting on the block are:
- The gravitational force mg acting downward.
- The normal force N acting upward from the floor of the lift.
3. Applying Newton's Second Law:
- According to Newton's second law, the net force acting on the block is equal to its mass times its acceleration.
- Since the lift is falling freely, the acceleration of the block is also g downward.
4. Setting Up the Equation:
- The net force acting on the block can be expressed as:
mg−N=ma
- Here, a=g (the block is falling with the lift), so we can substitute:
mg−N=mg
5. Solving for Normal Force N:
- Rearranging the equation gives:
mg−N=mg⟹N=0
- This means that the normal force N acting on the block is zero.
6. Calculating Limiting Frictional Force:
- The limiting frictional force Ff is given by:
Ff=μN
- Since N=0:
Ff=μ×0=0
Final Answer:
The limiting frictional force between the block and the surface when the lift is falling freely is 0.
- The block of mass m is resting on the floor of a lift.
- The lift is falling freely, which means it is in free fall under the influence of gravity.
- When the lift is in free fall, both the lift and the block experience the same acceleration, which is equal to g (acceleration due to gravity).
- The forces acting on the block are:
- The gravitational force mg acting downward.
- The normal force N acting upward from the floor of the lift.
- According to Newton's second law, the net force acting on the block is equal to its mass times its acceleration.
- Since the lift is falling freely, the acceleration of the block is also g downward.
- The net force acting on the block can be expressed as:
mg−N=ma
- Here, a=g (the block is falling with the lift), so we can substitute:
mg−N=mg
- Rearranging the equation gives:
mg−N=mg⟹N=0
- This means that the normal force N acting on the block is zero.
- The limiting frictional force Ff is given by:
Ff=μN
- Since N=0:
Ff=μ×0=0
The limiting frictional force between the block and the surface when the lift is falling freely is 0.
Similar Questions
A block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal. If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View Solution
Knowledge Check
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A block of mass m rests on a rough floor . The coefficient of friction between the block and the floor is μ
a. Two boys apply force P at an angle θ to the horizontal. One of them pushes the block , the other one pulls. Which one would reqire less efferts to cause impending motion of the block?
b. What is the minimum force required to move the block by pulling it ?
c. Shown that the block is pushes at a certain angle θ0 it cannot be moved fro whatever the value of P be
View SolutionA block of mass m is in contact with the cart C as shown in The coefficient of static friction between the block and the cart is μ The acceleration a of the cart that will prevent the block from falling satisfies
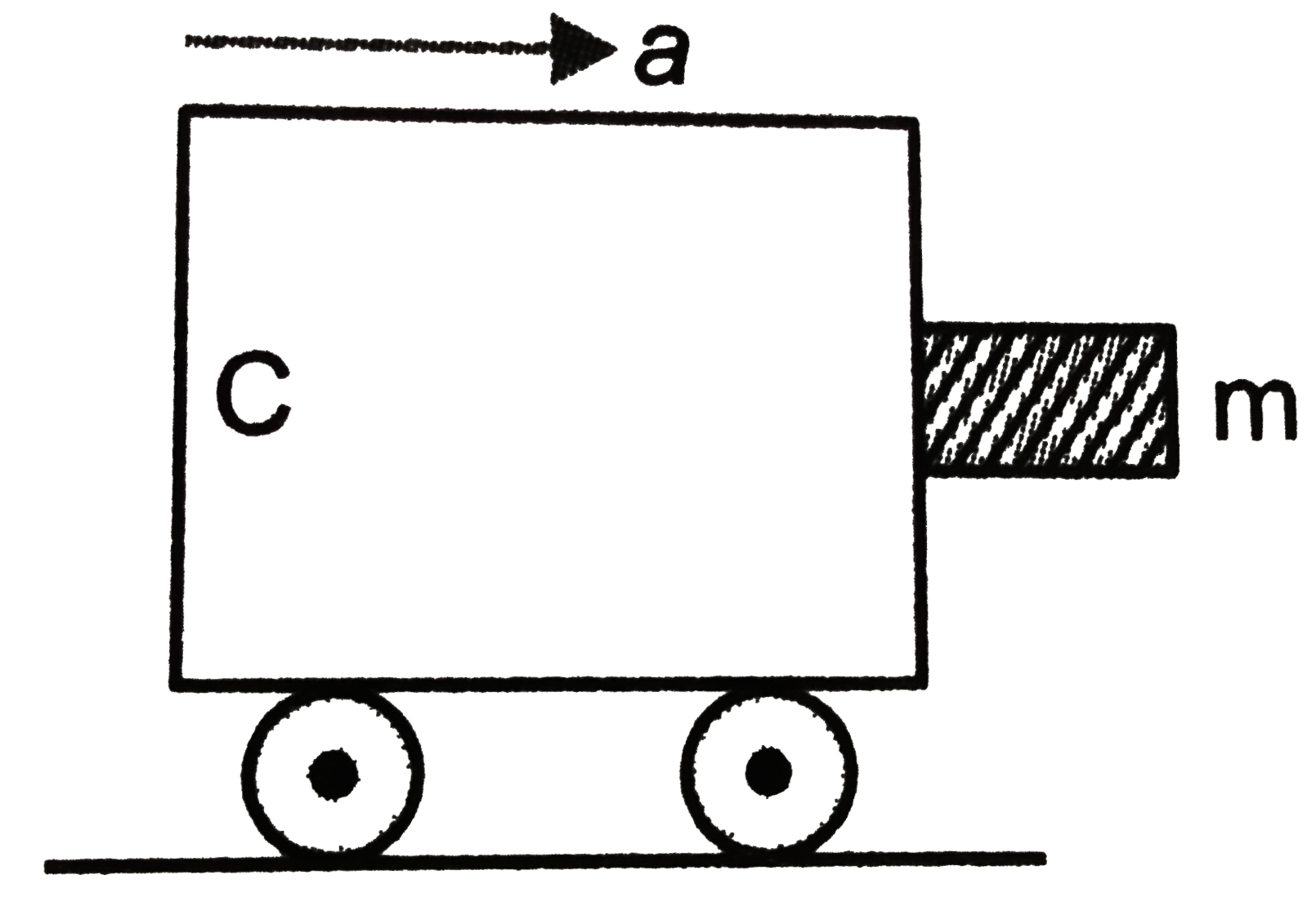 .
.
View SolutionA block of mass 0.1 kg is held against a wall applying a horizontal force of 5 N on the block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is :
View SolutionA block of mass 0.1 is held against a wall applying a horizontal force of 5N on block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is:
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
View SolutionA block of mass M is kept on as rough horizontal surface. The coefficient of static friction between the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
View Solution
