If we imagine two ideally smooth surfaces & if they are kept in contact, the frictional force acting between them is,
zero
a small finite value but not zero
very high
we can't predict
The correct Answer is:C
To solve the problem, we need to determine the frictional force acting between two ideally smooth surfaces that are in contact. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Concept of Friction
Friction is a force that opposes the relative motion of two surfaces in contact. The frictional force (f) can be calculated using the formula:
f=μ⋅N
where:
- μ is the coefficient of friction between the two surfaces,
- N is the normal force acting perpendicular to the surfaces.
Step 2: Identify the Coefficient of Friction
For ideally smooth surfaces, the coefficient of friction (μ) is considered to be zero. This is because ideally smooth surfaces do not have any roughness or irregularities that would allow for friction to occur.
Step 3: Apply the Formula
Since we have established that μ=0 for ideally smooth surfaces, we can substitute this value into the friction formula:
f=0⋅N
This simplifies to:
f=0
Step 4: Conclusion
Thus, the frictional force acting between two ideally smooth surfaces in contact is zero.
Final Answer
The frictional force acting between two ideally smooth surfaces kept in contact is zero.
---
Friction is a force that opposes the relative motion of two surfaces in contact. The frictional force (f) can be calculated using the formula:
f=μ⋅N
where:
- μ is the coefficient of friction between the two surfaces,
- N is the normal force acting perpendicular to the surfaces.
For ideally smooth surfaces, the coefficient of friction (μ) is considered to be zero. This is because ideally smooth surfaces do not have any roughness or irregularities that would allow for friction to occur.
Since we have established that μ=0 for ideally smooth surfaces, we can substitute this value into the friction formula:
f=0⋅N
This simplifies to:
f=0
Thus, the frictional force acting between two ideally smooth surfaces in contact is zero.
The frictional force acting between two ideally smooth surfaces kept in contact is zero.
Similar Questions
Knowledge Check
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
A10NB5NC2.5ND20N
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionTwo balls, each of radius R, equal mass and density are placed in contact, then the force of gravitation between them is proportional to
View SolutionTwo balls, each of radius R, equal mass and density are placed in contact, then the force of gravitation between them is proportional to
View SolutionIn a situation the contact force by a rough horizontal surface on a body placed on it has constant magnitude. If the angle between this force and the vertical is decreased, the frictional force between the surface and the body will
View SolutionTwo blocks A and B of masses 2kg and 8kg respectively are kept in contact and pressed against a roguh vertical wall by applying a horizontal force F=50N as shown, The frictional force acting between the two blocks is
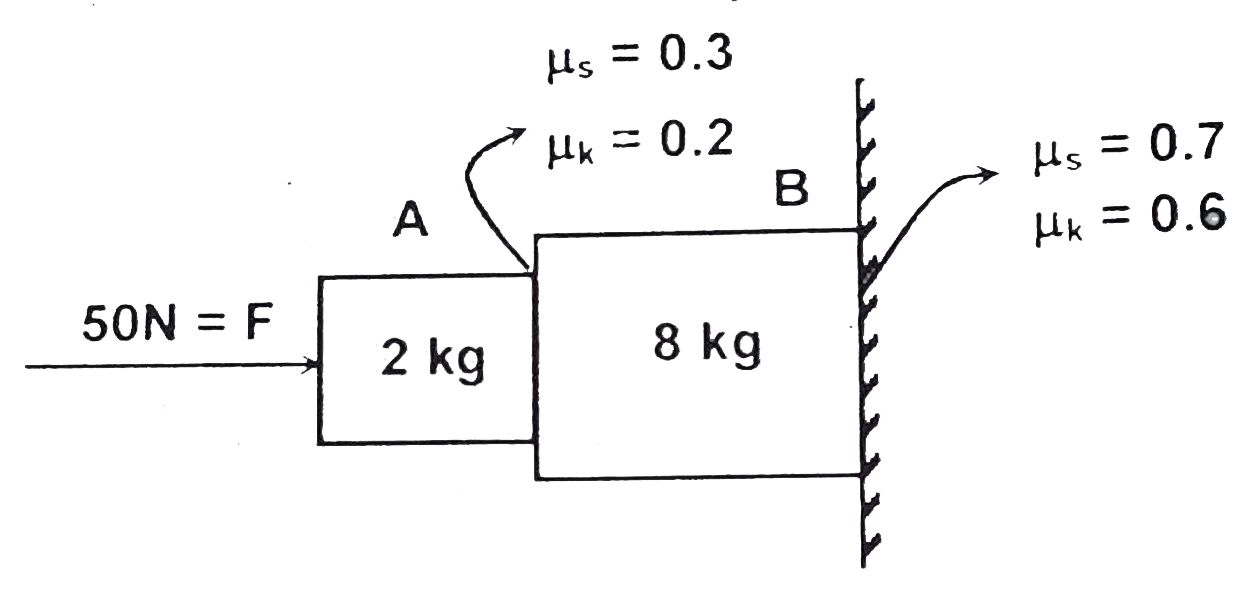
View SolutionIn Fig the block are at rest a force of 10N act on the block of 4kg mass .The coefficient of staic friction and the coefficient of kinetic friction are μs=0.2andμs=0.15 for both the surface in contact The magnitudeof frcition force acting between the surface of contact between the 2kg and 4kg block in this situation is

View SolutionStatic friction between two surfaces
View Solution
