A wooden block of 100 kg is about to be pushed on a floor of coefficient of friction 0.4. What is the magnitude of the force of friction on the wooden block when it is just pushed ?
392 N
294N
196N
490N
The correct Answer is:A
To solve the problem, we need to find the magnitude of the force of friction acting on a wooden block when it is just about to be pushed. Here are the steps to arrive at the solution:
Step 1: Identify the given values
- Mass of the wooden block (m) = 100 kg
- Coefficient of friction (μ) = 0.4
Step 2: Calculate the weight of the block
The weight (W) of the block can be calculated using the formula:
W=m×g
where g is the acceleration due to gravity (approximately 9.8m/s2).
Substituting the values:
W=100kg×9.8m/s2=980N
Step 3: Determine the normal force
In this scenario, the normal force (N) acting on the block is equal to its weight when it is on a horizontal surface. Therefore:
N=W=980N
Step 4: Calculate the force of friction
The force of friction (F_friction) can be calculated using the formula:
Ffriction=μ×N
Substituting the values:
Ffriction=0.4×980N
Ffriction=392N
Conclusion
The magnitude of the force of friction on the wooden block when it is just pushed is 392 N.
---
- Mass of the wooden block (m) = 100 kg
- Coefficient of friction (μ) = 0.4
The weight (W) of the block can be calculated using the formula:
W=m×g
where g is the acceleration due to gravity (approximately 9.8m/s2).
W=100kg×9.8m/s2=980N
In this scenario, the normal force (N) acting on the block is equal to its weight when it is on a horizontal surface. Therefore:
N=W=980N
The force of friction (F_friction) can be calculated using the formula:
Ffriction=μ×N
Ffriction=0.4×980N
Ffriction=392N
The magnitude of the force of friction on the wooden block when it is just pushed is 392 N.
Similar Questions
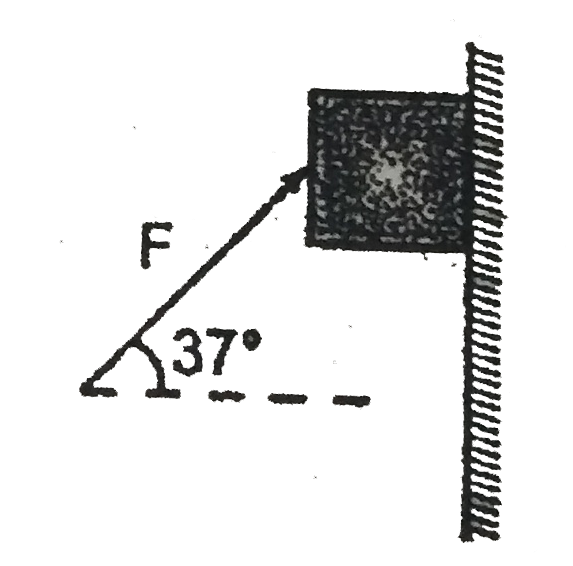
A 1 kg block is being pushed against a wall by a force F=75N as shown in the figure. The coefficient of friction is 0.25. The magnitude of acceleration of the block is:
View SolutionA block of mass 1 kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5, magnitude of the frictional force acting on the block is
View Solution
Knowledge Check
A block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4. A force F of 3 N is applied on the block as shown in figure. The force of friction between the block and the floor is (Take g=10ms−2)
 A3 NB8 NC4 ND6 N
A3 NB8 NC4 ND6 NA crate of mass 100 kg is at rest on a horizontal floor. The coefficient of static friction between the crate and the floor is 0.4, and the coefficient of kinetic friction is 0.3 . A force F of magnitude 344 N is then applied to the crate, parallel to the floor. Which of the following is true ?
AThe crate will accelerate across the floor at 0.5m/s2.BThe static friction force, which is the reaction force to F as guaranteed by Newton 's Third Law, will also have a magnitude of 344 N.CThe crate will slide across the floor at a constant speed of 0.5m/s.DThe crate will not move.
A block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-

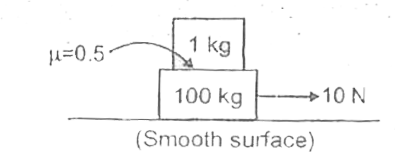
View SolutionThe frictional force acting on 1 kg block is
View SolutionA block of mass 0.1 kg is held against a wall applying a horizontal force of 5 N on the block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is :
View SolutionA block of mass 0.1 is held against a wall applying a horizontal force of 5N on block. If the coefficient of friction between the block and the wall is 0.5, the magnitude of the frictional force acting on the block is:
View SolutionA block of mass 20kg is pushed with a horizontal force of 90N. If the coefficient of static & kinetic friction are 0.4 & 0.3, the frictional force acting on the block is: (g=10ms−2)
View SolutionA block of mass 2 kg is placed on the floor . The coefficient of static friction is 0.4 . If a force of 2.8 N is applied on the block parallel to floor , the force of friction between the block and floor is : (Taking g = 10m/s2)
View Solution
