A block is pushed up a rough inclined plane of 45°. If the time of descent is twice the time of ascent, the coefficient of friction is
0.6
0.4
0.5
0.25
The correct Answer is:A
To solve the problem of a block being pushed up a rough inclined plane at an angle of 45°, where the time of descent is twice the time of ascent, we need to find the coefficient of friction. Let's break down the solution step by step.
Step 1: Understanding the Forces Acting on the Block
When the block is on the inclined plane, the forces acting on it include:
- Gravitational force (mg) acting downwards.
- Normal force (N) acting perpendicular to the surface of the incline.
- Frictional force (f) acting opposite to the direction of motion.
Step 2: Analyzing the Forces
For an inclined plane at an angle θ (which is 45° in this case):
- The component of gravitational force parallel to the incline: F∥=mgsin(θ)
- The component of gravitational force perpendicular to the incline: F_{\perpendicular} = mg \cos(θ)
Since θ = 45°, we have:
- F∥=mgsin(45°)=mg√22
- F_{\perpendicular} = mg \cos(45°) = mg \frac{\sqrt{2}}{2}
Step 3: Normal Force and Friction
The normal force (N) is equal to the perpendicular component of the weight:
N=mgcos(45°)=mg√22
The frictional force (f) can be expressed as:
f=μN=μmg√22
where μ is the coefficient of friction.
Step 4: Equations of Motion
Let ta be the time of ascent and td be the time of descent. According to the problem, td=2ta.
Using the equations of motion, we can express the acceleration during ascent and descent:
- For ascent (against gravity and friction):
aa=gsin(45°)+μgcos(45°)=g√22+μg√22
- For descent (with gravity and friction):
ad=gsin(45°)−μgcos(45°)=g√22−μg√22
Step 5: Relating Time and Acceleration
Using the relationship between time, distance, and acceleration:
- For ascent:
d=12aat2a
- For descent:
d=12adt2d=12ad(2ta)2=2adt2a
Setting the distances equal gives:
12aat2a=2adt2a
Step 6: Simplifying the Equation
Dividing both sides by t2a and simplifying:
12aa=2ad
aa=4ad
Step 7: Substituting for Accelerations
Substituting the expressions for aa and ad:
g√22+μg√22=4(g√22−μg√22)
Step 8: Solving for the Coefficient of Friction
Now, simplifying the equation:
g√22+μg√22=4g√22−4μg√22
μg√22+4μg√22=4g√22−g√22
5μg√22=3g√22
Dividing both sides by g√22:
5μ=3
μ=35
Final Answer
The coefficient of friction μ is 35.
---
When the block is on the inclined plane, the forces acting on it include:
- Gravitational force (mg) acting downwards.
- Normal force (N) acting perpendicular to the surface of the incline.
- Frictional force (f) acting opposite to the direction of motion.
For an inclined plane at an angle θ (which is 45° in this case):
- The component of gravitational force parallel to the incline: F∥=mgsin(θ)
- The component of gravitational force perpendicular to the incline: F_{\perpendicular} = mg \cos(θ)
- F∥=mgsin(45°)=mg√22
- F_{\perpendicular} = mg \cos(45°) = mg \frac{\sqrt{2}}{2}
The normal force (N) is equal to the perpendicular component of the weight:
N=mgcos(45°)=mg√22
f=μN=μmg√22
where μ is the coefficient of friction.
Let ta be the time of ascent and td be the time of descent. According to the problem, td=2ta.
- For ascent (against gravity and friction):
aa=gsin(45°)+μgcos(45°)=g√22+μg√22
- For descent (with gravity and friction):
ad=gsin(45°)−μgcos(45°)=g√22−μg√22
Using the relationship between time, distance, and acceleration:
- For ascent:
d=12aat2a
- For descent:
d=12adt2d=12ad(2ta)2=2adt2a
12aat2a=2adt2a
Dividing both sides by t2a and simplifying:
12aa=2ad
aa=4ad
Substituting the expressions for aa and ad:
g√22+μg√22=4(g√22−μg√22)
Now, simplifying the equation:
g√22+μg√22=4g√22−4μg√22
μg√22+4μg√22=4g√22−g√22
5μg√22=3g√22
Dividing both sides by g√22:
5μ=3
μ=35
The coefficient of friction μ is 35.
Similar Questions
A body of mass m is launched up on a rough inclined plane making an angle 45∘ with horizontal If the time of ascent is half of the time of descent, the frictional coefficient between plane and body is
View SolutionA body of mass 5×10−3 kg is launched upon a rough inclined plane making an angle of 30∘ with the horizontal. Obtain the coefficient of friction between the body and the plane if the time of ascent is half of the time of descent.
View Solution
Knowledge Check
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3NA block of mass m=2kg is resting on a rough inclined plane of inclination of 30∘ as shown in figure. The coefficient of friction between the block and the plane is μ=0.5. What minimum force F should be applied perpendicular to the plane on the block, so that blocks does not slip on the plane? (g=10m/s2)
 A2.68 NBZeroC4.34 ND6.24 N
A2.68 NBZeroC4.34 ND6.24 N
A block is pushed up a rough inclined plane of 45°. If the time of descent is twice the time of ascent, the coefficient of friction is
View SolutionA block is projected upwards on a rough inclined plane at 20 m/s. let the time in going up, then is equal to
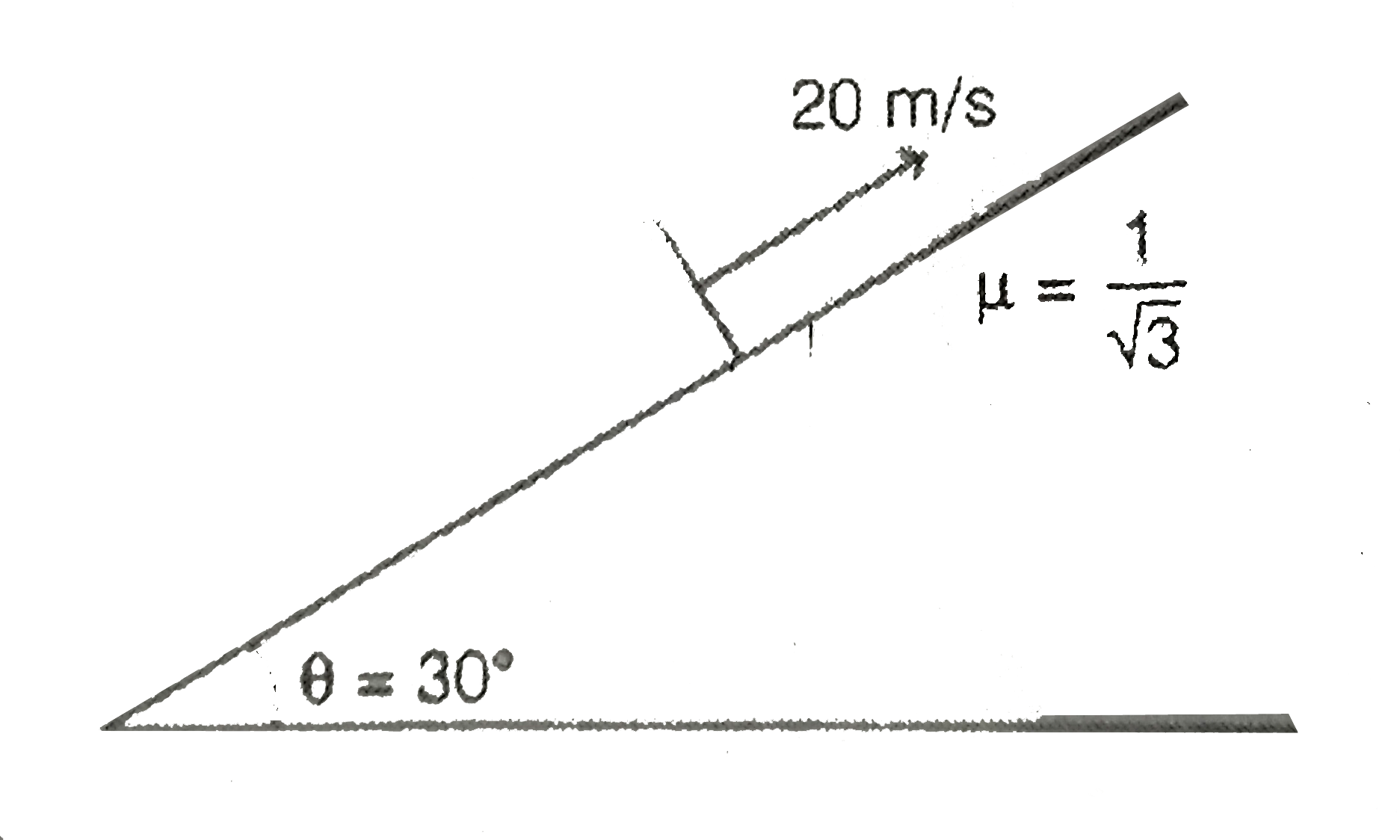
View SolutionA body is pushed up on a rough inclined plane making an angle 30∘ to the horizontal. If its time of ascent on the plane is half the time of its descent, find coefficient of friction between the body and the incined plane.
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View Solution
