The standard deviation of a poisson distribution is 4 then its mean is
4
16
2
8
The correct Answer is:B
To find the mean of a Poisson distribution given that its standard deviation is 4, we can follow these steps:
1. Understand the relationship between mean and variance in a Poisson distribution:
- In a Poisson distribution, the mean (μ) is equal to the variance (σ²). This means that if we denote the mean by λ, we have:
μ=λandσ2=λ
2. Relate standard deviation to variance:
- The standard deviation (σ) is the square root of the variance. Therefore, we can express this as:
σ=√σ2=√λ
3. Set up the equation using the given standard deviation:
- According to the problem, the standard deviation is given as 4. Thus, we can write:
√λ=4
4. Square both sides to solve for λ:
- To eliminate the square root, we square both sides of the equation:
λ=42
- This simplifies to:
λ=16
5. Conclusion:
- Since the mean (μ) of the Poisson distribution is equal to λ, we conclude that:
Mean=16
Final Answer:
The mean of the Poisson distribution is 16.
---
- In a Poisson distribution, the mean (μ) is equal to the variance (σ²). This means that if we denote the mean by λ, we have:
μ=λandσ2=λ
- The standard deviation (σ) is the square root of the variance. Therefore, we can express this as:
σ=√σ2=√λ
- According to the problem, the standard deviation is given as 4. Thus, we can write:
√λ=4
- To eliminate the square root, we square both sides of the equation:
λ=42
- This simplifies to:
λ=16
- Since the mean (μ) of the Poisson distribution is equal to λ, we conclude that:
Mean=16
The mean of the Poisson distribution is 16.
Similar Questions
Knowledge Check
If the mean and standard deviation of a binomial distribution are 12 and 2 respectively , then the value of its parameter p is
A14B13C12D23
If the standerd deviation of the binomial distribution (q+p)16 is 2, then mean is
View SolutionCalculate the standard deviation of the following distribution:

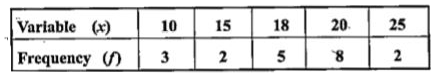
View SolutionCompute the standard deviation for the following distribution.
View SolutionIf the mean of a binomial distribution is 25, then its standard deviation lies in the interval
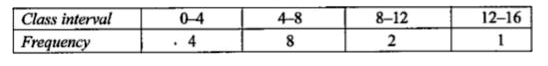
View SolutionCalculate the standard deviation for the following distribution:
View SolutionCalculate the standard deviation for the following distribution.

View SolutionWhat is the algebraic sum of deviations of a frequency distribution about its mean?
View Solution