A person has 2 parents, 4 grandparents, 8 great grandparents, and so on, then the number of his ancestors during the ten generations preceeding his own is
2046
3046
4046
5056
The correct Answer is:A
To find the total number of ancestors a person has during the ten generations preceding their own, we can follow these steps:
Step 1: Understand the pattern of ancestors
Each person has:
- 2 parents
- 4 grandparents (2^2)
- 8 great-grandparents (2^3)
- And so on...
This means that for each generation, the number of ancestors doubles. Therefore, the number of ancestors in each generation can be expressed as powers of 2.
Step 2: Write down the formula for ancestors
For the nth generation, the number of ancestors is given by 2n. Thus, for 10 generations preceding the person, we have:
- Generation 1 (parents): 21
- Generation 2 (grandparents): 22
- Generation 3 (great-grandparents): 23
- ...
- Generation 10: 210
Step 3: Calculate the total number of ancestors
To find the total number of ancestors from generation 1 to generation 10, we need to sum the series:
Total Ancestors=21+22+23+...+210
This series can be recognized as a geometric series where:
- First term a=21
- Common ratio r=2
- Number of terms n=10
Step 4: Use the formula for the sum of a geometric series
The sum Sn of the first n terms of a geometric series can be calculated using the formula:
Sn=arn−1r−1
Substituting the values:
- a=21=2
- r=2
- n=10
We get:
S10=2210−12−1=2(210−1)
Step 5: Calculate 210
Calculating 210:
210=1024
Step 6: Substitute back into the sum formula
Now substituting 210 back into the sum:
S10=2(1024−1)=2×1023=2046
Step 7: Include the person themselves
Since the question asks for the total number of ancestors during the ten generations preceding the person, we need to add the person themselves to the total:
Total Ancestors including the person=2046+1=2047
Final Answer
Therefore, the total number of ancestors of that person during the ten generations preceding their own is 2047.
---
Each person has:
- 2 parents
- 4 grandparents (2^2)
- 8 great-grandparents (2^3)
- And so on...
For the nth generation, the number of ancestors is given by 2n. Thus, for 10 generations preceding the person, we have:
- Generation 1 (parents): 21
- Generation 2 (grandparents): 22
- Generation 3 (great-grandparents): 23
- ...
- Generation 10: 210
To find the total number of ancestors from generation 1 to generation 10, we need to sum the series:
Total Ancestors=21+22+23+...+210
- First term a=21
- Common ratio r=2
- Number of terms n=10
The sum Sn of the first n terms of a geometric series can be calculated using the formula:
Sn=arn−1r−1
Substituting the values:
- a=21=2
- r=2
- n=10
S10=2210−12−1=2(210−1)
Calculating 210:
210=1024
Now substituting 210 back into the sum:
S10=2(1024−1)=2×1023=2046
Since the question asks for the total number of ancestors during the ten generations preceding the person, we need to add the person themselves to the total:
Total Ancestors including the person=2046+1=2047
Therefore, the total number of ancestors of that person during the ten generations preceding their own is 2047.
Similar Questions
A person has 2 parents, 4 grandparents, 8 great grand parents, and so on. Find the number of his ancestors during the ten generations preceding his one.
View SolutionA person has 2 parents, 4 grandparents, 8 great grand parents, and so on. Find the number of his ancestors during the ten generations preceding his one.
View SolutionAssertion : If the muscles of both auricles of the person permanently fail to contract due to any reason there will still be no change in his general condition at rest.
Reason :75% of the amount of blood transferred from auricles to ventricles is during auricular diastole.
View SolutionA man X has 7 friends, 4 of them are ladies and 3 are men. His wife Y also has 7 friends, 3 of them are ladies and 4 are men. Assume X and Y have no common friends. Then the total number of ways in which X and Y together can throw a party inviting 3 ladies and 3 men, so that 3 friends of each of X and Y are in the party, is : 469 (2) 484 (3) 485 (4) 468
View SolutionPankaj distributes 4 sweets to each student in his class and has 7 sweets left over. Find a rule for the number of sweets he had in terms of the number of students in his class.
View SolutionA person walks along a straight road from his house to a market 2.5kms away with a speed of 5 km/hr and instantly turns back and reaches his house with a speed of 7.5 kms/hr. The average speed of the person during the time interval 0 to 50 minutes is (in m/sec)
View Solution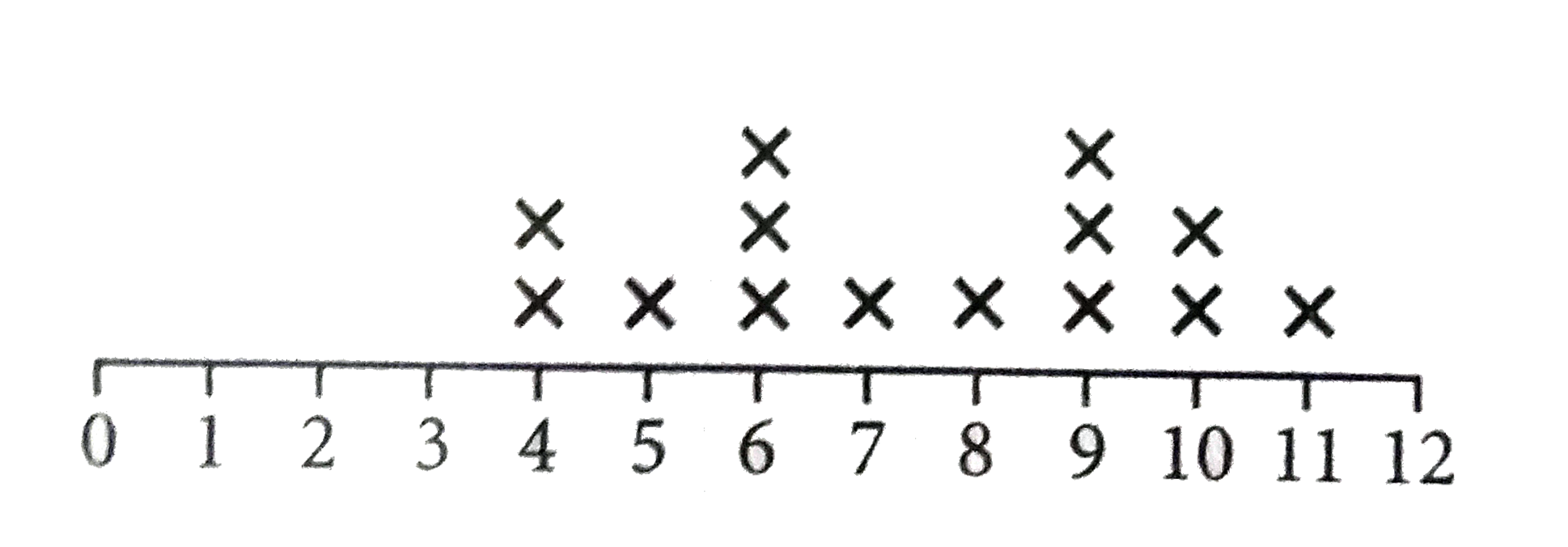
Brian and several of his work colleagues are on a kickball team together. The dot plot above shows the number of points his team scored during each of 14 games played so far this season. The league's record for highest average points scored per game during a season is 7.5. To break this record, a team's season average must be at least half a point higher. If Brian and his teammates are to set a new season record, how many points must they average during their final two games?
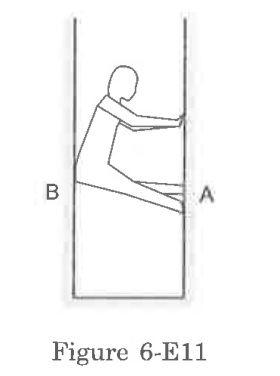
View SolutionA person (40 kg) is managing to be at rest between two verticle walls by pressing one wall A by hi hands and feet and the other wall B by his back figure. Assume that the friction coefficient between his body and the walls is 0.8 and that limiting friction acts at all the contacts. a. show that the person pushes the two walls with equal force. b. find the normal force exerted by either wall on the person Take g=10ms2.
View SolutionIn each of the following experiments specify appropriate sample space(i) A boy has a 1 rupee coin, a 2 rupee coin and a 5 rupee coin in his pocket. He takes out two coins out of his pocket, one after the other.(ii) A person is noting down the number of accidents along a busy highway during a year.
View Solution105 goats, 140 donkeys and 175 cows have to be taken across a river. There is only one boat which will have to make many trips in order to do so. The lazy boatman has his own conditions for transporting them. He insists that he will take the same number of animals in every trip and they have to be of the same kind. He will naturally like to take the largest possible number each time. Can you tell how many animals went in each trip?
View Solution