A stair case contains ten steps each 10cm high and 20 cm wide. The minimum horizontal velocity with which the ball has to be rolled off the upper most step, so as to hit directly the edge of the lowest steps is (approximately)
42ms−1
4.2ms−1
24ms−1
2.4ms−1
The correct Answer is:B
Step by step video & image solution for A stair case contains ten steps each 10cm high and 20 cm wide. The minimum horizontal velocity with which the ball has to be rolled off the upper most step, so as to hit directly the edge of the lowest steps is (approximately) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION128videos
APPENDICES (REVISION EXERCISE)
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - MOTION IN A STRAIGHT LINE59videos
APPENDICES (REVISION EXERCISE)
- BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )52videos
APPENDICES ( REVISION EXERCISE )
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE21videos
ATOMS
Similar Questions
A staircase contains three steps each 10 cm high and 20 cm wide figure. What should be the minimum horizontal velocity of a bal rolling off the upper most plane so as to hit directly the lowest plane?
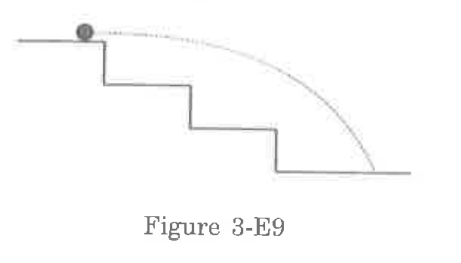 View Solution
View SolutionA staircase contains three steps each 10 cm high and 20 cm wide figure. What should be the minimum horizontal velocity of a bal rolling off the upper most plane so as to hit directly the lowest plane?
 View Solution
View SolutionA staircase contains three steps each 10 cm high and 20 cm wide figure. What should be the minimum horizontal velocity of a bal rolling off the upper most plane so as to hit directly the lowest plane?
 View Solution
View SolutionA ball rolls off the top of a staircase with a horizontal velocity um/s. If the steps are h meter high and b meter wide, the ball will hit the edge of the nth steps, if:
View SolutionA ball rolls off the top of a staircase with a horizontal velocity um/s. If the steps are h meter high and b meter wide, the ball will hit the edge of the nth steps, if:
View SolutionA ball rolls off the top of a staircase with a horizontal velocity um/s. If the steps are h meter high and b meter wide, the ball will hit the edge of the nth steps, if:
View SolutionA small rolls of the top of a stairway horizontally with a velocity of 4.5ms−1. Each step is 0.2 m high and 0.3 m wide. If g is 10ms−2, then the ball will strike the nth step where n is equal to (assume ball strike at the edge of the step).
View SolutionA tennis ball rolls off the top of a stair case way with a horizontal velocity ms−1 If the steps are b metre wide and h metre high, the ball will hit the edge of the nth step, if
View SolutionA popular game in Indian villages is goli which is played with small glass balls called golis. The goli of one player is situatted at a distance of 2.0 m from the goli of the second player. This second player has to project his goli by keeping the thumb of the left hand at the place of his goli, holding the goli between his two middlefilngers and making the throw. If he projected is 19.6 cm from the ground and the goli is to be projected horizontally, with what speed shold it be projected so that it directly hits the stationary goli without falling on the ground earlier?
View SolutionA ball rolls off the top of a stariway with a horizontal velocity magnitude 150 cm/sec. The steps are 20 cm high and 20 cm wide . Which step will the ball hit first ?
View Solution
