If the line 3x-2y + 6=0 meets X-axis and Y-axis respectively at A and B, then find the equation of the circle with radius AB and centre at A.
x2+y2+4x+9=0
x2+y2+4x−9=0
x2+y2−4+4=0
x2+y2+4x−4=0
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Find the coordinates of points A and B
The line given is 3x−2y+6=0. To find where this line intersects the x-axis (point A), we set y=0:
3x+6=0⟹3x=−6⟹x=−2
So, the coordinates of point A are (−2,0).
Next, to find where this line intersects the y-axis (point B), we set x=0:
−2y+6=0⟹−2y=−6⟹y=3
Thus, the coordinates of point B are (0,3).
Step 2: Calculate the distance AB
The distance AB can be calculated using the distance formula:
AB=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of A and B:
AB=√(0−(−2))2+(3−0)2=√(0+2)2+32=√22+32=√4+9=√13
Step 3: Write the equation of the circle
The center of the circle is at point A (−2,0) and the radius R is AB=√13.
The standard equation of a circle with center (h,k) and radius R is given by:
(x−h)2+(y−k)2=R2
Substituting h=−2, k=0, and R=√13:
(x−(−2))2+(y−0)2=(√13)2
This simplifies to:
(x+2)2+y2=13
Step 4: Rearranging the equation
To express this in a standard form, we can expand it:
(x+2)2+y2=13
Expanding (x+2)2:
x2+4x+4+y2=13
Now, rearranging gives us:
x2+4x+y2+4−13=0⟹x2+4x+y2−9=0
Final Answer
The equation of the circle is:
x2+4x+y2−9=0
---
The line given is 3x−2y+6=0. To find where this line intersects the x-axis (point A), we set y=0:
The distance AB can be calculated using the distance formula:
The center of the circle is at point A (−2,0) and the radius R is AB=√13.
To express this in a standard form, we can expand it:
The equation of the circle is:
Similar Questions
Find the equation of the circle with centre
(3,4) and touching y− axis.
View SolutionThe line 2x−y+6=0 meets the circle x2+y2−2y−9=0 at A and B. Find the equation of the circle on AB as diameter.
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
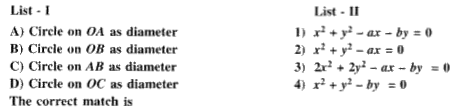
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
equation of perpendicular bisector of line segment AB
View SolutionA line AB meets X-axis at A and Y-axis at B. P(4, -1) divides AB in the ratio 1:2.
(ii) Find the equation of the line through P and perpendicular to AB
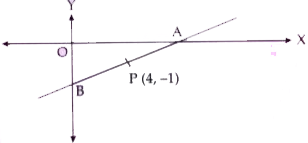
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
the co-ordinates of A and B.
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
equation of line AB.
View SolutionA line 5x+3y+15=0 meets y-axis at point P. Find the co-ordinates of point P. Find the equation of a line through P and perpendicular to x−3y+4=0.
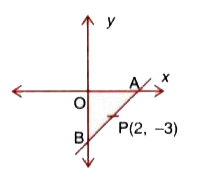
View SolutionA line AB meets X-axis at A and Y-axis at B. P(4, -1) divides AB in the ratio 1:2.
(i) Find the coordinates of A and B.
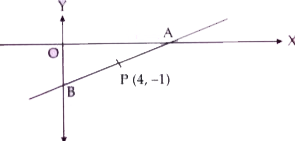
View SolutionA line AB meets X-axis at A and Y-axis at B. P(4,−1) divides AB in the ratio 1:2.
Find the Coordinates of A and B
View Solution
