The shape of orbital for which l = 1 is
Spherical
Dumb-bell
Double dumb-bell
Circular
The correct Answer is:B
To determine the shape of the orbital for which the azimuthal quantum number l=1, we can follow these steps:
Step 1: Understand the Quantum Number l
The azimuthal quantum number l is associated with the shape of the orbital. It can take on integer values starting from 0 up to n−1, where n is the principal quantum number.
Step 2: Identify the Corresponding Orbital Type
For different values of l:
- l=0 corresponds to the s orbital (spherical shape).
- l=1 corresponds to the p orbital (dumbbell shape).
- l=2 corresponds to the d orbital (double dumbbell shape).
- l=3 corresponds to the f orbital (complex shapes).
Step 3: Determine the Shape of the Orbital for l=1
Since we are looking for the shape of the orbital when l=1, we conclude that it corresponds to the p orbital. The p orbital is characterized by its dumbbell shape.
Conclusion
The shape of the orbital for which l=1 is a dumbbell shape.
---
The azimuthal quantum number l is associated with the shape of the orbital. It can take on integer values starting from 0 up to n−1, where n is the principal quantum number.
For different values of l:
- l=0 corresponds to the s orbital (spherical shape).
- l=1 corresponds to the p orbital (dumbbell shape).
- l=2 corresponds to the d orbital (double dumbbell shape).
- l=3 corresponds to the f orbital (complex shapes).
Since we are looking for the shape of the orbital when l=1, we conclude that it corresponds to the p orbital. The p orbital is characterized by its dumbbell shape.
The shape of the orbital for which l=1 is a dumbbell shape.
Similar Questions
The shape of p orbital is
View SolutionThe shape of s-orbital is ………while the shape of p-orbital is...........
View SolutionThe shape of l3 is
View SolutionAn orbital with l=0 is
View SolutionHow many electrons can fit in the orbital for which n = 3 and l = 1 ?
View SolutionThe shape of the orbital with the value of l = 2 and m = 0 is
View SolutionThe shape of orbitals are related to the ratio of principal quantum number (n) to substiary quantum number (k,a modifacation of Bohr-sommerfield theory ).The value of k for any shell has a value ranging betwe3en n to l .The amximum value for k is given for x sub-shell white k becomes with p, d,f........ repectively upto minimum value
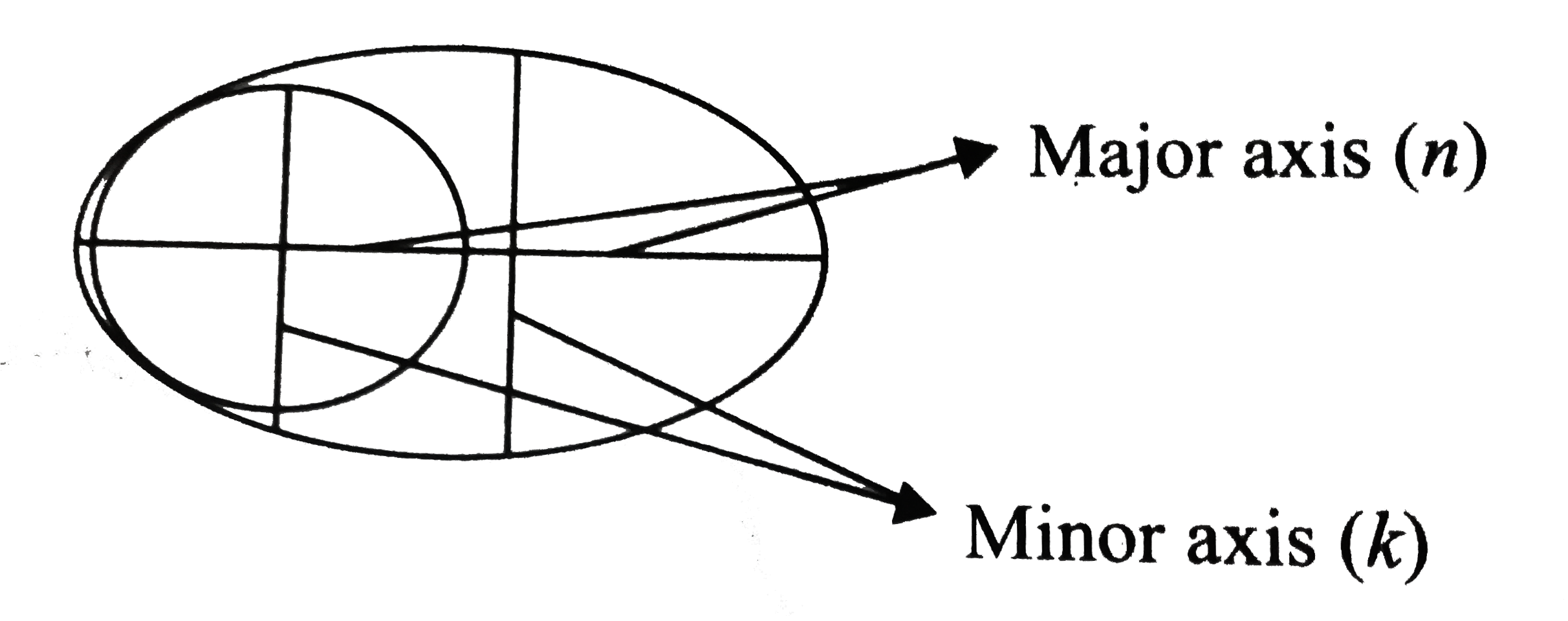
If n is the major axis and k is th e minor axis , then n/k=1 for circular shape white n/k>1 for elliptical shape
Which orbit shape has highest n/k>>1 value?
View SolutionThe shape of orbitals are related to the ratio of principal quantum number (n) to substiary quantum number (k,a modifacation of Bohr-sommerfield theory ).The value of k for any shell has a value ranging betwe3en n to l .The amximum value for k is given for x sub-shell white k becomes with p, d,f........ repectively upto minimum value
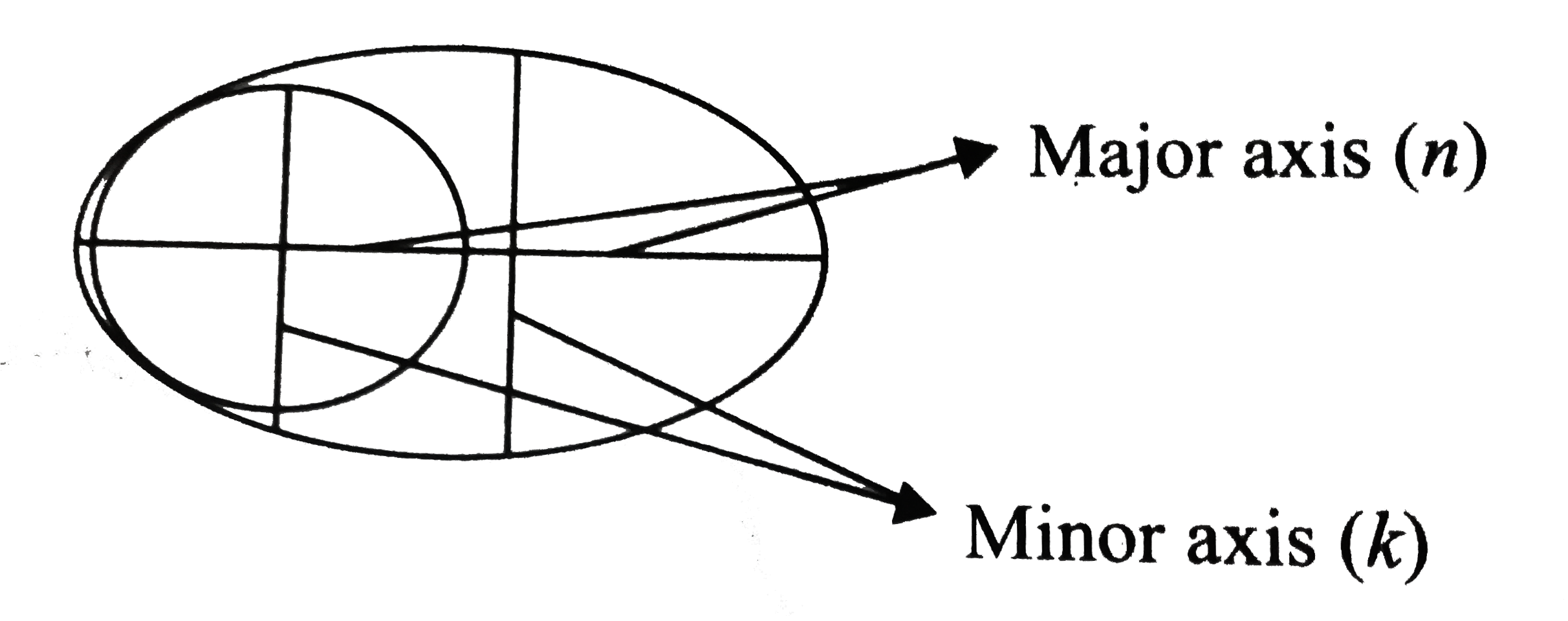
If n is the major axis and k is th e minor axis , then n/k=1 for circular shape white n/k>1 for elliptical shape
Which shape is used to be circular having n/k value
View SolutionThe shape of orbitals are related to the ratio of principal quantum number (n) to substiary quantum number (k,a modifacation of Bohr-sommerfield theory ).The value of k for any shell has a value ranging betwe3en n to l .The amximum value for k is given for x sub-shell white k becomes with p, d,f........ repectively upto minimum value
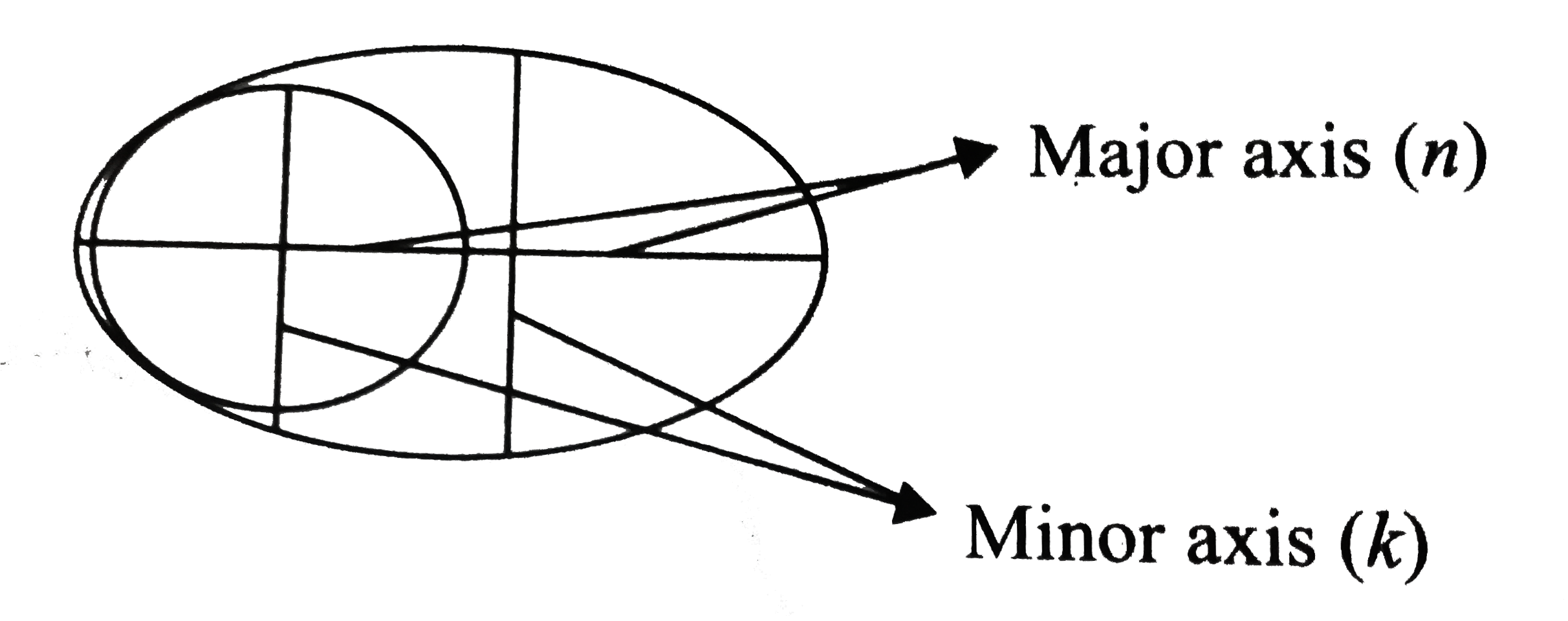
If n is the major axis and k is th e minor axis , then n/k=1 for circular shape white n/k>1 for elliptical shape
Which value of n and k suggest about the shape of 3s orbitsl?
View SolutionThe shape of orbital is determined by ………quantum number
View Solution
