The wave length of two photons A and B are 100 Å and 100 nm. The ratio of their energies will be
1:1
2:1
10:1
1:10
The correct Answer is:C
To find the ratio of the energies of two photons A and B with wavelengths of 100 Å and 100 nm, we can follow these steps:
Step 1: Understand the relationship between energy and wavelength
The energy (E) of a photon is related to its wavelength (λ) by the formula:
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34J s),
- c is the speed of light (3.00×108m/s),
- λ is the wavelength of the photon.
Step 2: Convert wavelengths to meters
We need to convert the given wavelengths from Ångströms and nanometers to meters:
- Wavelength of photon A (λ₁) = 100 Å = 100×10−10m=1×10−8m
- Wavelength of photon B (λ₂) = 100 nm = 100×10−9m=1×10−7m
Step 3: Write the energy formulas for both photons
Using the energy formula, we can express the energies of photons A and B:
- Energy of photon A (E₁):
E1=hcλ1=hc1×10−8
- Energy of photon B (E₂):
E2=hcλ2=hc1×10−7
Step 4: Find the ratio of their energies
Now, we can find the ratio of the energies:
E1E2=hcλ1hcλ2=λ2λ1
Substituting the values of λ₁ and λ₂:
E1E2=1×10−71×10−8=10
Step 5: Conclusion
The ratio of the energies of the two photons A and B is:
E1E2=10
Final Answer
The ratio of their energies E1:E2=10:1.
---
The energy (E) of a photon is related to its wavelength (λ) by the formula:
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34J s),
- c is the speed of light (3.00×108m/s),
- λ is the wavelength of the photon.
We need to convert the given wavelengths from Ångströms and nanometers to meters:
- Wavelength of photon A (λ₁) = 100 Å = 100×10−10m=1×10−8m
- Wavelength of photon B (λ₂) = 100 nm = 100×10−9m=1×10−7m
Using the energy formula, we can express the energies of photons A and B:
- Energy of photon A (E₁):
E1=hcλ1=hc1×10−8
- Energy of photon B (E₂):
E2=hcλ2=hc1×10−7
Now, we can find the ratio of the energies:
E1E2=hcλ1hcλ2=λ2λ1
Substituting the values of λ₁ and λ₂:
E1E2=1×10−71×10−8=10
The ratio of the energies of the two photons A and B is:
E1E2=10
The ratio of their energies E1:E2=10:1.
Similar Questions
Two bulbs A and B of equal capacity are filled with He and SO2, respectively, at the same temperature.
(a) If the pressures in the two bulbs are same, what will be the ratio of the velocities of the molecules of the two gases?View SolutionThe ratio of wavelength of a photon and that of an electron of same energy E will be
View Solution
Knowledge Check
- Question 1 - Select One
A proton has kinetic energy E = 100 keV which is equal to that of a photon. The wavelength of photon is λ2 and that of proton is λ1. The ratio of λ2/λ1 is proportional to
AE2BE1/2CE−1DE−1/2 - Question 2 - Select One
There are two sources of light, each emitting with a power of 100W. One emits X-rays of wavelength 1nm and the other visible light at 500nm. Find the ratio of number of photons of X-rays to the photons of visible light of the given wavelength?
A1:500B1:400C1:300D1:200
The energy of a 700- nm photon is :-
View SolutionThe amplitude of two waves are in ratio 5 : 2. If all other conditions for the two waves are same, then what is the ratio of their energy densities
View SolutionThere are two sources of light, each emitting with a power of 100W. One emits X-rays of wavelength 1nm and the other visible light at 500nm. Find the ratio of number of photons of X-rays to the photons of visible light of the given wavelength?
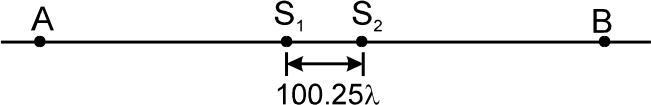
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
 View Solution
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
 View Solution
View SolutionThe two paricles A and B have de Broglie wavelengths 1 nm and 5 nm respectively if mass of A is four times the mass of B, the ratio of kinetic energies of A and B would be :-
View Solution

