Sand is pilled up on a horizontal ground in the form of a regular cone of a fixed base radius R. The coefficient of static friction between sand layers is μ. The maximum volume of sand that can be pilled up, without the sand slipping on the surface is
μR33π
μR33
μR33μ
μπR33
The correct Answer is:D
To solve the problem of finding the maximum volume of sand that can be piled up in the form of a regular cone without slipping, we can follow these steps:
Step 1: Understand the Geometry of the Cone
We have a cone with a fixed base radius R and height h. The cone is formed by piling up sand, and we need to ensure that the sand does not slip over the slant surface of the cone.
Hint: Visualize the cone and identify the parameters: base radius R and height h.
Step 2: Analyze the Forces Acting on the Sand Layers
For the sand layers to remain stable and not slip, the angle of the slant surface of the cone must be such that the static friction can hold the layers in place. This means we need to relate the angle of the cone to the coefficient of static friction μ.
Hint: Recall that the condition for no slipping is given by the relationship between the angle of the cone and the coefficient of static friction.
Step 3: Relate the Angle of the Cone to the Coefficient of Friction
Let θ be the angle of the slant surface of the cone. The tangent of this angle can be expressed as:
tan(θ)=hR
For the sand not to slip, we need:
tan(θ)≤μ
Thus, we have:
hR≤μ
Hint: This inequality tells us how high the cone can be relative to its radius.
Step 4: Solve for the Maximum Height h
From the inequality hR≤μ, we can rearrange it to find the maximum height:
h≤μR
Hint: This gives us the maximum height at which the sand can be piled without slipping.
Step 5: Calculate the Maximum Volume of the Cone
The volume V of a cone is given by the formula:
V=13πR2h
Substituting the maximum height h=μR into the volume formula, we get:
Vmax=13πR2(μR)=13πμR3
Hint: This is the final expression for the maximum volume of sand that can be piled up.
Final Answer
The maximum volume of sand that can be piled up without slipping is:
Vmax=13πμR3
We have a cone with a fixed base radius R and height h. The cone is formed by piling up sand, and we need to ensure that the sand does not slip over the slant surface of the cone.
For the sand layers to remain stable and not slip, the angle of the slant surface of the cone must be such that the static friction can hold the layers in place. This means we need to relate the angle of the cone to the coefficient of static friction μ.
Let θ be the angle of the slant surface of the cone. The tangent of this angle can be expressed as:
tan(θ)=hR
For the sand not to slip, we need:
tan(θ)≤μ
Thus, we have:
hR≤μ
From the inequality hR≤μ, we can rearrange it to find the maximum height:
h≤μR
The volume V of a cone is given by the formula:
V=13πR2h
Substituting the maximum height h=μR into the volume formula, we get:
Vmax=13πR2(μR)=13πμR3
The maximum volume of sand that can be piled up without slipping is:
Vmax=13πμR3
Similar Questions
A car is taking turn on a circular path of radius R. If the coefficient of friction between the tyres and road is μ, the maximum velocity for no slipping is
View SolutionA car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tyre and the road is μs=2.0, find the maximum speed the car can take without skidding.
View SolutionA solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to

View SolutionA horizontal force F acts on the sphere at its centre as shown. Coefficient of friction between ground and sphere is μ. What is maximum value of F for which there is no slipping?
View SolutionA horizontal force F acts on the sphere at its centre as shown. Coefficient of friction between ground and sphere is μ. What is maximum value of F for which there is no slipping?
View SolutionA block is kept on a horizontal table. The table is undergoing simple farmonic motion of frequency 3Hz in a horizontal plane. The coefficient of static friction between the block and the table surface is 0.72. find the maximum amplitude of the table at which the block does not slip on the surface g=10ms−1
View SolutionTwo hemispheres of radii R and r(<R) are fixed on a horizontal table touching each other (see figure). A uniform rod rests on to spheres as shown. The coefficient of friction between the rod and two spherers is μ. Then the maximum value of the ratio rR for which the rod will not slide is :
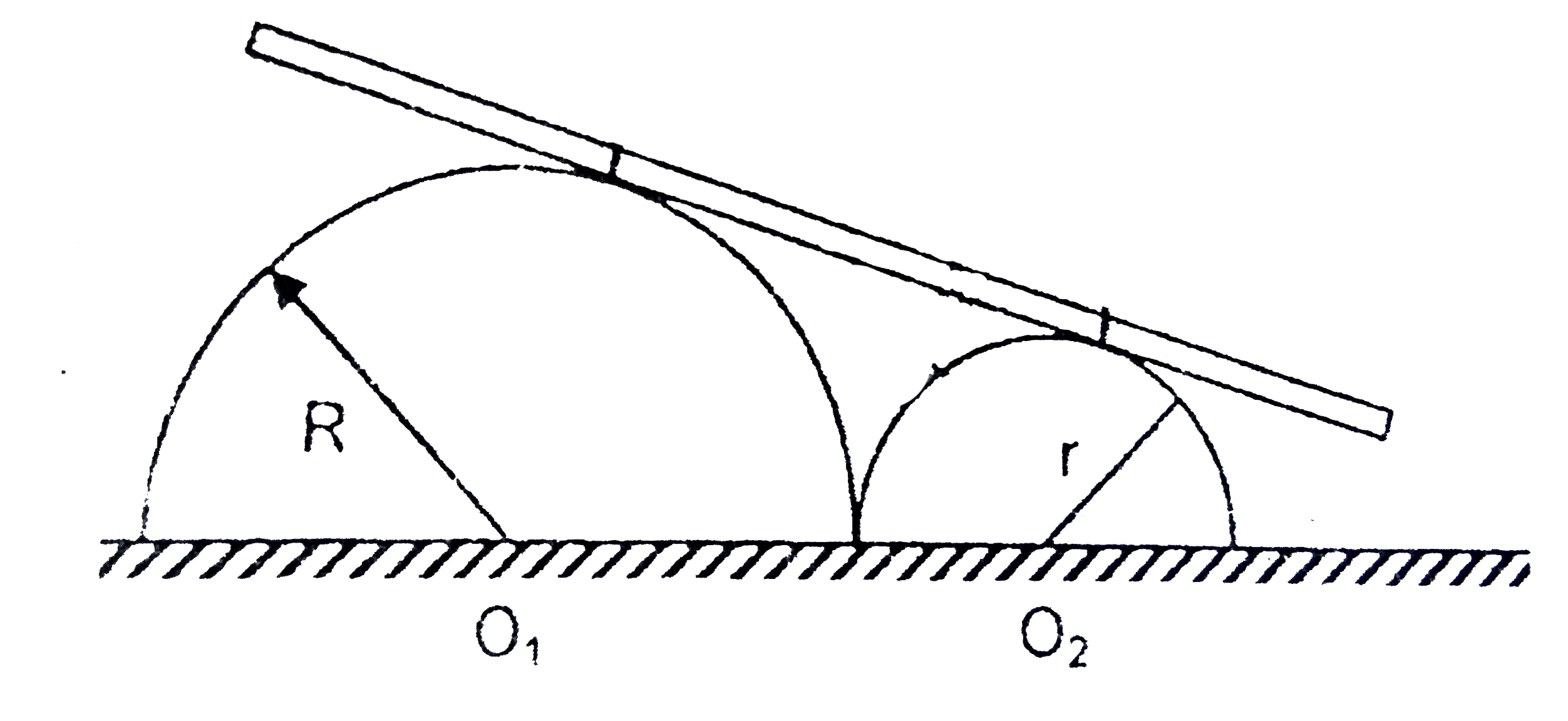
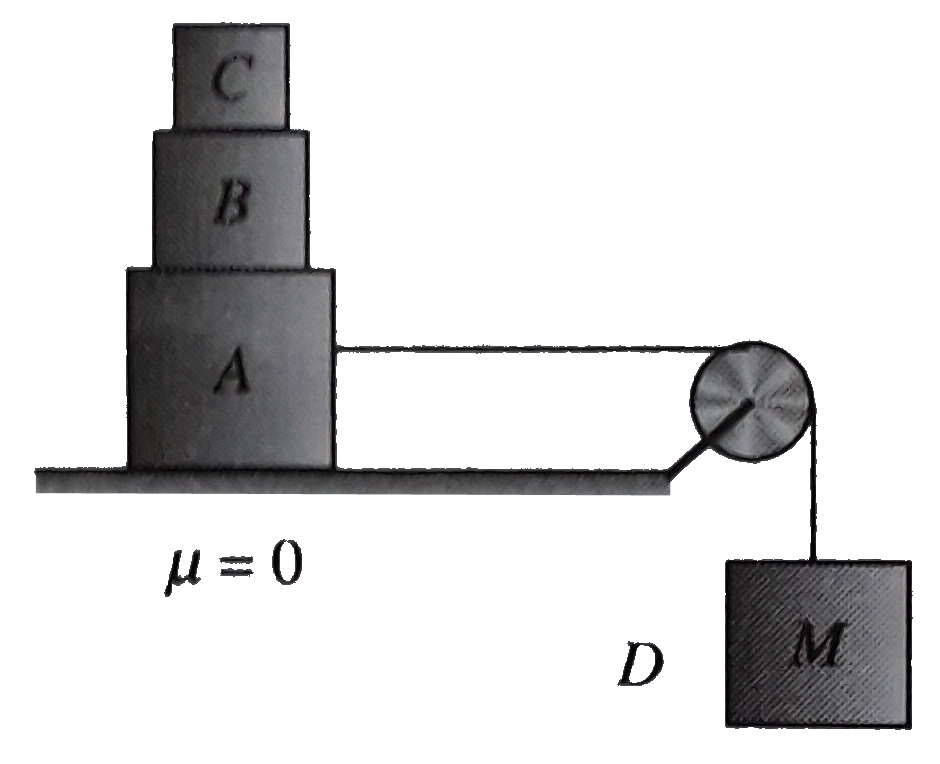
View SolutionThere block A B ,and C of equal mass m are placed one over the other on a frictionless surface (table) as shown in figure The coefficient of friction between any blocks A ,B and C is μ The maximum value of mass of block D so that the blocks A,B and C move without slipping over each other is
View SolutionA solid sphere of mass 0.50 kg is kept on a horizontal surface. The coefficient of static friction between the surfaces in contact is 2/7. What maximum force can be applied at the highest point in the horizontal direction so that the sphere does not slip on the surface?
View SolutionA solid sphere of mass 3kg is kept on a horizontal surface. The coefficient of static friction between the surfaces in contact is 2/7. What maximum force can be applied at the highest point in the horizontal direction so that the sphere does not slip on the surface? (in N)
View Solution