A block of mass 2 kg slides down an incline plane of inclination 30°. The coefficient of friction between block and plane is 0.5. The contact force between block and plank is :
20 N
10√3N
5√7N
5√15N
The correct Answer is:D
To solve the problem of finding the contact force between a block of mass 2 kg sliding down an incline of 30° with a coefficient of friction of 0.5, we can follow these steps:
Step 1: Identify the Forces Acting on the Block
The forces acting on the block include:
- The gravitational force (weight) W=mg acting vertically downward.
- The normal force N acting perpendicular to the incline.
- The frictional force f acting parallel to the incline, opposing the motion.
Step 2: Calculate the Weight of the Block
Given:
- Mass m=2 kg
- Acceleration due to gravity g=10 m/s² (approximate value)
The weight W of the block is calculated as:
W=mg=2kg×10m/s2=20N
Step 3: Resolve the Weight into Components
The weight can be resolved into two components:
- Perpendicular to the incline: W⊥=mgcos(θ)
- Parallel to the incline: W∥=mgsin(θ)
For θ=30°:
W⊥=20cos(30°)=20×√32=10√3N
W∥=20sin(30°)=20×12=10N
Step 4: Calculate the Normal Force
The normal force N is equal to the perpendicular component of the weight:
N=W⊥=10√3N
Step 5: Calculate the Frictional Force
The frictional force f can be calculated using the coefficient of friction μ:
f=μN=0.5×N=0.5×10√3=5√3N
Step 6: Calculate the Resultant Contact Force
The contact force Fc is the resultant of the normal force and the frictional force. Since these forces are perpendicular to each other, we can use the Pythagorean theorem:
Fc=√N2+f2
Substituting the values:
Fc=√(10√3)2+(5√3)2
=√100×3+25×3=√375=5√15N
Final Answer
The contact force between the block and the incline is:
Fc=5√15N
---
The forces acting on the block include:
- The gravitational force (weight) W=mg acting vertically downward.
- The normal force N acting perpendicular to the incline.
- The frictional force f acting parallel to the incline, opposing the motion.
Given:
- Mass m=2 kg
- Acceleration due to gravity g=10 m/s² (approximate value)
W=mg=2kg×10m/s2=20N
The weight can be resolved into two components:
- Perpendicular to the incline: W⊥=mgcos(θ)
- Parallel to the incline: W∥=mgsin(θ)
W⊥=20cos(30°)=20×√32=10√3N
W∥=20sin(30°)=20×12=10N
The normal force N is equal to the perpendicular component of the weight:
N=W⊥=10√3N
The frictional force f can be calculated using the coefficient of friction μ:
f=μN=0.5×N=0.5×10√3=5√3N
The contact force Fc is the resultant of the normal force and the frictional force. Since these forces are perpendicular to each other, we can use the Pythagorean theorem:
Fc=√N2+f2
Substituting the values:
Fc=√(10√3)2+(5√3)2
=√100×3+25×3=√375=5√15N
The contact force between the block and the incline is:
Fc=5√15N
Similar Questions
A block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
View SolutionA block of mass m=2kg is resting on a inclined plane of inclination 30∘ The coefficient of friction between the block and the plane is μ=0.5 what minimum force F (in newton) should be applied perpendicular to the plane on the block so that the does not slip on the plane?

View Solution
Knowledge Check
A block of mass m=2kg is resting on a rough inclined plane of inclination of 30∘ as shown in figure. The coefficient of friction between the block and the plane is μ=0.5. What minimum force F should be applied perpendicular to the plane on the block, so that blocks does not slip on the plane? (g=10m/s2)
 A2.68 NBZeroC4.34 ND6.24 N
A2.68 NBZeroC4.34 ND6.24 N
A block of mass m is placed over rough inclined plane having inclination 30∘ .The co-efficient of friction between the block and inclined plane is 0.75.The contact force on the block is x4mg. Find the value of x
View SolutionA block of mass m=2kg is resting on a inclined plane of inclination 30∘ as shown in figure The coefficient of friction between the block and the plane is μ=0.5 what minimum force F (in newton) should be applied perpendicular to the plane on the block so that the does not slip on the plane?

View SolutionA block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View SolutionA block of mass m sides down an inclined right angled trough .If the coefficient of friction between block and the trough is μk acceleration of the block down the plane is
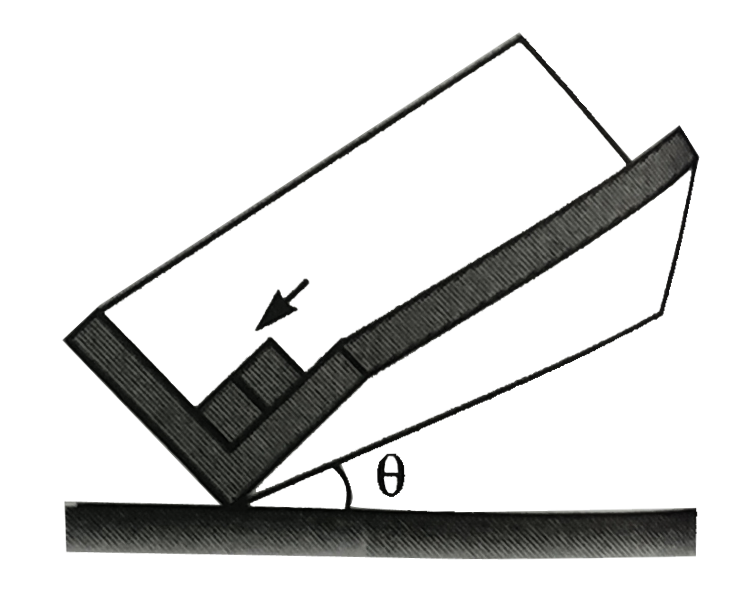
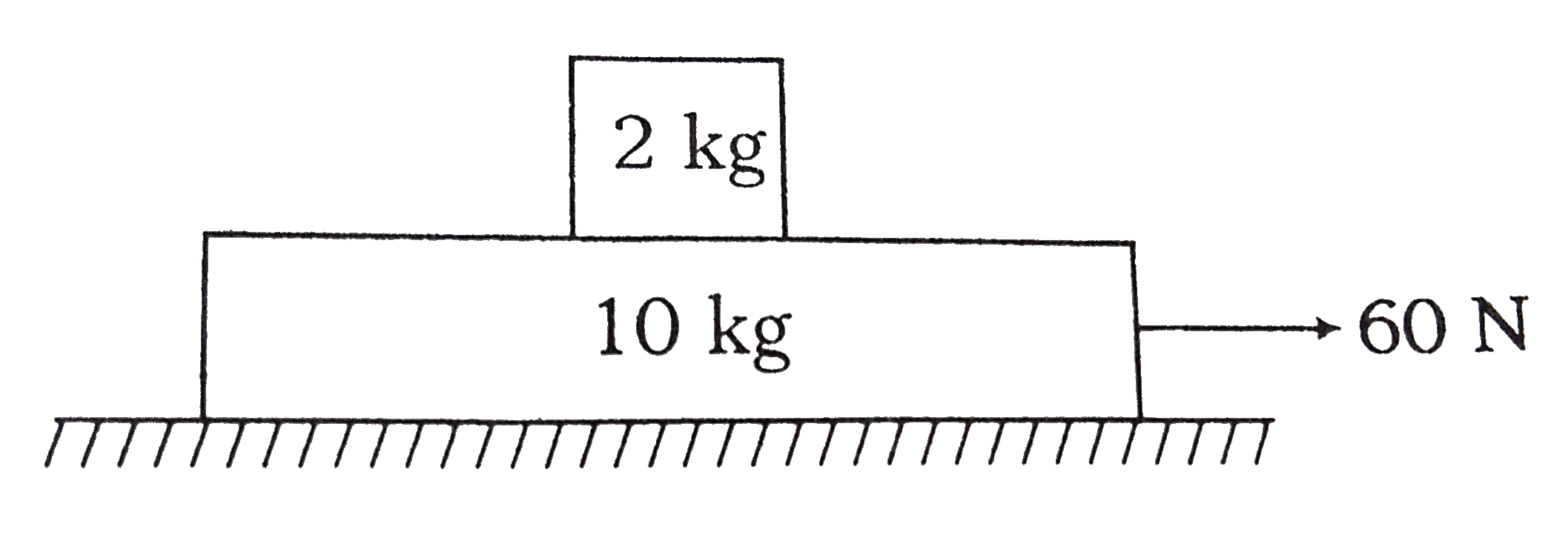
View SolutionA plank of mass 10 kg and a block of mass 2 kg are placed on a horizontal plane as shown in the figure. There is no friction between plane and plank. The coefficient of friction between block and plank is 0.5 .A force of 60 N is applied on plank horizontally. In first 2 s the work done by friction on the block is
View SolutionA block of mass m=4kg is placed over a rough inclined plane as shown in fig . The coefficient of friction between the block and the plane μ=0.5 A force F=10N is applied on the block at an angle of 30∘. Find the contact force between the block and the plane.
k

View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal. If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View Solution
