Statement-I : In Rutherford's gold foil experiment, very few α-particles are deflected back.
Because
Statement-II : Nucleus present inside the atom is heavy.
Both 'A' and 'R' are true and 'R' is the correct explanation of 'A'.
Both 'A' and 'R' are true and 'R' is not the correct explanation of 'A'
'A' is true and 'R' is false
'A' is false and 'R' is true
The correct Answer is:B
Most of the particles move without any deflection. This is because most of the space inside the atom is empty. Some of the the particles get deflected through small angles, this shows the presence of the positive charge concentration in the centre of each atom i.e the nucleus.
Topper's Solved these Questions
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE -II
35 Videos
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE -III
25 Videos
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE -IA
51 Videos
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION
128 Videos
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (ADVANCED) (Integer Type Questions)
2 Videos
Similar Questions
Assertion (A) : In rutherford's gold foil experiment, very few α particle are deflected back
Reason (R ) : Nuclear present inside the atom is heavy
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
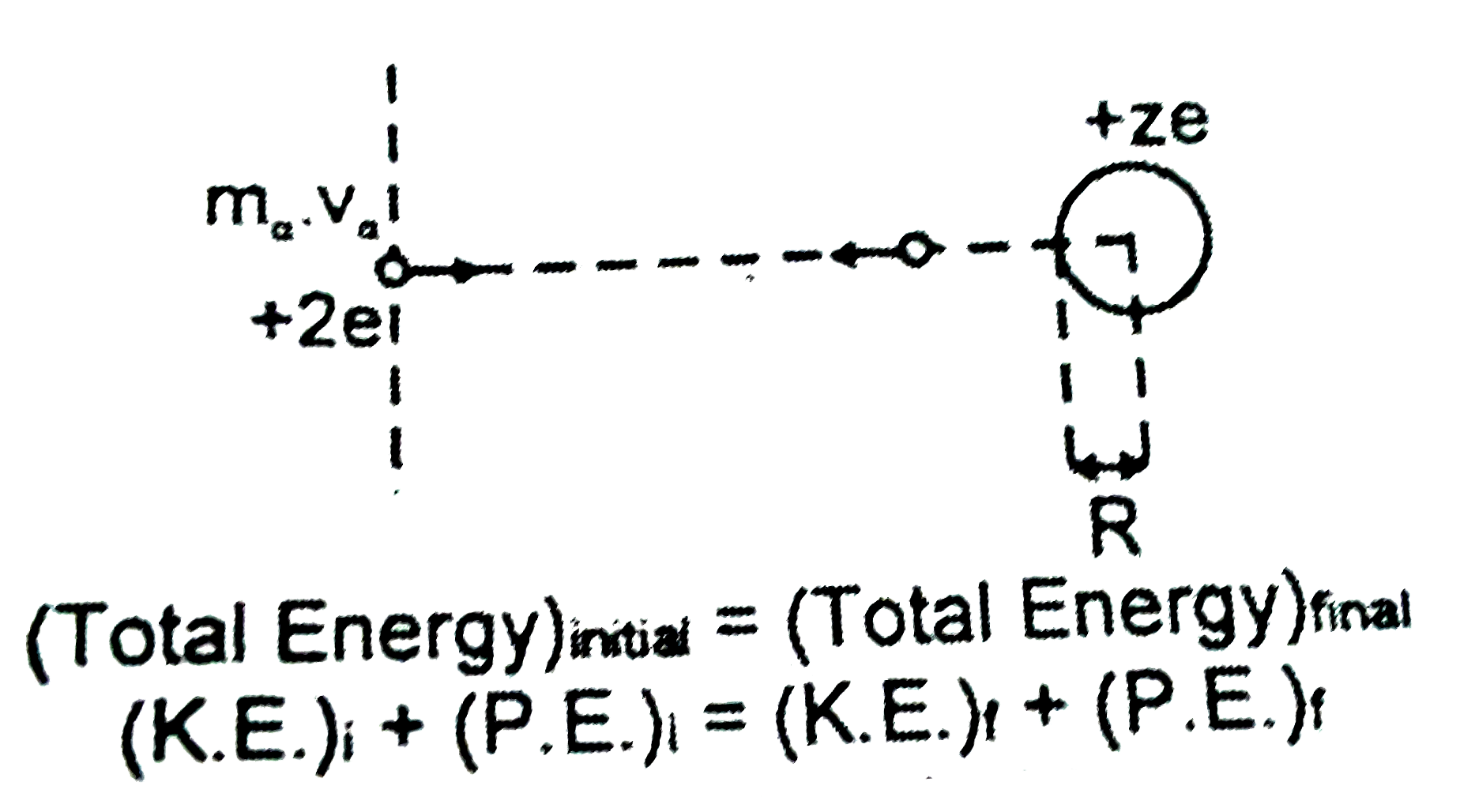
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
Radius of a particular nucleus is calculated by the projection of α-particle from infinity at a particular speed. Let this radius is the true radius . If the radius calculation for the same nucleus is made by another α-particle with half of the earlier speed, then the percentage error involved in the radius calculation is :
View Solution
Knowledge Check
Assertion : In Rutherford's α-particle scattering experiment, most of the α-particles were deflected by nearly 180∘.
Reason : The positive charge of the atom is spread throughout the atom that repelled and deflected the positively charged α-particles.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Statement -1 : Large angle scattering of alpha particles led to the discovery of atomic nucleus.
Statement -2 : Entire positive charge of atom is concentrated in the central core.Aif both assertion and reason are true and reason is the correct explanation of assertion.Bif both assertion and reason are true but reason is not the correct explanation of assertion.Cif assertion is true but reason is false.Dif both assertion and reason are false.Assertion : During α -particles scattering experiment, 99% of the α-particles get deflected through very large angles.
Reason : The nuclei of atoms are positively charged and repel α-particles which are also positively charged.AIf both Assertion and Reason are correct and Reason is the correct explanation of Assertion.BIf both Assertion and Reason are correct and Reason is not the correct explanation of Assertion.CIf Assertion is correct but Reason is incorrect.DIf Assertion is incorrect but Reason is correct.
Rutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
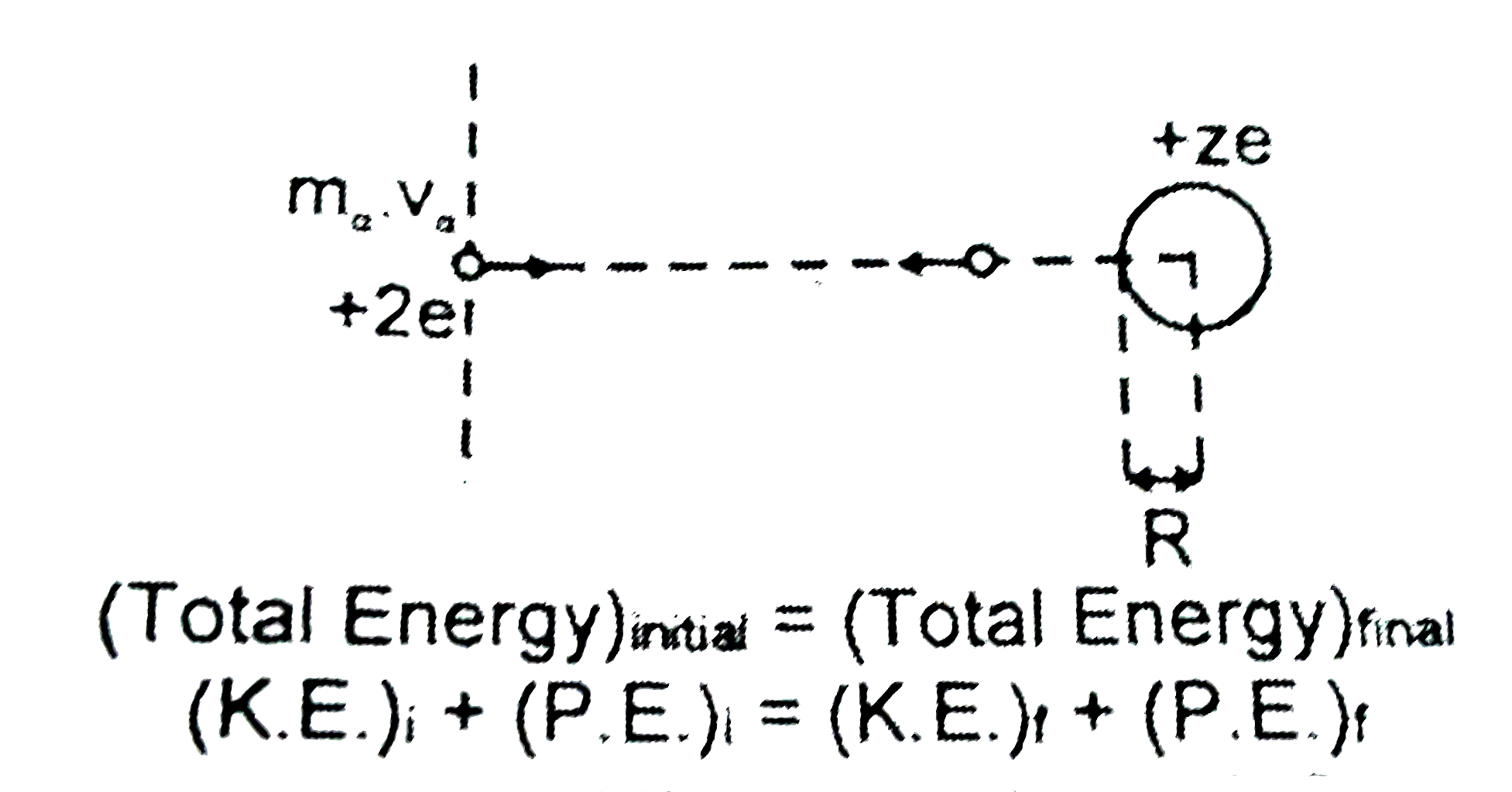
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionStatement-I : No two electrons in an atom can have the same values of four quantum number.
Because
Statement-II : No two electrons in an atom can be simultaneously in the same shell, same subshell, same orbitals and have same spin.
View SolutionIn the gold foil experiment of Geiger and Marsden, that paved the way for Rutherford's model of an atom, ~1.00% of the α-particles were found to deflect at angles >50∘. If one mole of α-particles were bombarded on the gold foil, compute the number of α-particles that would deflect at angles less than 50∘.
View SolutionAssertion: In Rutherford's experiment, a-particles from a sodium source were allowed to fall on a 104 mm thick gold foil. Most of the particles passed straight through the foil.
Reason : The entire positive charge and nearly whole of the mass of an atom is concentrated in the nucleus.
View SolutionWhich of the following statement about Rutherford's model of atom are correct?
(i) Considered the nucleus as positively charged.
(ii) Established that the α-particles are four times as heavy as a hydrogen atom.
(iii) Can be compared to solar system.
(iv) Was in agreement with Thomson's model.
View Solution
