Two point charges of +Q each have been placed at the positions .The locus of the points where - Q charge can be placed such the that total electrostatic potential energy of the system can become equal to zero, is represented by which of the following equations ?
A)
B)
C)
D) None
The correct Answer is:C
To solve the problem, we need to find the locus of points where a charge of -Q can be placed such that the total electrostatic potential energy of the system becomes zero. We have two positive charges of +Q each located at (-a/2, 0, 0) and (a/2, 0, 0).
1. Identify the Positions of Charges:
- The two positive charges are located at:
- Charge 1: Q1=+Q at (−a2,0,0)
- Charge 2: Q2=+Q at (a2,0,0)
- The negative charge Q3=−Q will be placed at a point (0,y,z).
2. Calculate Distances:
- The distance between the two positive charges is a.
- The distance from the negative charge to each positive charge can be calculated using the distance formula:
- Distance from Q1 to Q3:
r1=√(0+a2)2+y2+z2=√a24+y2+z2
- Distance from Q2 to Q3:
r2=√(0−a2)2+y2+z2=√a24+y2+z2
3. Write the Expression for Total Electrostatic Potential Energy:
- The potential energy U of the system is given by the formula:
U=k(Q2a−Q⋅(−Q)r1−Q⋅(−Q)r2)
- Substituting the distances:
U=k⎛⎜
⎜⎝Q2a+Q2√a24+y2+z2+Q2√a24+y2+z2⎞⎟
⎟⎠
- Simplifying gives:
U=k⎛⎜
⎜⎝Q2a+2⋅Q2√a24+y2+z2⎞⎟
⎟⎠
4. Set Total Potential Energy to Zero:
- For the total potential energy to be zero:
Q2a+2⋅Q2√a24+y2+z2=0
- This implies:
1a=−2√a24+y2+z2
5. Cross-Multiply and Square Both Sides:
- Cross-multiplying gives:
√a24+y2+z2=2a
- Squaring both sides results in:
a24+y2+z2=4a2
- Rearranging gives:
y2+z2=4a2−a24=16a2−a24=15a24
6. Final Equation:
- The equation representing the locus of points where the charge -Q can be placed is:
y2+z2=15a24
Conclusion:
The correct option that represents the locus of points is:
Option C: z2+y2=15a24.
- The two positive charges are located at:
- Charge 1: Q1=+Q at (−a2,0,0)
- Charge 2: Q2=+Q at (a2,0,0)
- The negative charge Q3=−Q will be placed at a point (0,y,z).
- The distance between the two positive charges is a.
- The distance from the negative charge to each positive charge can be calculated using the distance formula:
- Distance from Q1 to Q3:
r1=√(0+a2)2+y2+z2=√a24+y2+z2
- Distance from Q2 to Q3:
r2=√(0−a2)2+y2+z2=√a24+y2+z2
- The potential energy U of the system is given by the formula:
U=k(Q2a−Q⋅(−Q)r1−Q⋅(−Q)r2)
- Substituting the distances:
U=k⎛⎜
⎜⎝Q2a+Q2√a24+y2+z2+Q2√a24+y2+z2⎞⎟
⎟⎠
- Simplifying gives:
U=k⎛⎜
⎜⎝Q2a+2⋅Q2√a24+y2+z2⎞⎟
⎟⎠
- For the total potential energy to be zero:
Q2a+2⋅Q2√a24+y2+z2=0
- This implies:
1a=−2√a24+y2+z2
- Cross-multiplying gives:
√a24+y2+z2=2a
- Squaring both sides results in:
a24+y2+z2=4a2
- Rearranging gives:
y2+z2=4a2−a24=16a2−a24=15a24
- The equation representing the locus of points where the charge -Q can be placed is:
y2+z2=15a24
The correct option that represents the locus of points is:
Option C: z2+y2=15a24.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS)6videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) LINKED COMPPREHENSION TYPE QUESTIONS)3videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL -II LECTURE SHEET (ADVANCED)INTEGER TYPE QUESTIONS)2videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - ADDITIONAL EXERCISE13videos
ELECTROMAGNETICS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS10videos
ELEMENTS OF VECTORS
Similar Questions
Two identical charge of value Q each are placed at (-a,0) and (a , 0) . The end coordinates of the point where the net electric field is zero and maximum are respectively-
View SolutionTwo point charges 2q and 8q are placed at a distance r apart. Where should a third charge −q be placed between them so that the electrical potential energy of the system is a minimum
View Solution
Knowledge Check
- Question 1 - Select One
Two charges of magnitude 5nCand−2nC are placed at points (2cm,0,0) and (x cm,0,0) in a region of space. Where there is no other external field. If the electrostatic potential energy of the system is −0.5μJ. What is the value of x ?
A20 cmB80 cmC4 cmD16 cm - Question 2 - Select One
The electrostatic potential energy of two point charges, 1μC each, placed 1 metre apart in air is :
A9×103JB9×109JC9×10−3JD9×10−3J - Question 3 - Select One
A point charge .q. is kept at each of the vertices of an equilateral triangle having each side .a.. Total electrostatic potential energy of the system is:
A(14πε0)3q2a2B(14πε0)3qaC(14πε0)3q2aD(14πε0)3qa2
Two point charges q and -2q are kept on x-axis at ( -a,0,0) and ( 2a,0,0) . Find the locus of all points wher electric potential is zero. Assume that electric potential at infinite distance from a point charge is zero.
View SolutionTwo point charges (Q each are placed at (0,y) and (0-y) A point charge q of the same polarity can move along the x-axis. Then
View SolutionTwo point charges of magnitude +qand−q are placed at (−d/2,0,0)and(d/2,0,0) are respectively. Find the equation of the euipotential surface where the potential is zero.
View SolutionSix equal point charges q each are placed at six corners of a hexago of side a. Find out potential energy of charge system
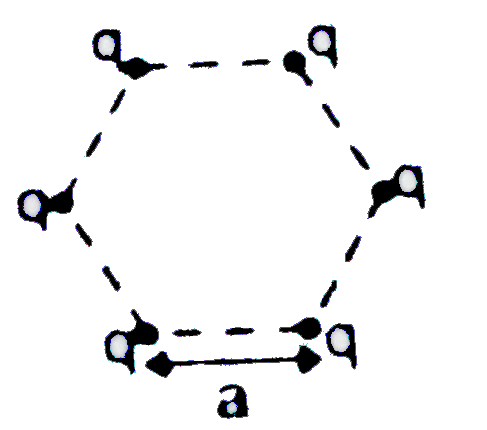 View Solution
View SolutionThree charges −q,+Q and −q are placed in a straight line as shown

If the total potential energy of the system is zero, then the ratio qQ isView Solution

