Find the capacitance of a system of two identical metal balls of radius . a . if the distance between their centres in equal to .b., with to b>>a The system is located in a uniform dielectric with relative permittivity ε
C=πε0εa
C=5πε0εa
C=2πε0εa
C=2πε0ε/a
The correct Answer is:C
To find the capacitance of a system of two identical metal balls of radius a with the distance between their centers equal to b (where b≫a), located in a uniform dielectric with relative permittivity ϵ, we can follow these steps:
1. Understand the Configuration:
- We have two identical metal spheres (balls) each with radius a.
- The distance between their centers is b, and it is given that b≫a.
- The system is placed in a dielectric medium characterized by its relative permittivity ϵ.
2. Capacitance of a Single Sphere:
- The capacitance C of a single isolated sphere in a medium with permittivity ϵ is given by:
C=4πϵ0ϵa
- Here, ϵ0 is the permittivity of free space.
3. Capacitance of Each Sphere:
- For both spheres, since they are identical, the capacitance for each sphere is:
C1=C2=4πϵ0ϵa
4. Combine the Capacitances:
- When two capacitors are in series, the total capacitance Cnet can be calculated using the formula:
1Cnet=1C1+1C2
- Substituting the values of C1 and C2:
1Cnet=14πϵ0ϵa+14πϵ0ϵa
- This simplifies to:
1Cnet=24πϵ0ϵa
5. Calculate the Net Capacitance:
- Inverting the equation gives:
Cnet=4πϵ0ϵa2=2πϵ0ϵa
6. Final Result:
- Thus, the capacitance of the system of two identical metal balls is:
Cnet=2πϵ0ϵa
Conclusion:
The capacitance of the system of two identical metal balls is given by:
C=2πϵ0ϵa
- We have two identical metal spheres (balls) each with radius a.
- The distance between their centers is b, and it is given that b≫a.
- The system is placed in a dielectric medium characterized by its relative permittivity ϵ.
- The capacitance C of a single isolated sphere in a medium with permittivity ϵ is given by:
C=4πϵ0ϵa
- Here, ϵ0 is the permittivity of free space.
- For both spheres, since they are identical, the capacitance for each sphere is:
C1=C2=4πϵ0ϵa
- When two capacitors are in series, the total capacitance Cnet can be calculated using the formula:
1Cnet=1C1+1C2
- Substituting the values of C1 and C2:
1Cnet=14πϵ0ϵa+14πϵ0ϵa
- This simplifies to:
1Cnet=24πϵ0ϵa
- Inverting the equation gives:
Cnet=4πϵ0ϵa2=2πϵ0ϵa
- Thus, the capacitance of the system of two identical metal balls is:
Cnet=2πϵ0ϵa
The capacitance of the system of two identical metal balls is given by:
C=2πϵ0ϵa
Topper's Solved these Questions
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - LECTURE SHEET (EXERCISE - I) (LEVEL - II (ADVANCED)) (Matrix Matching Type Questions)
2 Videos
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - LECTURE SHEET (EXERCISE - I) (LEVEL - II (ADVANCED)) (Integer Type Questions)
1 Videos
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (ADVANCED) (Integer Type Questions)
2 Videos
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE
21 Videos
- COMMUNICATION SYSTEM BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEM EXERCISE - LECTURE SHEET (LEVEL - I) (MAIN) (EXERCISE - IV) (Straight Objective & More than one Correct Answer Type Questions)
20 Videos
Similar Questions
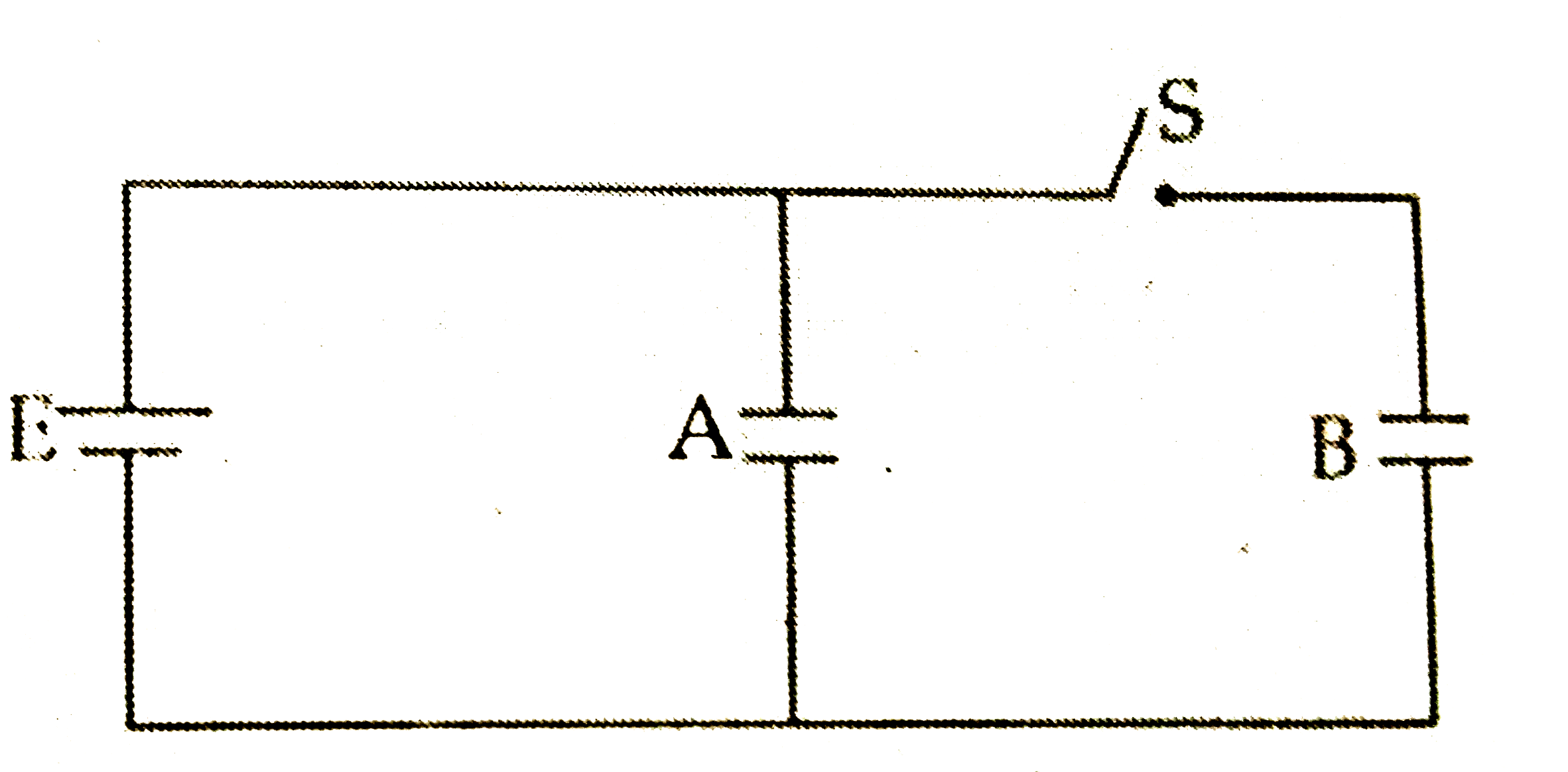
Capacitance of a system of capacitors between points A and B shown in the given figure.
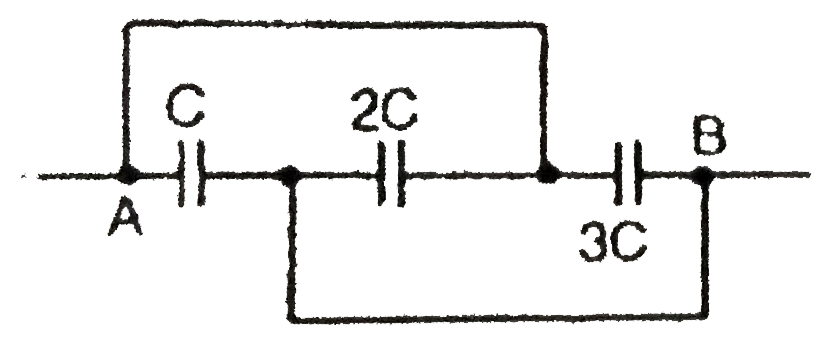
View SolutionFind the equivalent capacitance of the system shown in figure between the points a and b.

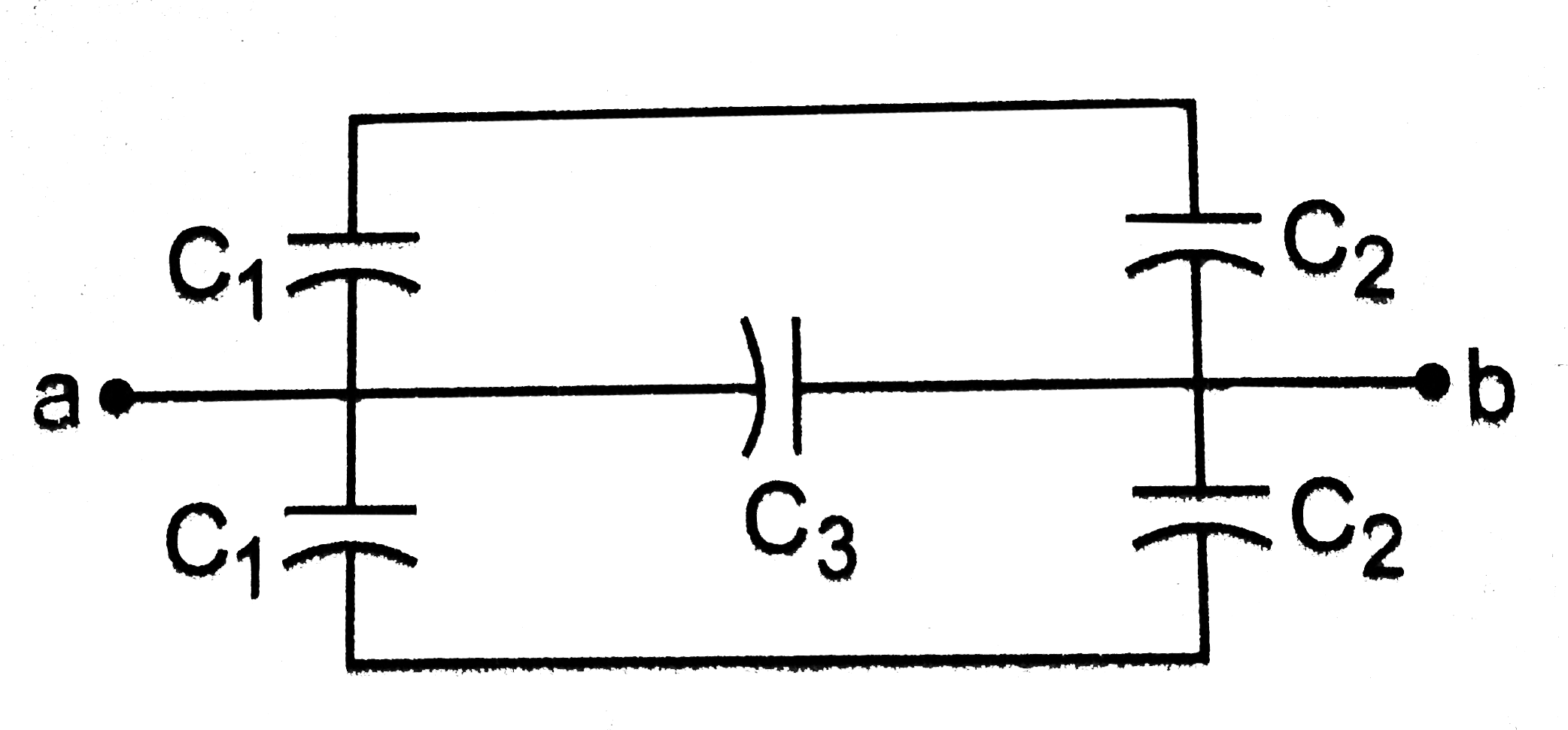
View SolutionFind the equivalent capacitance of the system shown in figure between the points a and b .
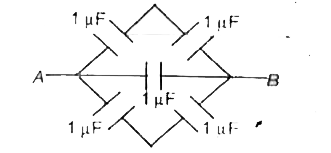
View SolutionThe net capacitance of system of capacitance as shown in the figure between points A and B is
View SolutionCharges 2q and −3q are given to two identical metal plates of area of cross section A. the distance between the plates is d. Find the capacitance and potential difference between the plates.
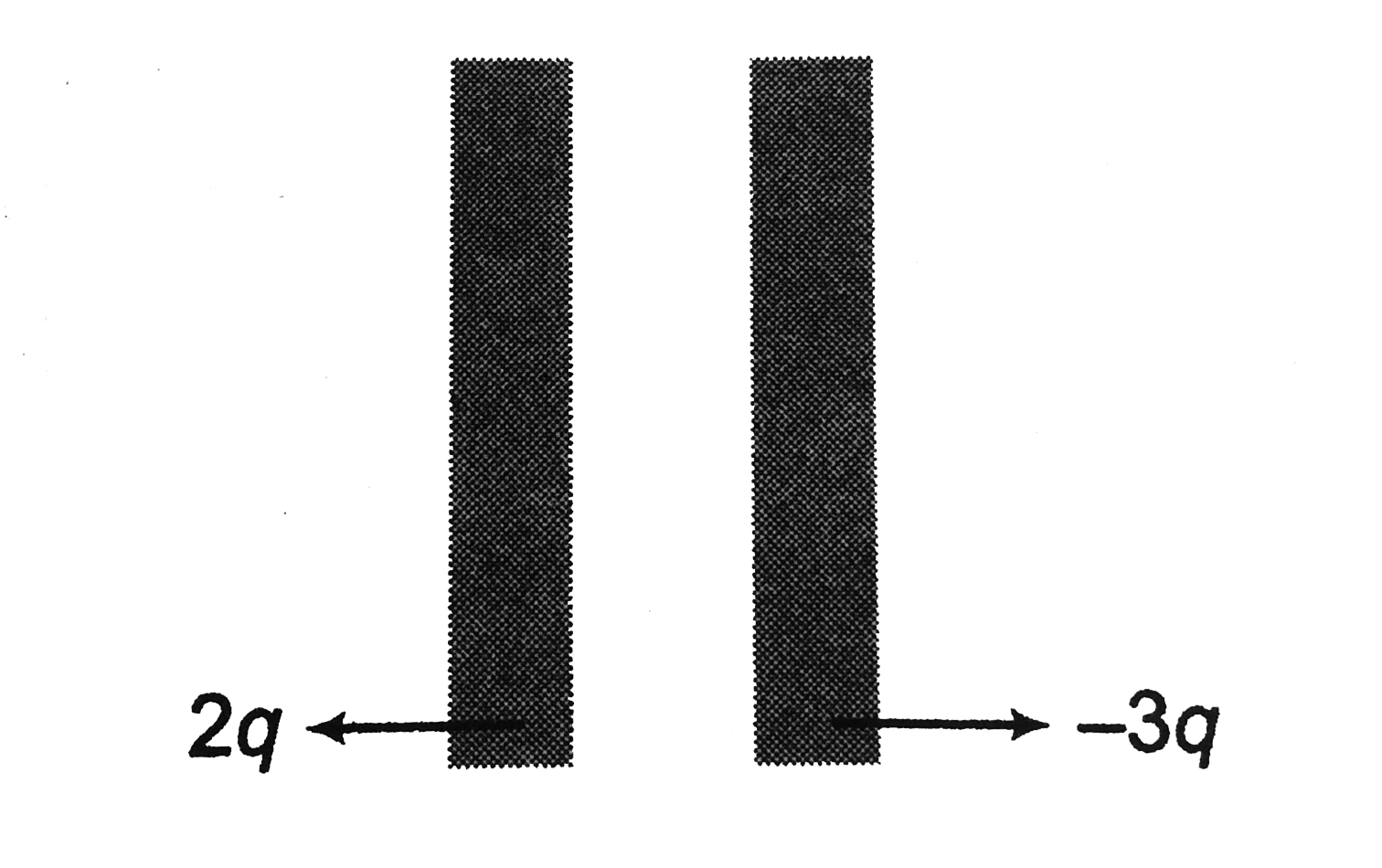
View SolutionThe figure shows two identical parallel plate capacitors connected to a battery with the switch S closed. The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant(or relative permittivity) 3. Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric.
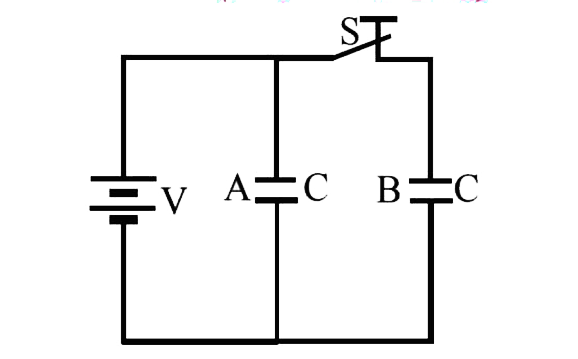
View SolutionTwo identical parallel plate capacitors A and B are
connected to a battery of V volts with the switch S
closed . The switch is now opend and the free
space between the plantes of the capacitors is filled
with a dielectric of dielectric constant K. Find the
rario of the total electrostatic energy stored in both
capacitors before and after the introduction of the dielectric.
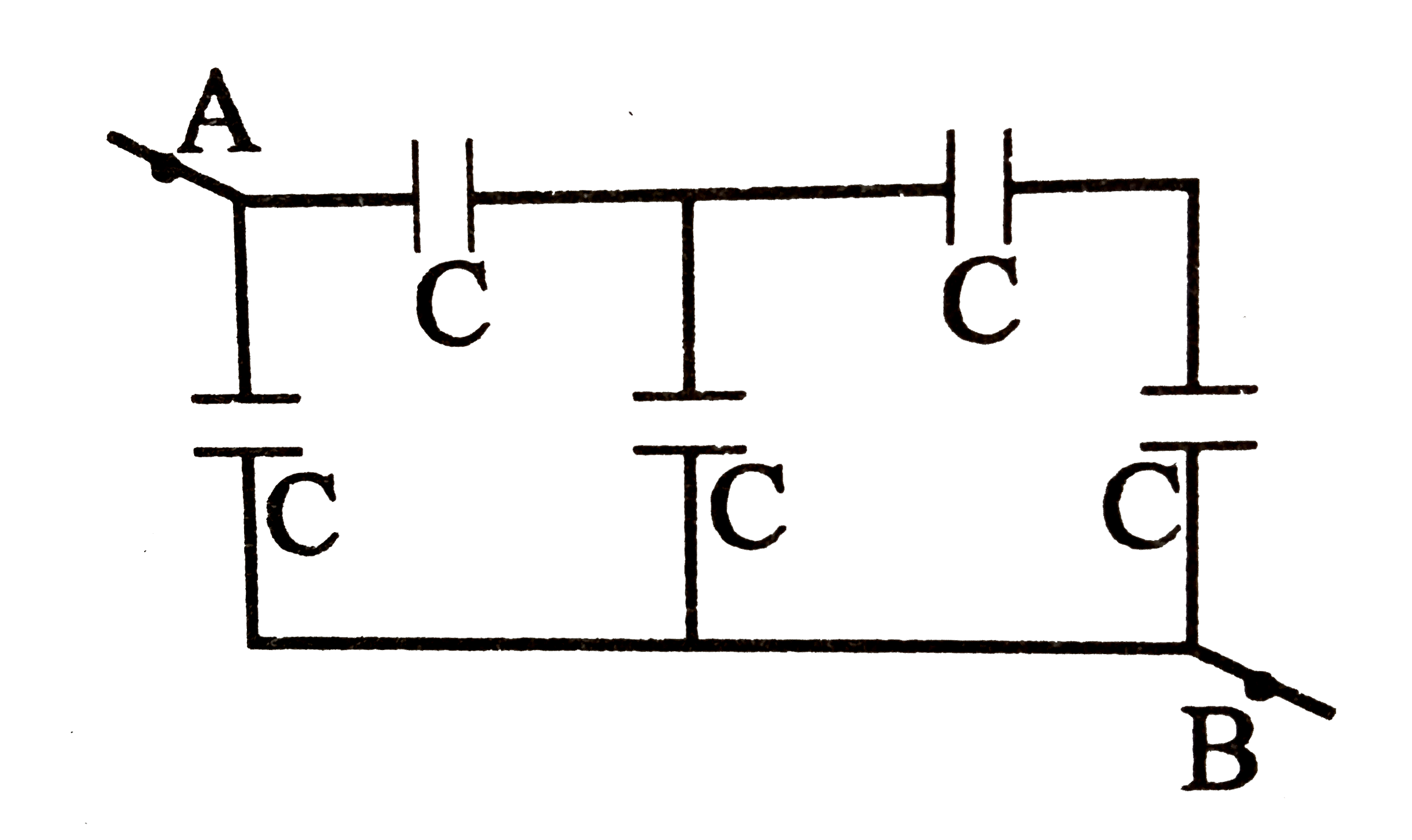
View SolutionWhat is the equivalent capacitance of the system of capacitors between A&B
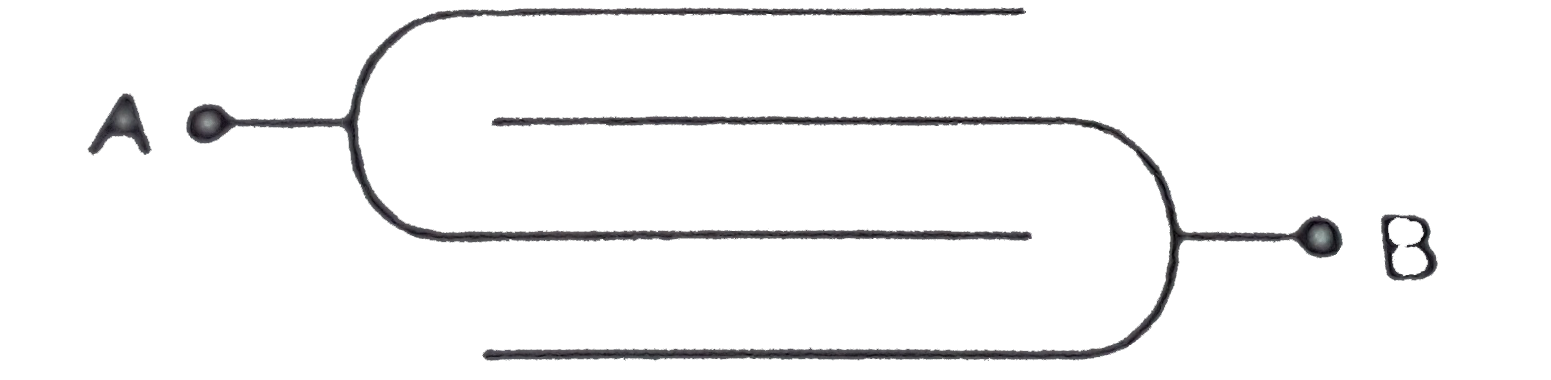
View SolutionFind out equivalent capacitance between A and B. (take each plate Area = A and distance between two conjugative plates is d)
View SolutionFind the equivalent capacitance of each of the two combinations between A and B shown in the figures (a) and (b)
View Solution
