A dielectric slab of length l, width b, thickness d and dielectric constant K fills the space inside a parallel plate capacitor. At t = 0, the slab begins to be pulled out slowly with speed y. At time t, the capacity of the capacitor is
ε0bd[Kl−(K−1)vt]
ε0bb[Kl+(K+1)vt]
ε0bb[(Kl+1)vt]
ε0bb[l+(K−1)vt]
The correct Answer is:A
To solve the problem step by step, we need to analyze the situation of the parallel plate capacitor with a dielectric slab being pulled out.
1. Identify the Parameters:
- Length of the capacitor plates: l
- Width of the capacitor plates: b
- Thickness of the dielectric slab: d
- Dielectric constant of the slab: K
- Speed at which the slab is pulled out: v
- Distance moved by the slab at time t: x=vt
2. Determine the Remaining Distance:
- The remaining distance of the dielectric slab inside the capacitor at time t is:
l−x=l−vt
3. Capacitance Calculation:
- The capacitor can be divided into two parts:
- C1: The part without the dielectric (length x)
- C2: The part with the dielectric (length l−x)
- The areas for these sections are:
- Area of C1: A1=x⋅b=vt⋅b
- Area of C2: A2=(l−x)⋅b=(l−vt)⋅b
4. Capacitance Formulas:
- The capacitance without the dielectric:
C1=A1ϵ0d=(vt⋅b)ϵ0d
- The capacitance with the dielectric:
C2=A2Kϵ0d=((l−vt)⋅b)Kϵ0d
5. Total Capacitance:
- Since C1 and C2 are in parallel, the total capacitance C is:
C=C1+C2
- Substituting the values:
C=(vt⋅b)ϵ0d+((l−vt)⋅b)Kϵ0d
6. Simplifying the Expression:
- Factor out common terms:
C=bϵ0d(vt+K(l−vt))
- Distributing K:
C=bϵ0d(Kl+vt(1−K))
7. Final Expression:
- Therefore, the capacitance of the capacitor at time t is:
C(t)=bϵ0d(Kl+vt(1−K))
- Length of the capacitor plates: l
- Width of the capacitor plates: b
- Thickness of the dielectric slab: d
- Dielectric constant of the slab: K
- Speed at which the slab is pulled out: v
- Distance moved by the slab at time t: x=vt
- The remaining distance of the dielectric slab inside the capacitor at time t is:
l−x=l−vt
- The capacitor can be divided into two parts:
- C1: The part without the dielectric (length x)
- C2: The part with the dielectric (length l−x)
- The areas for these sections are:
- Area of C1: A1=x⋅b=vt⋅b
- Area of C2: A2=(l−x)⋅b=(l−vt)⋅b
- The capacitance without the dielectric:
C1=A1ϵ0d=(vt⋅b)ϵ0d
- The capacitance with the dielectric:
C2=A2Kϵ0d=((l−vt)⋅b)Kϵ0d
- Since C1 and C2 are in parallel, the total capacitance C is:
C=C1+C2
- Substituting the values:
C=(vt⋅b)ϵ0d+((l−vt)⋅b)Kϵ0d
- Factor out common terms:
C=bϵ0d(vt+K(l−vt))
- Distributing K:
C=bϵ0d(Kl+vt(1−K))
- Therefore, the capacitance of the capacitor at time t is:
C(t)=bϵ0d(Kl+vt(1−K))
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (EXERCISE - IV) (LEVEL - II (ADVANCED) (Straight Objective Type Questions )5videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (EXERCISE - IV) (LEVEL - II (ADVANCED) (More than one correct answer Type Questions)4videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (EXERCISE - III) (LEVEL - II (ADVANCED)) (Integer Type Questions)1videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE21videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEM EXERCISE - LECTURE SHEET (LEVEL - I) (MAIN) (EXERCISE - IV) (Straight Objective & More than one Correct Answer Type Questions)20videos
COMMUNICATION SYSTEM
Similar Questions
A parallel plate capacitor has plate of length 'l' width 'w' and separation of plates is 'd' . It is connected to a battery of emf V . A dielectric slab of the same thickness 'd' and of dielectric constant k=4 is being inserted between the plates of the capacitor . At what lenght of the slab inside plates , will the energy stored in the capacitor be two time the initial energy stored ?
View SolutionA dielectric slab of thickness 1.0 cm and dielectric constant 5 is placed between the plates of a parallel plate capacitor of plate area 0.01m2 and separation 2.0 cm. Calculate the change in capacity on introduction of dielectric. What would be on the change, if the dielectric slab were conducting?
View SolutionShown a parallel plate capacitor with plates of width b and length l. The separation between the platesis d. The plates are rigidly clamped and connected to a battery of emf V. A dielectric slab of thickness d and dielectric constant K is slowly inserted between the plates. (a) Calculate the energy of the system when a length x of the slab is introduced into the capacitor. (b) what force should be applied on the slab to ensure that is goes slowly into the capacitor? Neglect any effect of friction or gravity.
 View Solution
View SolutionA parallel plate capacitor of capacitance C (without dielectric) is filled by dielectric slabs as shown in figure. Then the new capacitance of the capacitor is
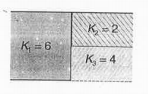 View Solution
View SolutionIf a dielectric slab of thickness t and area A is inserted in between the plates of a parallel plate capacitor of plate area A and distance between the plates d(d>t) then find out capacitance of system. What do you predict about the dependence of capacitance on location of slab?
View SolutionA slab of materail of dielectric constant K has the same area as the plates of a parallel plate capacitor but has thickness d/2, where d is the separation between the plates. Find the expression for the capacitance when the slab is inserted between the plates.
View SolutionFigure shows a parallel-plate capacitor with plates of width b and length l. The separation between the plates is d. The plates are rigidly clamped and connected to a battery of emf V. A dielectric slab of thickness d and dielectric constant K is slowly inserted between the plates. Calculate the energy of the system when a length x of the slab is introduced into the capacitor.
 View Solution
View SolutionWhen a dielectric slab of thickness 6 cm is introduced between the plates of parallel plate condenser, it is found that the distance between the plates has to be increased by 4 cm to restore to capacity original value. The dielectric constant of the slab is
View SolutionA slab of copper of thickness b is inserted in between the plates of parallel plate capacitor as shown in figure. The separation of the plate is d. If b=d/2, then the ratio of capacities of the capacitor after and before inserting that slab will be
 View Solution
View SolutionA slab of copper of thickness y is inserted between the plates of parallel plate capacitor as shown in figure. The separation between the plates is d. If y=d4, then the ratio of capacitance of the capacitor after nd before inserting the slab is
 View Solution
View Solution

