The plates of a parallel plate capacitor have surface area .A. and are initially separated by a distance .d.. They are connected to a battery of voltage v0 Now, the plates of the capacitor are pulled apart w a separation 2d. Then, increase in the energy of the battery is
∈0AV204d
∈0AV202d
∈0AV208d
there will be no change in the energy of the battery
The correct Answer is:B
To solve the problem step by step, we will analyze the situation of the parallel plate capacitor before and after the plates are pulled apart.
Step 1: Understand the initial conditions
- The initial capacitance C of a parallel plate capacitor is given by the formula:
C=ϵ0Ad
where ϵ0 is the permittivity of free space, A is the surface area of the plates, and d is the distance between the plates.
Step 2: Determine the initial energy stored in the capacitor
- The energy U stored in a capacitor is given by:
U=12QV
Since the capacitor is connected to a battery of voltage V0, the charge Q on the capacitor is:
Q=CV0=ϵ0AdV0
- Thus, the initial energy Ui is:
Ui=12QV0=12(ϵ0AdV0)V0=ϵ0AV202d
Step 3: Analyze the new conditions after pulling the plates apart
- When the plates are pulled apart to a distance of 2d, the new capacitance C′ becomes:
C′=ϵ0A2d
Step 4: Calculate the new energy stored in the capacitor
- The charge Q remains the same since the battery is still connected, so:
Uf=12Q2C′=12Q2ϵ0A2d=Q2⋅2d2ϵ0A
- Substituting Q=ϵ0AdV0:
Uf=12(ϵ0AdV0)2ϵ0A2d=12⋅ϵ20A2V20d2⋅2dϵ0A=ϵ0AV20d
Step 5: Calculate the increase in energy of the battery
- The increase in energy ΔU is given by:
ΔU=Uf−Ui
- Substituting the expressions for Uf and Ui:
ΔU=ϵ0AV20d−ϵ0AV202d=ϵ0AV20d−ϵ0AV202d=ϵ0AV202d
Final Answer:
The increase in the energy of the battery is:
ΔU=ϵ0AV202d
- The initial capacitance C of a parallel plate capacitor is given by the formula:
C=ϵ0Ad
where ϵ0 is the permittivity of free space, A is the surface area of the plates, and d is the distance between the plates.
- The energy U stored in a capacitor is given by:
U=12QV
Since the capacitor is connected to a battery of voltage V0, the charge Q on the capacitor is:
Q=CV0=ϵ0AdV0
- Thus, the initial energy Ui is:
Ui=12QV0=12(ϵ0AdV0)V0=ϵ0AV202d
- When the plates are pulled apart to a distance of 2d, the new capacitance C′ becomes:
C′=ϵ0A2d
- The charge Q remains the same since the battery is still connected, so:
Uf=12Q2C′=12Q2ϵ0A2d=Q2⋅2d2ϵ0A
- Substituting Q=ϵ0AdV0:
Uf=12(ϵ0AdV0)2ϵ0A2d=12⋅ϵ20A2V20d2⋅2dϵ0A=ϵ0AV20d
- The increase in energy ΔU is given by:
ΔU=Uf−Ui
- Substituting the expressions for Uf and Ui:
ΔU=ϵ0AV20d−ϵ0AV202d=ϵ0AV20d−ϵ0AV202d=ϵ0AV202d
The increase in the energy of the battery is:
ΔU=ϵ0AV202d
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II LECTURE SHEET (ADVANCED))(Straight Objective Type Questions)9videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II LECTURE SHEET (ADVANCED))(Matrix Matching Type Questions)1videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (EXERCISE - IV) (LEVEL - II (ADVANCED) (More than one correct answer Type Questions)4videos
CAPACITORS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE21videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEM EXERCISE - LECTURE SHEET (LEVEL - I) (MAIN) (EXERCISE - IV) (Straight Objective & More than one Correct Answer Type Questions)20videos
COMMUNICATION SYSTEM
Similar Questions
Knowledge Check
- Question 1 - Select One
A parallel plate capacitor without any dielectric within its plates, has a capacitance C, and is connected to a battery of emf V. The battery is disconnected and the plates of the capacitor are pulled apart until the separation between the plates is doubled. What is the work done by the agent pulling the plates apart, in this process ?
A12CV2B32CV2C−32CV2DCV2
Two plates of a parallel plate capacitor carry charges q and -q and are separated by a distance a from each other. The capacitor is connected to a constant voltage source V0. The distance between the plates is changed to x+dx. Then in steady state.
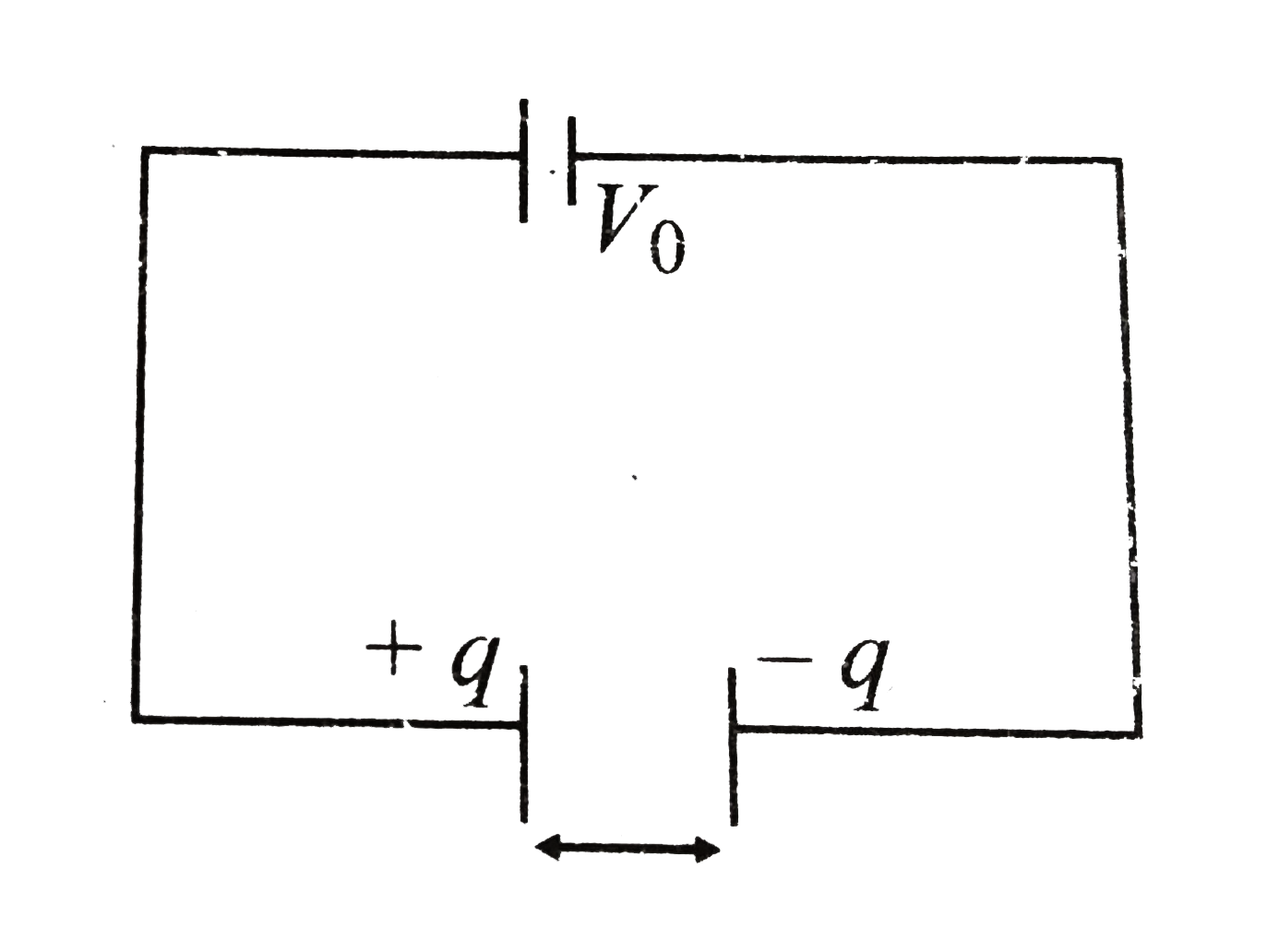 View Solution
View SolutionA parallel plate capacitor is charged. If the plates are pulled apart
View SolutionA parallel plate capacitor has plate A and separation d between the plates. The capacitor is connected to a battery of emf V. (a) find the charge on the capacitor. (b) the plate separation is decreases to d/2. Find the extra charge given by the battery to the positive plate. (c) work done in reducing separation between plates.
View SolutionEach plate of a parallel plate capacitor has a charge q on it. The capacitor is now connected to a battery. Now,
View SolutionA pararllel plate capacitor has plates of area A and separation d and is charged to potential diference V. The charging battery is then disconnected and the plates are pulle apart until their separation is 2d. What is the work required to separate the plates?
View SolutionA parallel plate capacitor is charged and then isolated. On increasing the plate separation
View SolutionImagine a light planet revolving around a very massive star in a circular orbit of radius r with direction T. On what power of r, will the square of time period depends if the gravitational force of attraction between the planet and the star is proportional to r−5/2 .
View Solution

