Find the ratio of escape velocities of two planets if values of g on the two planets are 9.8m/s2 and 3.3m/s2 and ther radii are 6400 km and 3400 km respectively.
To find the ratio of escape velocities of two planets given their gravitational accelerations and radii, we can follow these steps:
Step 1: Understand the formula for escape velocity
The escape velocity ve from the surface of a planet is given by the formula:
ve=√2gR
where g is the acceleration due to gravity on the planet, and R is the radius of the planet.
Step 2: Write the escape velocity for both planets
Let’s denote the first planet as Planet 1 and the second planet as Planet 2. The escape velocities for both planets can be expressed as:
ve1=√2g1R1
ve2=√2g2R2
Step 3: Find the ratio of escape velocities
To find the ratio of the escape velocities ve1ve2, we can set up the equation:
ve1ve2=√2g1R1√2g2R2=√g1R1g2R2
Step 4: Substitute the given values
From the problem, we have:
- g1=9.8m/s2
- g2=3.3m/s2
- R1=6400km=6400×103m
- R2=3400km=3400×103m
Now substituting these values into the ratio:
ve1ve2=
⎷9.8×(6400×103)3.3×(3400×103)
Step 5: Simplify the expression
Calculating the values inside the square root:
=√9.8×64003.3×3400
Calculating the numerator and denominator:
- Numerator: 9.8×6400=62720
- Denominator: 3.3×3400=11262
Now substituting these values:
=√6272011262≈√5.57≈2.36
Step 6: Final ratio
Thus, the ratio of escape velocities is approximately:
ve1ve2≈2.36:1
Conclusion
The final answer is:
The ratio of escape velocities ve1:ve2≈2.36:1
The escape velocity ve from the surface of a planet is given by the formula:
ve=√2gR
where g is the acceleration due to gravity on the planet, and R is the radius of the planet.
Let’s denote the first planet as Planet 1 and the second planet as Planet 2. The escape velocities for both planets can be expressed as:
ve1=√2g1R1
ve2=√2g2R2
To find the ratio of the escape velocities ve1ve2, we can set up the equation:
ve1ve2=√2g1R1√2g2R2=√g1R1g2R2
From the problem, we have:
- g1=9.8m/s2
- g2=3.3m/s2
- R1=6400km=6400×103m
- R2=3400km=3400×103m
ve1ve2=
⎷9.8×(6400×103)3.3×(3400×103)
Calculating the values inside the square root:
=√9.8×64003.3×3400
- Numerator: 9.8×6400=62720
- Denominator: 3.3×3400=11262
=√6272011262≈√5.57≈2.36
Thus, the ratio of escape velocities is approximately:
ve1ve2≈2.36:1
The final answer is:
The ratio of escape velocities ve1:ve2≈2.36:1
Similar Questions
Find the ratio of 3 km to 300 m
View SolutionWhat is the ratio of the escape velcoities from two planets of equal densities but different masses M1 and M2 ?
View SolutionThe ratio of the escape speed from two planets is 3 : 4 and the ratio of their masses is 9 : 16. What is the ratio of their radii ?
View SolutionCompare the time periods of a simple pendulum at places where g is 9.8ms−2 and 4.36 m s−2 respectively .
View SolutionIf g on the surface of the earth is 9.8m/s2, find its value at a height of 6400 km.
(Radius of the earth = 6400 km)View SolutionThere are two planets and the ratio of radius of the two planets is k but ratio of acceleration due to gravity of both planets is g. What will be the ratio of their escape velocities ?
View SolutionA 10 kg steel ball is suspended by two strings as shown. The tensions in the two strings are: (g=9.8 m/s2)
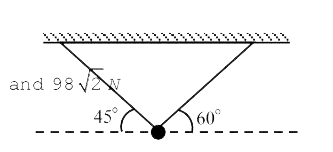 View Solution
View SolutionThe ratio of the masses and radii of two planets are 2:3 and 8:27.The ratio of respective escape speeds from their surfaces are
View SolutionThe escape velocity corresponding to a planet of mass M and radius R is 50kms−1. If the planet's mass and radius were 4M and R, respectively, then the corresponding escape velocity would be
View SolutionThe ratio of the masses and radii of two planets are 2 : 3 and 4 : 9. What is the ratio of the escape speed at their surface ?
View Solution

