A thin uniform ring of radius R carrying charge q and mass m rotates about its axis with angular velocity ω. Find the ratio of its magnetic moment and angular momentum.
To find the ratio of the magnetic moment (μ) and angular momentum (L) of a thin uniform ring of radius R, carrying charge q and mass m, rotating about its axis with angular velocity ω, we can follow these steps:
Step 1: Calculate the Current (I)
The current (I) in the ring can be defined as the charge (q) passing through a point in the ring per unit time. The time period (T) for one complete rotation is given by:
T=2πω
Thus, the current can be calculated as:
I=qT=q2πω=q⋅ω2π
Step 2: Calculate the Area (A) of the Ring
The area (A) of the circular path of the ring is given by the formula for the area of a circle:
A=πR2
Step 3: Calculate the Magnetic Moment (μ)
The magnetic moment (μ) is given by the product of the current (I) and the area (A):
μ=I⋅A=(q⋅ω2π)⋅(πR2)
Simplifying this gives:
μ=q⋅ω⋅R22
Step 4: Calculate the Angular Momentum (L)
The angular momentum (L) for a particle moving in a circular path is given by:
L=Icm⋅ω
For a ring, the moment of inertia (I_cm) about its axis is given by:
Icm=mR2
Thus, the angular momentum can be expressed as:
L=mR2⋅ω
Step 5: Find the Ratio of Magnetic Moment to Angular Momentum
Now, we can find the ratio of the magnetic moment (μ) to the angular momentum (L):
μL=q⋅ω⋅R22mR2⋅ω
Cancelling out common terms (R² and ω):
μL=q2m
Final Result
Thus, the ratio of the magnetic moment to the angular momentum is:
μL=q2m
The current (I) in the ring can be defined as the charge (q) passing through a point in the ring per unit time. The time period (T) for one complete rotation is given by:
The area (A) of the circular path of the ring is given by the formula for the area of a circle:
The magnetic moment (μ) is given by the product of the current (I) and the area (A):
The angular momentum (L) for a particle moving in a circular path is given by:
Now, we can find the ratio of the magnetic moment (μ) to the angular momentum (L):
Thus, the ratio of the magnetic moment to the angular momentum is:
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IA
142 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-IB
71 Videos
- MOTION IN A STRAIGHT LINE BOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - very Short answer type question
15 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE PRACTICE SHEET (ADVANCED) Integer Type Questions
3 Videos
Similar Questions
A ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(iii)Magnetic moment of ring.
View SolutionA thin disc of radius R has charge Q distributed uniformly on its surface. The disc is rotated about one of its diametric axis with angular velocity ω. The magnetic moment of the arrangement is
View Solution
Knowledge Check
A solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
A12MR2ω2BMR2ω2C14MR2ω2D18MR2ω2
A ring of mass m and radius R is being rotated about its axis with angular velocity o. If o increases then tension in ring
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(i)Current produced due to motion of ring
View SolutionSolid uniform conductiong sphere of mass 'm' and charge Q, rotates about its axis of symmetry with constant angular velocity 'ω' then the ratio of magnetic moment to the moment of inertia of the sphere is xQω6m then x is :(Neglect induced charges due to centrifugal force)
View SolutionA long cylinder of radius a carrying a uniform surface charge rotates about its axis with an angular velocity ω. Find the magnetic field energy per unit length of the cylinder if the linear charge density equals λ and μ=1.
View SolutionA rod is rotating with angular velocity ω about axis AB. Find cosθ.

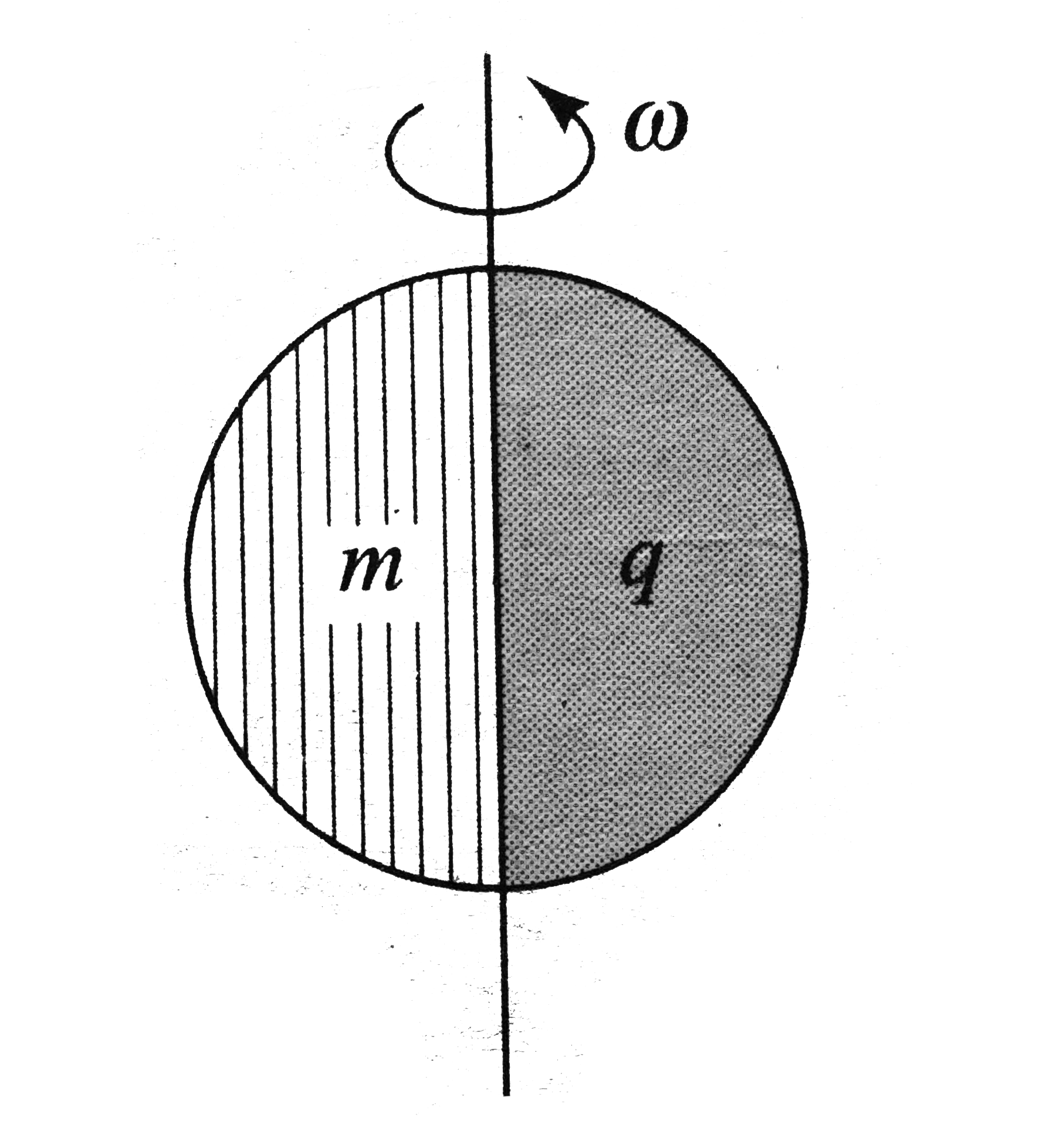
View SolutionConsider a hypothetic spherical body. The body is cut into two parts about the diameter. One of hemispherical portion has mass distribution m while the other portion has indentical charge distribution q. The body is rotated about the axis with constant speed ω. Then, the ratio of magnetic moment to angular momentum is
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
View Solution
