A concave - convex lens has faces of radii 4 cm and 3 cm respectively and is made of glass of refractive index 1.6. Determine
position of image when object is placed at 28 cm in front of the lens
To solve the problem of determining the position of the image formed by a concave-convex lens, we will follow these steps:
Step 1: Identify the given parameters
- Radius of curvature of the first surface (convex side), R1=3cm
- Radius of curvature of the second surface (concave side), R2=−4cm (negative because it is concave)
- Refractive index of the lens material, μ=1.6
- Object distance, u=−28cm (negative as per sign convention)
Step 2: Calculate the focal length using the lens maker's formula
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
Substituting the values:
1f=(1.6−1)(13−1−4)
1f=0.6(13+14)
Finding a common denominator (12):
1f=0.6(412+312)=0.6(712)=0.6×712=4.212=12.857
Calculating f:
f≈2.857cm(approximately 20 cm)
Step 3: Use the lens formula to find the image distance v
The lens formula is:
1f=1v−1u
Rearranging gives:
1v=1f+1u
Substituting the values:
1v=120+1−28
Finding a common denominator (140):
1v=7140−5140=2140=170
Calculating v:
v=70cm
Step 4: Conclusion
The image is formed at v=+70cm on the opposite side of the lens from the object.
- Radius of curvature of the first surface (convex side), R1=3cm
- Radius of curvature of the second surface (concave side), R2=−4cm (negative because it is concave)
- Refractive index of the lens material, μ=1.6
- Object distance, u=−28cm (negative as per sign convention)
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
1f=(1.6−1)(13−1−4)
1f=0.6(13+14)
1f=0.6(412+312)=0.6(712)=0.6×712=4.212=12.857
f≈2.857cm(approximately 20 cm)
The lens formula is:
1f=1v−1u
1v=1f+1u
1v=120+1−28
1v=7140−5140=2140=170
v=70cm
The image is formed at v=+70cm on the opposite side of the lens from the object.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-II)60videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )53videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - PRACTICAL PHYSICSEXERCISE - LECTURE SHEET LEVEL-II (ADVANCED) Linked Comprehension Type Questions4videos
PRACTICAL PHYSICS
- BOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - III3videos
SEMICONDUCTOR DEVICES
Similar Questions
A plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
View SolutionAn equiconvex lens is made from glass of refractive index 1.5. If the radius of each surface is changed from 5cm to 6cm, then the power
View SolutionThe two surfaces of a biconvex lens has same radii of curvatures . This lens is made of glass of refractive index 1.5 and has a focal length of 10 cm in air. The lens is cut into two equal halves along a plane perpendicular to its principal axis to yield two plane - convex lenses. The two pieces are glued such that the convex surfaces touch each other. If this combination lens is immersed in water (refractive index =43 ), its focal length (in cm ) is
View SolutionA biconvex lens has radii of curvature 20 cm and 40cm. The refractive index of the material of the lens is 1.5. An object is placed 40cm in front of the lens. Calculate the psition of the image.
View SolutionA plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?
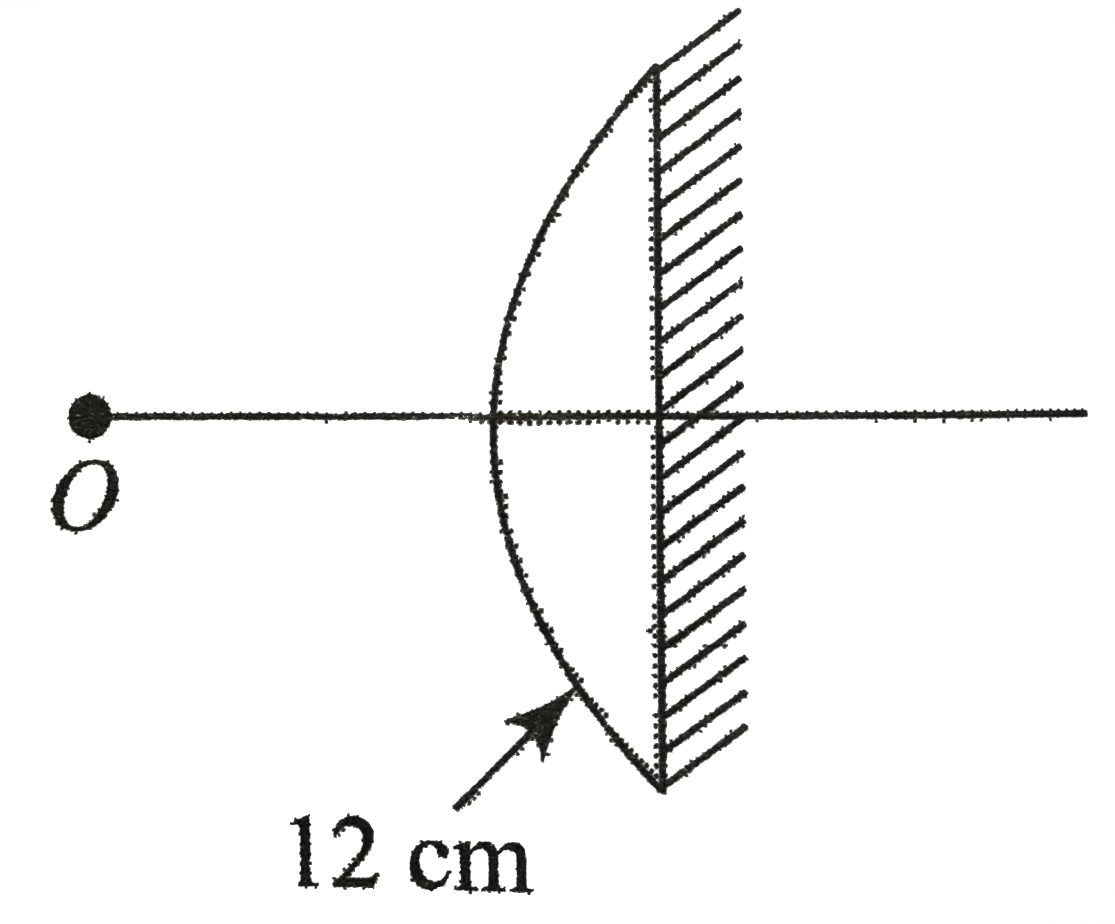 View Solution
View SolutionA convex lens of focal length 20cm is placed 10cm away from a second convex lens of focal length 25 cm. What wil be the location of the image of an object at 30 cm in front of the first lens?
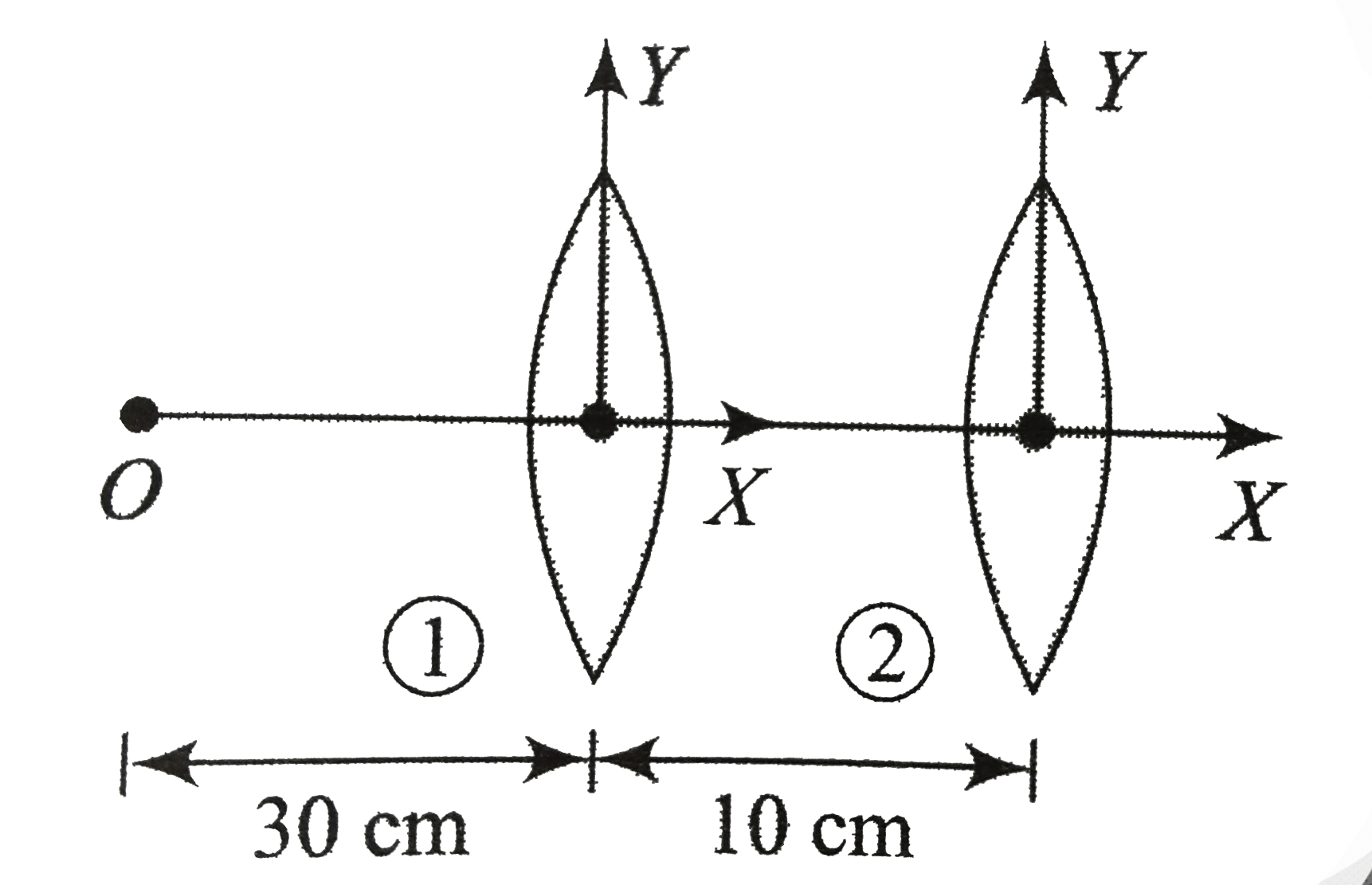 View Solution
View SolutionA thin equiconvex lens has focal length 10 cm and refractive index 1.5 . One of its faces is now silvered and for an object placed at a distance u in front of it, the image coincides with the object. The value of u is
View SolutionA convex lens of focal length 20cm is placed coaxilly with a convex mirror of radius of curvature 20 cm. The two are kept 15 cm apart. A point object is placed 40 cm in front of the convex lens. Find the position of the image formed by this combination. Draw the ray diagram showing the image formation.
View SolutionCalculate the focal length of a convex lens whose radii of curvature of two surfaces is 10cm and 15cm respectively and its refractive index is 1.5.
View SolutionA biconvex lens of focal length 20cm, made of glass or refractive index 1.5, has water (μ=1.33) on one side and air on the other. An object is placed 15 cm form the lens on the side with water. Where is the image formed?
View Solution

