Average marks of class XI are 'X' and when average marks of 15 students reduced by 6, then average marks of class reduced by 7.650. If number of students in class XII is 15 more than that of in class XI and total marks of class XII is 5120, then find average marks of class XII?
60.2
51.2
58.2
67.2
The correct Answer is:D
To solve the problem step by step, we can break it down as follows:
Step 1: Define Variables
Let the average marks of class XI be X and the number of students in class XI be Y.
Step 2: Calculate Total Marks for Class XI
The total marks for class XI can be expressed as:
Total Marks of Class XI=X×Y
Step 3: Understand the Reduction in Average
When the average marks of 15 students are reduced by 6, the total reduction in marks is:
Total Reduction=15×6=90
This reduction causes the average marks of the entire class to decrease by 7.650.
Step 4: Set Up the Equation for Average
The new total marks after the reduction will be:
New Total Marks=XY−90
The new average can be expressed as:
New Average=XY−90Y
According to the problem, this new average is equal to the old average minus 7.650:
XY−90Y=X−7.650
Step 5: Simplify the Equation
Multiply both sides by Y to eliminate the fraction:
XY−90=XY−7.650Y
Now, we can cancel XY from both sides:
−90=−7.650Y
Thus, we can solve for Y:
Y=907.650=11.76470588≈85
Step 6: Determine Number of Students in Class XII
The number of students in class XII is:
Y+15=85+15=100
Step 7: Calculate Average Marks of Class XII
We know the total marks of class XII is 5120. The average marks can be calculated as:
Average Marks of Class XII=Total Marks of Class XIINumber of Students in Class XII=5120100=51.2
Final Answer
The average marks of class XII is 51.2.
---
Let the average marks of class XI be X and the number of students in class XI be Y.
The total marks for class XI can be expressed as:
Total Marks of Class XI=X×Y
When the average marks of 15 students are reduced by 6, the total reduction in marks is:
Total Reduction=15×6=90
This reduction causes the average marks of the entire class to decrease by 7.650.
The new total marks after the reduction will be:
New Total Marks=XY−90
The new average can be expressed as:
New Average=XY−90Y
According to the problem, this new average is equal to the old average minus 7.650:
XY−90Y=X−7.650
Multiply both sides by Y to eliminate the fraction:
XY−90=XY−7.650Y
Now, we can cancel XY from both sides:
−90=−7.650Y
Thus, we can solve for Y:
Y=907.650=11.76470588≈85
The number of students in class XII is:
Y+15=85+15=100
We know the total marks of class XII is 5120. The average marks can be calculated as:
Average Marks of Class XII=Total Marks of Class XIINumber of Students in Class XII=5120100=51.2
The average marks of class XII is 51.2.
Topper's Solved these Questions
- BOOK - ADDA247CHAPTER - AVERAGE AND AGESEXERCISE - Mains Questions20videos
AVERAGE AND AGES
- BOOK - ADDA247CHAPTER - AVERAGE AND AGESEXERCISE - Previous Year Question30videos
AVERAGE AND AGES
- BOOK - ADDA247CHAPTER - AVERAGE AND AGESEXERCISE - Prelims Questions (Level - 1)35videos
AVERAGE AND AGES
- BOOK - ADDA247CHAPTER - BOAT AND STREAM EXERCISE - Previous Year Question22videos
BOAT AND STREAM
Similar Questions
If class mark is 15 and class widht is 10, then find the class.
View SolutionDirection: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The total number of students of class IV and the number of students of class II isView Solution
Knowledge Check
- Question 1 - Select One
A student's mark was wrongly entered as 108 instead of 98 due to this mistake average marks of class got increased by 1/5 total number of students in the class is equal to
A10B30C50D70 - Question 2 - Select One
The average marks obtained by a class of 60 students is 65. The average marks of half of the students is found to be 85. The average marks of the remaining students is
A35B45C55D65 - Question 3 - Select One
Two classes M and n have average marks 25 and 40 respectively, the overall average obtained is 30. The ratio of the students in the class M and N is
A2:1B5:6C5:3D1:2
Direction: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The average number of students of class II and class III isView SolutionDirection: There are 1250 total students in 5 different classes in a school. The number of students in class I is 50 less than the number of students in class II. The number of class IV students is 50 more than the number of students in class II. The average number of students in class III and class V is 250. The number of students in class III is 10% less than the number of students in class I.
The ratio of the total number of students of class V to the total number of students of class III isView SolutionThe average marks of 50 students of a class is 76. If the average marks of all boys is 70 and that of all girls is 80 in that class, then find the number of boys in the class.
View SolutionIn a class of 100 students, there are 70 boys whose average marks in a subject are 75. If the average marks of the complete class is 72, then what is the average marks of the girls ?
View SolutionThe data below shows the number of students in class A having secured different marks.
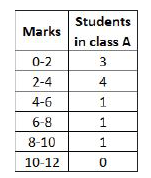
What is the mean marks of class A?View Solution

