Breadth of a rectangular park is 12m and ratio of area of rectangular park to perimeter of rectangular park is, 42:11. If radius of circular park is equal to length of rectangular park and cost of fencing circular park is Rs.20/m, then find total cost of fencing circular park
Rs.2250
Rs.2760
Rs.2800
Rs.2640
The correct Answer is:D
To solve the problem step by step, let's break it down:
Step 1: Understand the Given Information
- The breadth of the rectangular park (b) = 12 m
- The ratio of the area of the rectangular park to its perimeter is 42:11.
- The radius of the circular park is equal to the length of the rectangular park (l).
- The cost of fencing the circular park is Rs. 20 per meter.
Step 2: Set Up the Equations
1. Area of the rectangular park (A):
A=l×b=l×12
2. Perimeter of the rectangular park (P):
P=2(l+b)=2(l+12)
3. Ratio of Area to Perimeter:
AP=4211
Substituting the expressions for A and P:
l×122(l+12)=4211
Step 3: Cross Multiply to Solve for l
Cross multiplying gives:
11×(l×12)=42×2(l+12)
This simplifies to:
11×12l=84(l+12)
132l=84l+1008
Subtracting 84l from both sides:
132l−84l=1008
48l=1008
Dividing both sides by 48:
l=100848=21 m
Step 4: Find the Radius of the Circular Park
Since the radius of the circular park is equal to the length of the rectangular park:
r=l=21 m
Step 5: Calculate the Circumference of the Circular Park
The circumference (C) of the circular park is given by:
C=2πr=2×227×21
Calculating this:
C=447×21=9247=132 m
Step 6: Calculate the Total Cost of Fencing the Circular Park
The total cost (Cost) for fencing is given by:
Cost=Circumference×Cost per meter
Substituting the values:
Cost=132×20=2640 Rs
Final Answer
The total cost of fencing the circular park is Rs. 2640.
---
- The breadth of the rectangular park (b) = 12 m
- The ratio of the area of the rectangular park to its perimeter is 42:11.
- The radius of the circular park is equal to the length of the rectangular park (l).
- The cost of fencing the circular park is Rs. 20 per meter.
1. Area of the rectangular park (A):
A=l×b=l×12
2. Perimeter of the rectangular park (P):
P=2(l+b)=2(l+12)
AP=4211
Substituting the expressions for A and P:
l×122(l+12)=4211
Cross multiplying gives:
11×(l×12)=42×2(l+12)
This simplifies to:
11×12l=84(l+12)
132l=84l+1008
Subtracting 84l from both sides:
132l−84l=1008
48l=1008
Dividing both sides by 48:
l=100848=21 m
Since the radius of the circular park is equal to the length of the rectangular park:
r=l=21 m
The circumference (C) of the circular park is given by:
C=2πr=2×227×21
Calculating this:
C=447×21=9247=132 m
The total cost (Cost) for fencing is given by:
Cost=Circumference×Cost per meter
Substituting the values:
Cost=132×20=2640 Rs
The total cost of fencing the circular park is Rs. 2640.
Topper's Solved these Questions
- BOOK - ADDA247CHAPTER - MENSURATION EXERCISE - MAINS QUESTIONS20videos
MENSURATION
- BOOK - ADDA247CHAPTER - MENSURATION EXERCISE - PREVIOUS YEAR QUESTION31videos
MENSURATION
- BOOK - ADDA247CHAPTER - MENSURATION EXERCISE - PRELIMS QUESTIONS (LEVEL -1)50videos
MENSURATION
- BOOK - ADDA247CHAPTER - INEQUALITYEXERCISE - Previous Year Questions75videos
INEQUALITY
- BOOK - ADDA247CHAPTER - MIXTURE & ALLIGATIONEXERCISE - PREVIOUS YEAR QUESTIONS21videos
MIXTURE & ALLIGATION
Similar Questions
Knowledge Check
- Question 1 - Select One
The side of a square park is 100 m. Perimeter of the park is:
A1000 mB400 mC200 mD800 m - Question 2 - Select One
The sides of a rectangular park are in the ratio 4:3. If its area is 1728 m. find the cost of fencing it at Rs.30 per metre.
ARs 5540BRs 5000CRs 5040Dnone of these
Find the dimensions of a rectangular park whose perimeter is 60 m and area 200 m2
View SolutionThe length and breadth of a rectangular park is 250 m and 125 m respectively. Find the area and perimeter of the park.
View SolutionThe length of a rectangular park is twice its breadth and its perimeter is 840 m. Find the area of the park .
View SolutionThe length of a rectangular park is thrice its breadth. If the perimeter of the park is 168 metres, find its dimensions.
View SolutionTotal cost of fencing the park shown in Fig. 6.17 is Rs 55000. Find the cost of fencing per metre.
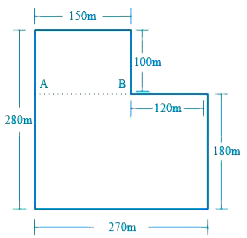 View Solution
View SolutionThe length of a wire which is tied as a boundary of a semi ciruclar park is 72 cm. Find the radius and the area of the semi circular park
View Solution