A particle of mass (m) is moving with constant velocity (v). Show that its angular momentum about any point remains constant throughout the motion.
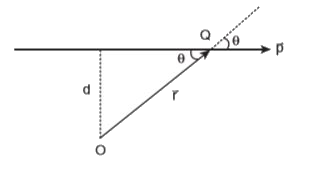
Let the particle of mass m move with constant velocity →v. As it is moving with constant velocity, its path is a straight line. Its momentum (→p=m→v) is also directed along the same path. Let us fix an origin (O) at a perpendicular distance (d) from the path. At a particular instant, we can connect the particle which is at positon Q with a position vector (→r=−−→OQ).
Take, the angle between the →r and →p as θ. The magnitude of angular momentum of that particle at that instant is,
L=OQψnθ=OQmvsinθ=mv(OQsinθ)
The term (OQsinθ) is the perpendicular distance (d) between the origin and line along which the mass is moving. Hence, the angular momentum of the particle about the origin is,
L = mvd
The above expression for angular momentum L, does not have the angle θ. As the momentum (p = mv) and the perpendicular distance (d) are constants, the angular momentum of the particle is also constant. Hence, the angular momentum is associated with bodies with linear motion also. If the straight path of the particle passes through the origin, then the angular momentum is zero, which is also a constant.
Topper's Solved these Questions
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES BOOK - NCERT TAMILCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES EXERCISE - EXERCISE (I. MULTI CHOICE QUESTION)
15 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES BOOK - NCERT TAMILCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES EXERCISE - EXERCISE (V. NUMERICAL PROBLEMS)
6 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT TAMILCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ADDITIONAL EXERCISES
5 Videos
- NATURE OF PHYSICAL WORLD AND MEASUREMENTBOOK - NCERT TAMILCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - EXERCISE (V. Conceptual Questions)
5 Videos
Similar Questions
A particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is

View SolutionAssertion : If a particle moves with a constant velocity, then angular momentum of this particle about any point remains constant.
Reason : Angular momentum has the units of Plank's constant.
View Solution
Knowledge Check
The angular momentum of a moving body remains constant if
Anet external force is appliedBnet external torque is appliedCnet pressure is appliedDnet external torque is not applied .A particle of mass m is moving with constant velocity v parallel to the x-axis as shown in the figure. Its angular momentum about origin O is
 AmvbBmvaCmv√a2+b2Dmv(a+b)
AmvbBmvaCmv√a2+b2Dmv(a+b)A mass M moving with a constant velocity parlale to the X-axis. Its angular momentum with respect to the origin
Ais zeroBremains constantCgoes on increasingDgoes on decreasing
A particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?

View SolutionAssertion : If a particle moves with a constant velocity, then angular momentum of this particle about any point remains constant.
Reason : Angular momentum has the units of Plank's constant.
View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about P?
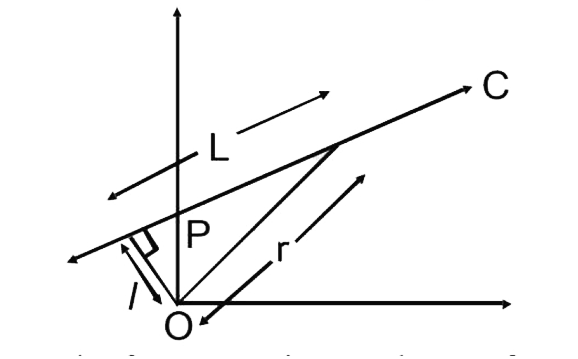
View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?

View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?

View Solution
