Repeat the previous problem if the particle C s displaced through a distance x along the line AB.
Net force
=14πε0⎡⎢
⎢
⎢⎣dθ((d2)+x)2−dθ((d2)−x)2⎤⎥
⎥
⎥⎦
=dθ4πε0⎡⎢
⎢
⎢
⎢
⎢⎣(d2)2+x2−xd−(d2)2−x2−xd[(d2)2−x2]2⎤⎥
⎥
⎥
⎥
⎥⎦
So,≠tforce=qθ4πε0−−2xdd4
=−qθ4πε0xd3
orm(2πT)2=qθ4πε0d3
T=[π3ε0md32θq]12.

Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
8 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMACHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Exercises
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
9 Videos
Similar Questions
Two particles A and B , each carrying charge Q are held fixed with a separation D between them. A particle C having mass m and charge q is kept at the middle point of the line AB. If particle C is displaced through a distance x along the line AB. What will be the time period of the oscillations.
View SolutionRepeat the previous problem in the following cases:

View Solution
Knowledge Check
In the previous problem, the maximum horizontal distance will be
A160√3mB140√3mC120√3mD100√3mIn the previous problem, the distance travelled by boat along the flow is
A50mB100mC150mD200m
Solve the previous problem , if particles moving in opposite direction.
View SolutionIn the previous problem, if the particle occupies a position x=7m at t=1s, then obtain an expression for the instantaneous displacement of the particle.
View SolutionIf in the previous problem, the particle travels 9/25 of the distance in its last second, find the time of fall and the height of the tower.
View SolutionIn the previous problem, the rod on which the ring slide is vertical Repeat the problem.
View SolutionSuppose the tube in the previous problem is kept verticla with B upward. Water enters through B at the rate of 1cm3s−1. Repeat parts a, b, and c. Note that the speed decreases as the water falls down.
View SolutionIn the previous problem, if tunnel is dug along a chord, find time period.
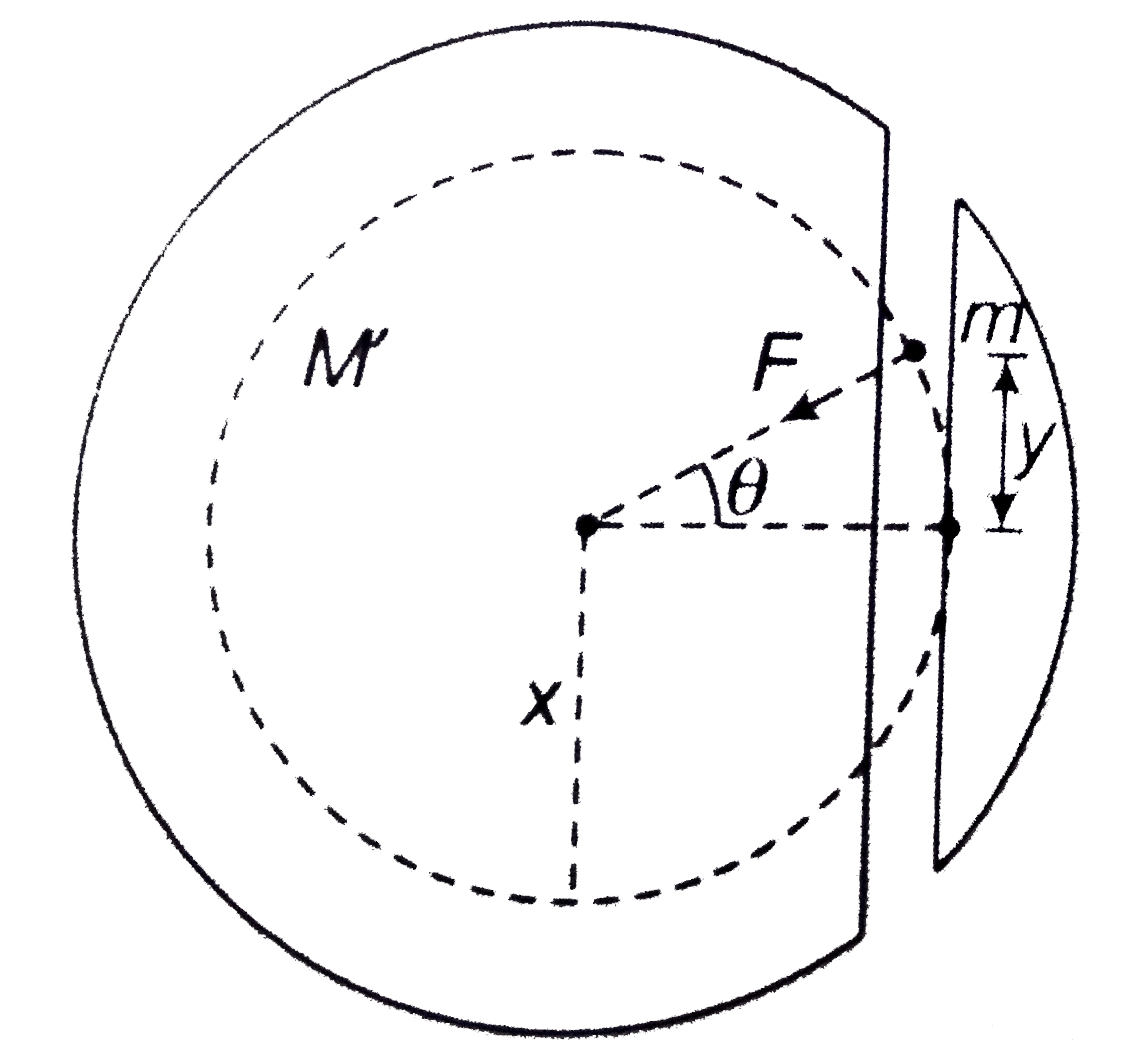
View Solution
