Electrons emitted with negligible speed from an electron gun are accelerated through a potential difference V along the x-axis. These electrons emerge from a narrow hole into a uniform magnetic field B directed along this axis. However. Some of the electrons emerging from the hole make slightly divergent angles as shown in . show that these paraxial electrons are refocussed on the x-axis at a distance √8π2mVeB2.

Give magnetic field = B,
Potential different = V, ltbr> Mass of electron = m,
Charge = q
Let electtric field be E'=Vr
v2=2×a×s [u = 0)<br>Timetakenbypartic≤→covertheareAsincethea∈isalong'y'aξs<br>ittravelalong−aξs∈uniformvelocity<br>TherformS = v xx t .
Topper's Solved these Questions
Similar Questions
Electrons emitted with negligible speed from an electron gun are accelerated through a potential difference V along X-axis.These electrons emerges from a narrow hole into a unifrom magnetic field B directed along the axis.However, some of the electrons emerging from the hole make slightly divergent angles as shown in figure.These paraxial electrons meet for second time on the X-axis at a distance √Nπ2mVeB2.Then find value of N.(Neglect interaction between electrons)
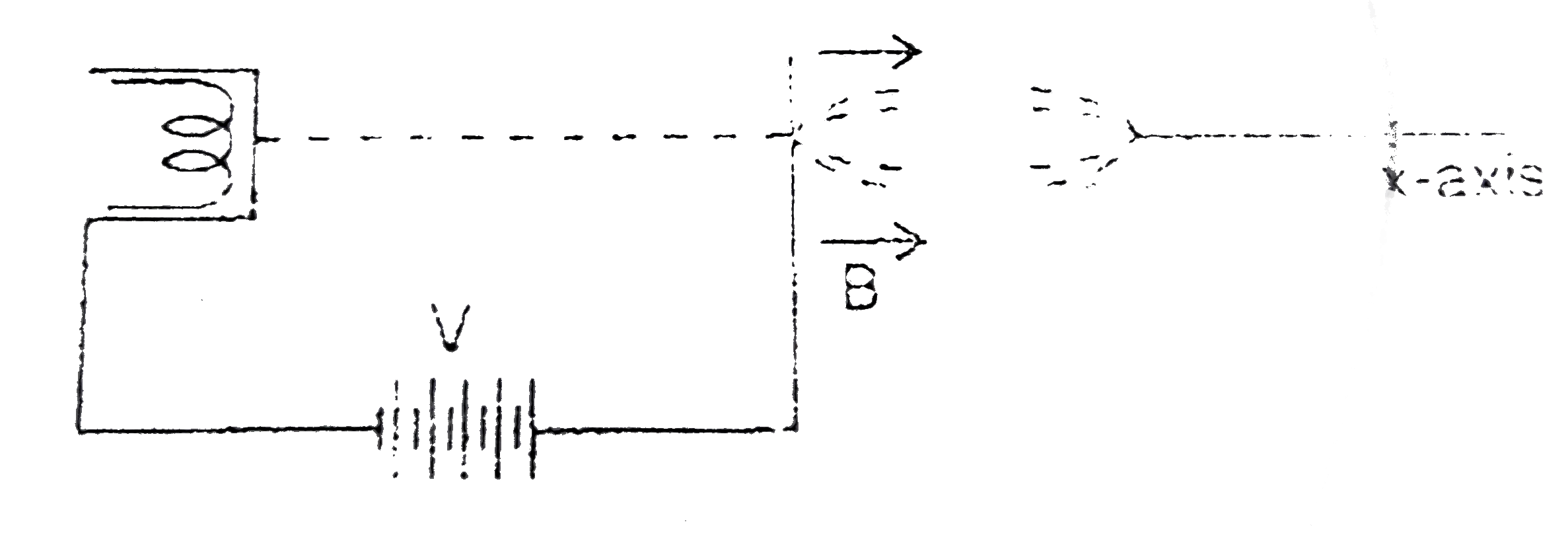
View SolutionIn a region of space, a uniform magnetic field B is along positive x-axis. Electrons are ernit.u.d from the origin with speed u at different, angles. Show that the paraxial electrons are refocused on the x-axis at a distance 2πmvBe. Here, m is the mass of electron and e the charge on it.
View Solution
Knowledge Check
When an electron is accelerated through a potential difference V, it experience a fromce F through as uniform transverse magnetic field. If the potential difference is increased to 2V, the force experoenced by the electron in the same magnetic field is
A2FB2√2FC√2FD4FAn electron is accelerated from rest through a potential difference V. This electron experiences a force F in a uniform magnetic field. On increasing the potential difference to V', the force experienced by the electron in the same magnetic field becomes 2F. Then, the ratio (V'/V) is equal to
A4/1B2/1C1/2D1/4An electron accelerated through a potential difference V passes through a uniform tranverses magnetic field and experiences a force F. If the accelerating potential is increased to 2V, the electron in the same magnetic field will experience a force:
AFBF/2C√2FD2F
In a region of space, a uniform magnetic field B is along positive x-axis. Electrons are ernit.u.d from the origin with speed u at different, angles. Show that the paraxial electrons are refocused on the x-axis at a distance 2πmvBe. Here, m is the mass of electron and e the charge on it.
View SolutionWhen an electron is accelerated through a potential difference V, it experience a force F through as uniform transverse magnetic field. If the potential difference is increased to 2V, the force experoenced by the electron in the same magnetic field is
View SolutionAn electron is accelerated from rest through a potential difference V. This electron experiences a force F in a uniform magnetic field. On increasing the potential difference to V', the force experienced by the electron in the same magnetic field becomes 2F. Then, the ratio (V'/V) is equal to
View SolutionAn electron accelerated through a potential difference V passes through a uniform tranverses magnetic field and experiences a force F. If the accelerating potential is increased to 2V, the electron in the same magnetic field will experience a force:
View SolutionAn electron accelerated through a potential difference V passes through a uniform transverse magnetic field and experiences a force F. If the accelerating potential is increased to 2V, the electron in the same magnetic field will experience a force.
View Solution
