Show a rectangular loop MNOP being pullled out of a magnetic field with a uniform velocity v by applying an external force F. The length MN is equal to l and the total resistance of the loop is R. Find(a) the current in the loop,(b) the magnetic force on the loop, (c) the external force F needed to magnetic the velocity, (d) the power delivered by the external force and (e) the thermal power developed in the loop.
 Solution in Gujrati
Solution in Gujrati
(a) The emf induced in the loop is due to the motion of the wire MN
The emf is varepsilon= vBl with the positive end at N and the negative and at M
The current is I=(ɛ)(R)=vBIR in the clockwise direction (b) The magnetic force on the wire MN is →F1=i→LX→B
The magnitude is F1=ilB=vB2l2R and is opposite to the velocity
the forces on the parts of the wire NO and PM, lying in the fijled, cancle each other
The resultant magnetic force on the loop is, therfore, F1=Bl^2vR opposite ot the velocity
(c)To move the loop at a constant velocity, the resultant force on it should be zero
Thus, one should pull the loop with a force F=F1=vB2l2R
(d) The power delivered by the external force is P=FV=v2B2l2R (e) The thermal power developed is P=l2R=(vBIR)2R=v2B2l2R
We see that the power delivered by the external force is equal to the thermal power developed in the loop
this is consistent with the principle of conservation of energy
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Worked Out Examples29videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Short Answer13videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises75videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises9videos
ELECTROMAGNETIC WAVES
Similar Questions
A conducting circular loop is placed is a uniform magnetic field B =0.20 T with its plane perpendicualr to the field . Somehow, the radius of the loop starts shrinking at a constant rate of 1.0mms−1. Find the induced emf in the loop at an instant when the radius is 2 cm.
04:25View SolutionA metallic loop is placed in a nonuiform steady magnetic field. Will an emf be induced in the loop?
View SolutionFind the dimensional formula of ε0 .
04:30View SolutionShow that the dimensions of the displacement current (ε0dΦEdt) are that of an electric current.
04:09View SolutionA current loop of arbitrary shape lien in a uniform magnetic field B. show that the net magnetic force acting on the loop is zero.
03:21View SolutionA triangular loop as shown in the figure is started to being pulled out at t=0 form a uniform magnetic field with a constant velocity v. Total resistancee of the loop is constant and equal to R. Then the variation of power produced in the loop with time will be :
06:33View SolutionA square shaped current loop of side length L and carrying current I lies in a uniform magnetic field B acting perpendicular to the plane of squre loop and directed inward. The net magnetic force acting on current loop is
05:09View SolutionA triangle loop as shown in the figure is started to being pulled out at t=0 from a uniform magnetic field with a constant velocity v.Total resistance of the loop is constant and equals to R.Then the variation of power produced in the loop with time will be:
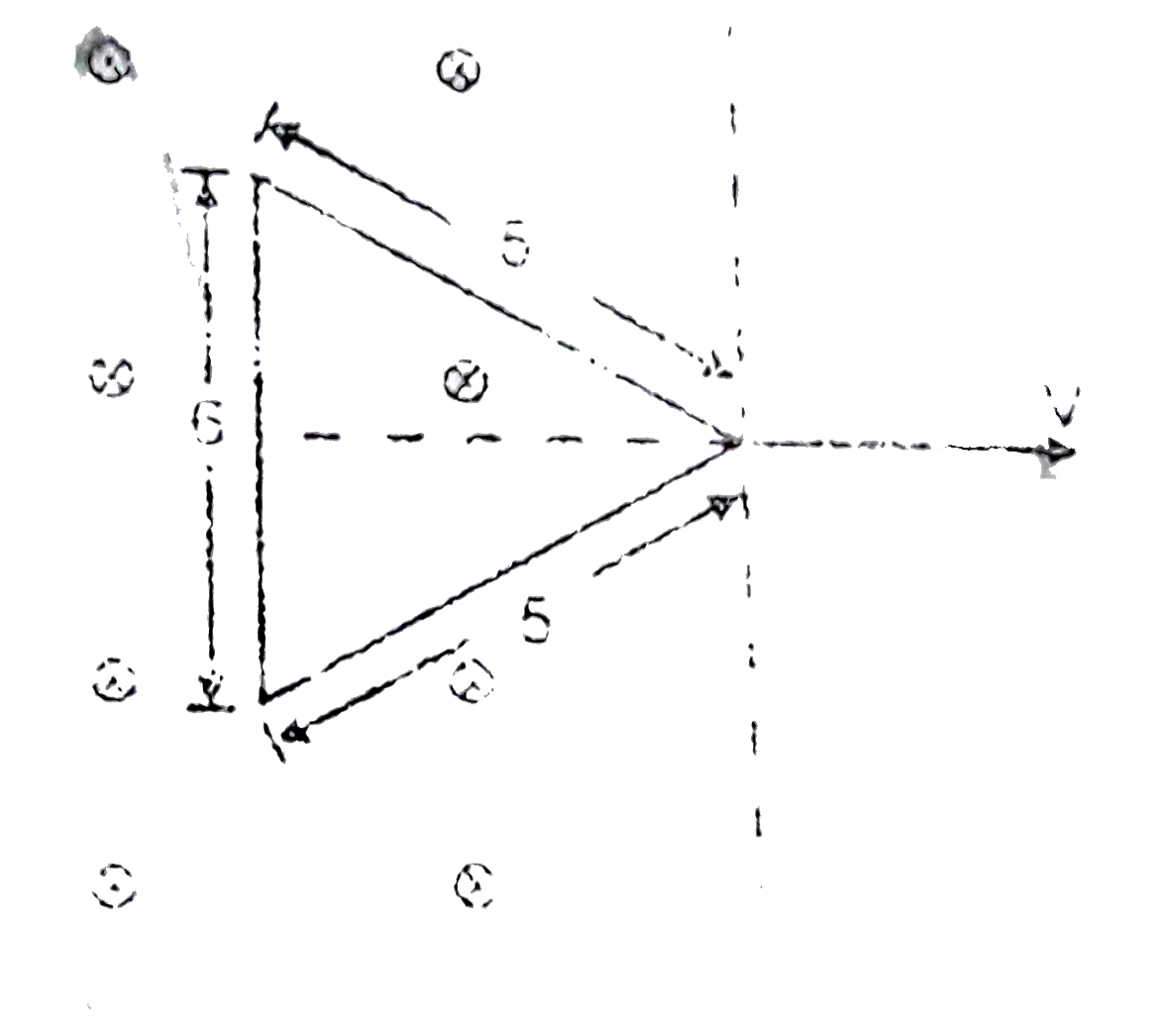 07:27View Solution
07:27View Solutionr त्रिज्या के एक चालक वृत्ताकार लूप में स्थायी धारा I बहती हैं। यह एकसामान चुंबकीय क्षेत्र →B लूप के तल के लंबवत हैं। लूप पर लगने वाला चुंबकीय बल हैं-
05:52View Solutionएक वर्गाकार धारावाही लूप एकसमान चुंबकीय क्षेत्र में इस प्रकार लटकाया जाता है कि चुंबकीय क्षेत्र लूप के तल में कार्य करे । यदि लूप की एक भुजा पर लगने वाले बल का मान →F हो तो शेष तीन भुजाओ पर नेट बल होगा -
03:59View Solutionएक आयताकार लूप और एक वृतीय लूप को एक नियत वेग से एकसमान चुम्बकीय क्षेत्र में वेग v से बाहर निकल रहे है । गति के दौरान किस लूप में प्रेरित वि .वा . बल नियत होगा ?
02:26View Solutionएकसमान चुम्बकीय क्षेत्र में लटके धारावाही लूप पर लगने वाला बल आघूर्ण अधिकतम होता है , जबकि लूप का तल होता है :
05:24View Solutionसामान क्षेत्रफल के एक आयताकार लूप तथा एक वृत्तकार लूप को नियत वेग से एकसमान चुंबकीय क्षेत्र से बाहर हटाया जाता है। यदि चुंबकीय क्षेत्र, लूप के तल के लंबवत हो, तो किस लूप में प्रेरित वि.वा. बल अधिक होगा ?
04:04View SolutionR प्रतिरोध वाला आयातकार लूप समचुंबकिय क्षेत्र B से दायी औरV वेग से खींचा जाता है लूप में प्रेरित धारा बनाए रखने के लिए आवश्यक बल F है
05:31View SolutionA current loop of arbitrary shape lies in a uniform magnetic field B. show that the net magnetic force acting on the loop is zero.
02:49View SolutionFig. Show a rectangular loop MNOP being pulled out of a magnetic field with a uniform velocity v by applying an external force F. The length MN is equal to l and the total resistance of the loop is R. Find(a) the current in the loop,(b) the magnetic force on the loop, (c) the external force F needed to maintain the velocity, (d) the power delivered by the external force and (e) the thermal power developed in the loop.
 04:30View Solution
04:30View Solution

