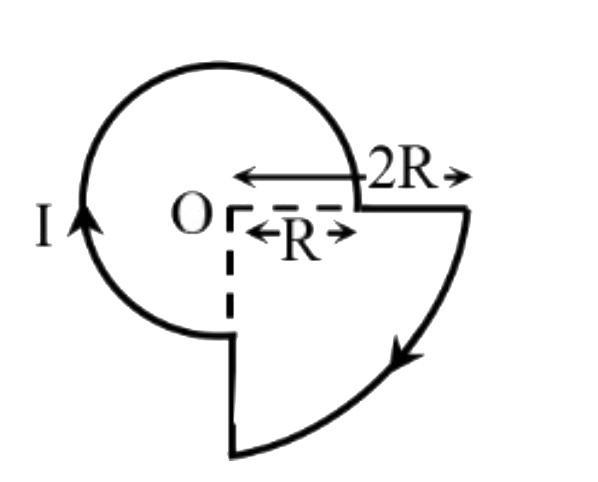
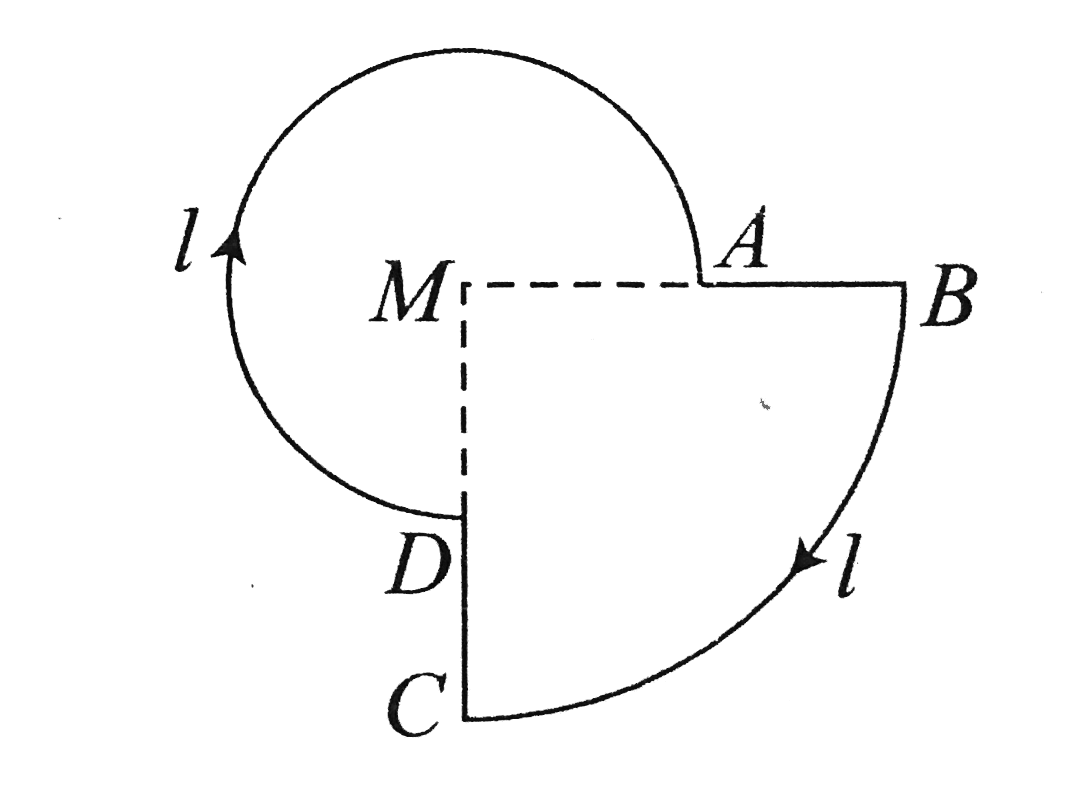
A current I is flowing through a loop. The direction of the current and the shape of the loop are as shown in figure. Let the magnitude magnetic field at the centre of the loop is found to be Q times μ0IR. What should be the value of Q?
(Here MA=R<MB=3R,∠DMA=90∘)
Video Solution
Text Solution
The correct Answer is:00.42
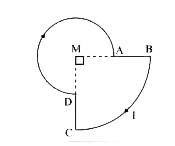
Magnetic field at the centre M due to current through the curved protion DA is
B1=μ0I4πR(3π2) inot the plane Magnetic field at the centre M due to current through the straight portion AB is B2=0.
Magnetic field at centre M due to current through the curved protion BC is
B3=μ0I4π(3R)×π2 into the plane Magnetic field at the centre M due to current through straight portion CD is
B4=0
The resultant magnetic field at M is
B=B1+B2+B3+B4
=3μ0I8R+0+μ0I24R+0=1024μ0IR
∴B=0.42μ0IR
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A current /is flowing through the loop. The direction of the current and the shape of the loop are as shown in the figure. The magnetic field at the centre of the loop is μ0IR×(MA=R,MB=2R,∠DMA=90∘
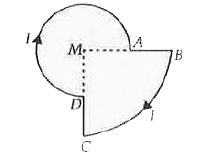 A516 ,but of the plane of the paper.B516, but into the plane of the paper.C716,but out of the plane of the paper.D716,but into the plane of the paper.
A516 ,but of the plane of the paper.B516, but into the plane of the paper.C716,but out of the plane of the paper.D716,but into the plane of the paper. - Question 2 - Select One
A current I is flowing thorugh the loop. The direction of the current and the shape of the loop are as the shown in the figure. The magnetic fild at the centre of the loop is μ0IR times
(MA=R,MB=2R,∠DMA=90∘)
 A516, but out of the plane of the paper.B716, but into of the plane of the paper.C516, but into of the plane of the paper.D716, but out of the plane of the paper.
A516, but out of the plane of the paper.B716, but into of the plane of the paper.C516, but into of the plane of the paper.D716, but out of the plane of the paper. - Question 3 - Select One
A square loop of side a carries a current I. The magnetic field at the center of the loop is
A2μ0I√2πaBμ0I√2πaC4μ0I√2πaDμ0Iπa
A current I flows through a closed loop as shown in figure. The magnetic field induction at the centre 0 is
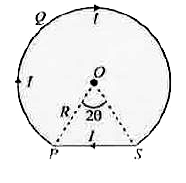 View Solution
View SolutionA current I flows through a closed loop as shown in figure .The magnetic field at the centre O is
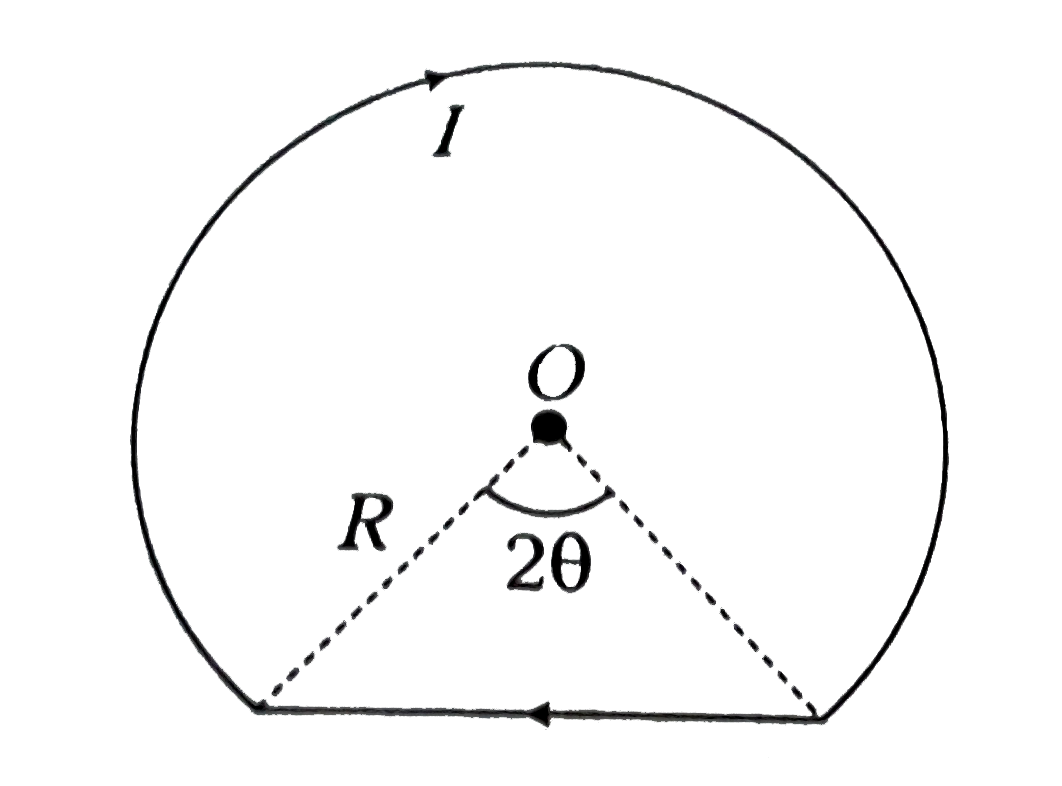 View Solution
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 View Solution
View SolutionEqual current I flows in two segments of a circular loop in the direction shown in figure. Radius of the loop is 7. The magnitude of magnetic field induction at the centre of the loop O is
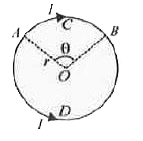 View Solution
View SolutionA rectangular loop of conductor of length a and breadtlı b carrying current / is shown in the figure. The magnetic field at the centre o of the loop is
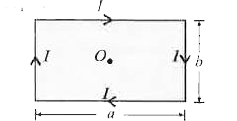 View Solution
View Solution