In fig., O is a point in the interior of a triangle ABC, OD ⊥ BC, OE ⊥ AC and OF ⊥ AB. Show that:- OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
To solve the problem, we need to prove the equation:
OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
where O is a point inside triangle ABC, and OD, OE, and OF are perpendiculars dropped from O to the sides BC, AC, and AB respectively.
1. Identify Right Triangles:
We have three right triangles formed by the perpendiculars:
- Triangle OAF (with right angle at F)
- Triangle OBD (with right angle at D)
- Triangle OEC (with right angle at E)
2. Apply Pythagorean Theorem:
For each of these triangles, we can apply the Pythagorean theorem.
- For triangle OAF:
OA2=AF2+OF2(1)
- For triangle OBD:
OB2=OD2+BD2(2)
- For triangle OEC:
OC2=OE2+CE2(3)
3. Rearranging the Equations:
We can rearrange these equations to express OF2, OD2, and OE2 in terms of the other sides:
- From equation (1):
OF2=OA2−AF2(4)
- From equation (2):
OD2=OB2−BD2(5)
- From equation (3):
OE2=OC2−CE2(6)
4. Substituting Back:
Now we substitute equations (4), (5), and (6) back into the original equation we want to prove:
OA2+OB2+OC2−(OB2−BD2)−(OC2−CE2)−(OA2−AF2)=AF2+BD2+CE2
5. Simplifying the Left Side:
Simplifying the left side:
OA2+OB2+OC2−OB2+BD2−OC2+CE2−OA2+AF2
This simplifies to:
AF2+BD2+CE2
6. Conclusion:
Thus, we have shown that:
OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
Hence, the statement is proved.
We have three right triangles formed by the perpendiculars:
- Triangle OAF (with right angle at F)
- Triangle OBD (with right angle at D)
- Triangle OEC (with right angle at E)
For each of these triangles, we can apply the Pythagorean theorem.
OA2=AF2+OF2(1)
- For triangle OBD:
OB2=OD2+BD2(2)
- For triangle OEC:
OC2=OE2+CE2(3)
We can rearrange these equations to express OF2, OD2, and OE2 in terms of the other sides:
- From equation (1):
OF2=OA2−AF2(4)
- From equation (2):
OD2=OB2−BD2(5)
- From equation (3):
OE2=OC2−CE2(6)
Now we substitute equations (4), (5), and (6) back into the original equation we want to prove:
OA2+OB2+OC2−(OB2−BD2)−(OC2−CE2)−(OA2−AF2)=AF2+BD2+CE2
Simplifying the left side:
OA2+OB2+OC2−OB2+BD2−OC2+CE2−OA2+AF2
This simplifies to:
AF2+BD2+CE2
Thus, we have shown that:
OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
Hence, the statement is proved.
Topper's Solved these Questions
- TRIANGLESBOOK - OSWAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - NCERT CORNER ( TEXTBOOK QUESTIONS) ( EXERCISE - 6.6 )
12 Videos
- TRIANGLESBOOK - OSWAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - NCERT EXEMPLAR ( EXERCISE - 6.1 )
12 Videos
- TRIANGLESBOOK - OSWAL PUBLICATIONCHAPTER - TRIANGLESEXERCISE - NCERT CORNER ( TEXTBOOK QUESTIONS) ( EXERCISE - 6.4 )
9 Videos
- SURFACE AREAS AND VOLUMES BOOK - OSWAL PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - BOARD CORNER (LONG ANSWER TYPE QUESTIONS)
9 Videos
Similar Questions
In figure, O is a point in the interior of a triangle ABC, OD⊥BC,OE⊥ACand OF⊥AB. Show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AW2+CD2+BF2

View SolutionIn the figure given below O is a point in the interior of a triangle ABC , OD ⊥ BC ,OE ⊥ AC and OF⊥AB . Show that
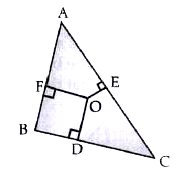
AF2+BD2+CE2=AE2+CD2+BF2
View Solution
Knowledge Check
What is the chemical formula of Ozone ?
A. O
B. O2
C. O3
D. O4ABBACDDC
O is any point in the plane of the triangle ABC,AO,BO and CO meet the sides BC,CA nd AB in D,E,F respectively show that ODAD+OEBE+OFCF=1.
View SolutionIn figure, ABC is triangle in which ∠ABC=90∘and AD⊥CBproduced. Prove that AC2=AB2+BC2−2BC.BD.

View SolutionIf B is an idempotent matrix, and A=I−B, then a. A2=A b. A2=I c. AB=O d. BA=O
View Solutionif D,E and F are the mid-points of the sides BC,CA and AB respectively of the ΔABCandO be any points, then prove that OA+OB+OC=OD+OE+OF
View SolutionIf △ABC is a right triangle such that ∠C=90∘ , ∠A=45∘ and BC=7 units. Find ∠B, AB and AC .
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
View SolutionIn an isosceles triangle ABC if AC=BC and AB2=2AC2 , then ∠C= 30o (b) 45o (c) 90o (d) 60o
View Solution