Consider a small mass m connected with a spring of constant K is constant K is executing SHM with amplitude A=1m. When it is has a phase of +π/3 from equilibrium position moving towards the right side, it receives an impulse that doubles its velocity without chaning its direction. The amplitude of the mass after the impulse is Bm. What is B=?(Take √3=3.61)(Approximate to two decimals)
The correct Answer is:01.80
T=2π√mk
v=ω√A2−y2
=2πT√A2−y2
Here y=A/2
⇒v=2πT√A2−(A/2)2=π√3AT
After impulse
v.=2v=2π√3AT
After impulse T,ω,m,k remains constant but amplitude will change
v.=2π√3AT=2πT√A21−y2
⇒√3A=√A21−y2
⇒3A2=A21−(A2)2
⇒A1=√132A=√132×1
∴A1=3.612m=1.802m
Topper's Solved these Questions
Similar Questions
Consider a horizontal spring-block system executing SHM. The block has a mass of 2 kg . When the block is at its equilibrium position, another object of mass 1 kg is put on it. Considering no slipping between the blocks, find the ratio of the new amplitude of the SHM? (Take √2=1.41,√3=1.73) (Approximate to two decimals)
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
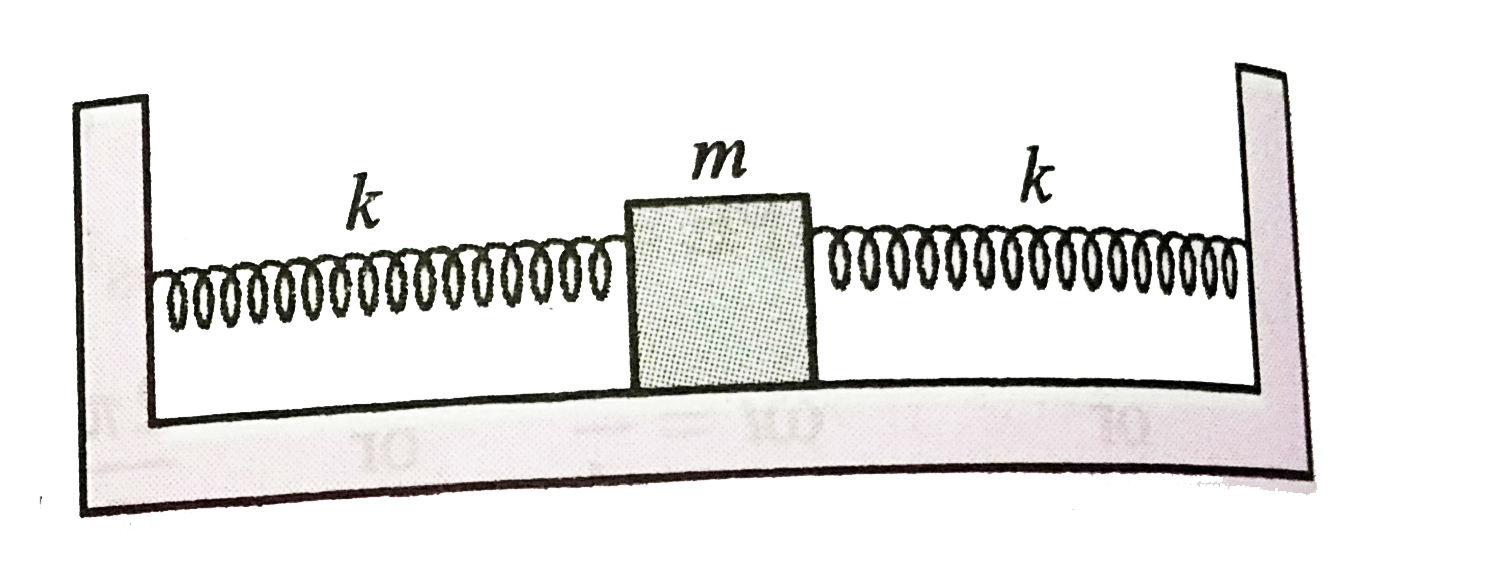
View Solution
Knowledge Check
A horizontal spring-block system of mass 1kg executes SHM of amplitude 10 cm. When the block is passing through its equilibrium position another mass of 1kg is put on it and the two move together.
Aamplitude will remain unchangedBamplitude will become 5√2 cmCthe frequency of oscillations will remain sameDthe frequency of oscillations will decrease.A mass (M), attached to a horizontal spring, executes S.H.M. whith amplitude A1.Whenthemass(M)passesthroghitsmeanpositionthenshal≤rmass(m)isplacedoveritand⊥hofthemmove→≥therwithamplitudeA_(2). The ration of.
A(a) M+mMB(b) M(M+m)12C(c ) (M+mM)12D(d) MM+mA particle exectues a linear S.H.M. of amplitude 2 cm. When it is at 1cm from the mean position, the magnitudes of its velocity and acceleration are equal. What is its maximum velocity ? [√3=1.732]
A2.5cm/sB1.4cm/sC3.464cm/sD4.322cm/s
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure . Show that when the mass is displaced from its equilibrium position on either side, it executes a simple harmonic motion. Find the period of oscillation.

View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shwon in figure . Show that when the mass is displaced from its equilibrium position on either side, it executes a simple harmonic motion. Find th eperiod of oscillation.

View SolutionA block of mass m is connected with two ideal pullies and a massless spring of spring constant K as shown in figure. The block is slightly displaced from its equilibrium position. If the time period of oscillation is μπ√mK. Then find the value of μ.

View SolutionA mass m, which is attached to a spring with spring constant k, oscillates on a horizontal table, with amplitude A. At an instant when the spring is streched by √3A/2, a second mass m is dropped vertically onto the original mass and immediately sticks to it. what is the amplitude of the resulting motion-
View SolutionIn the figure shown the spring constant is K the mass of the upper disc is m and that of the lower disc is 3m the upper block is depressed down from its equilibrium position by a distance δ=5mg/k and released at t=0 find the velocity of m when normal reaction on 3m is mg

View Solution
