A point source S is placed at the bottom of a 12 mm high transparent block of diamond ( refractive index = 2.4 ) . The block is immersed in an optically rarer liquid as shown in the figure. It is found that the light emerging from the block to the liquid forms a circular bright spot of diameter 18 mm on the top of the block. What is the refractive index of the liquid ?
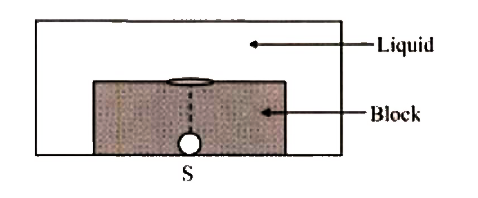
The correct Answer is:01.44
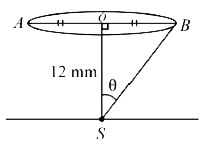
As the light is passing from optically denser medium to rarer medium. Let us assume θ is the critical angle.
From pythagoras theorem, SB=√SO2+OB2 =√122+92=15mm ∴sinθ=OBSB=915=35
sinθ=μ2μ1=μ2.4 ∴35=μ2.4⇒μ=1.44
Topper's Solved these Questions
Similar Questions
A jar 15cm long is filled with a transparent liquid. When viewed from the top, its bottom appears to be 12cm below. What is the refractive index of the liquid ?
View SolutionA point light source is attached to the base of an empty trough. A transparent liquid of refractive index 1.35 is filled in the trough to a height of 50 cm. Calculate the total area on the surface of the liquid through which light will emerge.
View Solution
Knowledge Check
A point source S is placed at the bottom of a tranparent block of height 10mm and refractive index 2.72. It is immersed in a lower refractive index liquid as shown in the figure. It is found that the light emerging from the block to the liquid forms a circular bright spot of diameter 11.54 mm on the top of the block. The refractive index of the liquid is
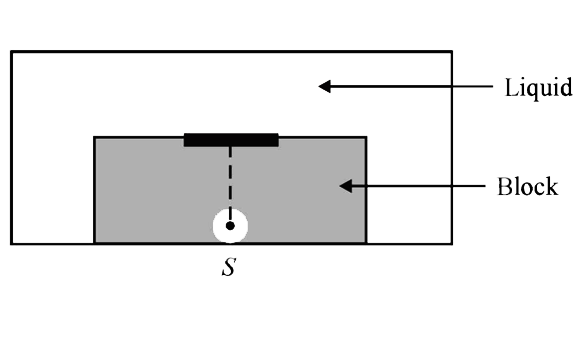 A1.21B1.3C1.36D1.42
A1.21B1.3C1.36D1.42A point source S is placed at the bottom of a transparent block of height 10mm and refractive index 2.72. It is immersed in a lower refractive index liquid as shwon in Fig. I tis found that the light emerging from the block to the liquid forms a circular bright spot of diameter 11.54mm on the top of the block. The refractive index of the liquid is
 A1.21B1.30C1.36D1.42
A1.21B1.30C1.36D1.42A block of mass 'm' is released on the top of a frictionless incline as shown in the figure. The time period of the oscillation of the block is
 A2π√mK+√2LsinθgB2π√mK+√2(L−l)gsinθC2π√(L−l)gsinθ+√mKDnone of these
A2π√mK+√2LsinθgB2π√mK+√2(L−l)gsinθC2π√(L−l)gsinθ+√mKDnone of these
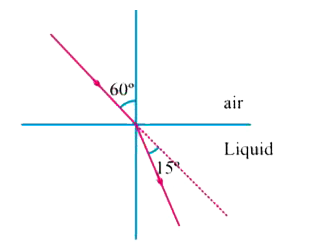
A light ray passes from air into a liquid as shown in figure. Find refractive index of liquid.
View SolutionWhen a ray of light emerges from a block of glass, the critical angle is
View SolutionWhen a ray of light emerges from a block of glass, the critical angle is
View SolutionA 10 kg block is connected to a 40 kg block as shown in the figure. The surface on that the blocks slide is frictionless. A force of 50 N pulls the blocks to the right.

What is the magnitude of the acceleration of the 40 kg block ?
View SolutionA 10 kg block is connected to a 40 kg block as shown in the figure. The surface on that the blocks slide is frictionless. A force of 50 N pulls the blocks to the right.

What is the magnitude of the tension T in the rope that connects the two blocks?
View Solution
