Gaussian surface should not pass through any discrete charge because:
Electric field remains constant
Electric field becomes zero
Electric field due to system of discrete charge is not will defined at location at any charge
Electric field is continuous charge distri bution
The correct Answer is:C
To answer the question of why a Gaussian surface should not pass through any discrete charge, we can break down the explanation into several steps:
1. Understanding Gaussian Surface:
A Gaussian surface is an imaginary closed surface used in Gauss's law to calculate electric fields. It is essential to choose the surface appropriately to simplify calculations.
2. Gauss's Law:
Gauss's law states that the electric flux through a closed surface is proportional to the charge enclosed within that surface. Mathematically, it is given by:
ΦE=Qencε0
where ΦE is the electric flux, Qenc is the total charge enclosed, and ε0 is the permittivity of free space.
3. Effect of Discrete Charges:
When a Gaussian surface passes through a discrete charge, it does not enclose the entire charge. This means that the total charge Qenc within the Gaussian surface is not well-defined.
4. Electric Field Calculation:
If the Gaussian surface intersects a charge, the electric field at the surface is not uniform. The electric field due to a point charge varies with distance, and thus, the flux calculation becomes complicated and inaccurate.
5. Flux Calculation Issues:
Since the electric field is not constant across the Gaussian surface when it intersects a charge, the total electric flux cannot be accurately calculated. This leads to difficulties in applying Gauss's law effectively.
6. Conclusion:
Therefore, to ensure that the electric field can be treated uniformly and that the total flux can be accurately calculated, a Gaussian surface should not pass through any discrete charge.
Final Answer:
A Gaussian surface should not pass through any discrete charge because it complicates the calculation of electric flux due to non-uniform electric fields and makes it difficult to apply Gauss's law effectively.
A Gaussian surface is an imaginary closed surface used in Gauss's law to calculate electric fields. It is essential to choose the surface appropriately to simplify calculations.
Gauss's law states that the electric flux through a closed surface is proportional to the charge enclosed within that surface. Mathematically, it is given by:
ΦE=Qencε0
where ΦE is the electric flux, Qenc is the total charge enclosed, and ε0 is the permittivity of free space.
When a Gaussian surface passes through a discrete charge, it does not enclose the entire charge. This means that the total charge Qenc within the Gaussian surface is not well-defined.
If the Gaussian surface intersects a charge, the electric field at the surface is not uniform. The electric field due to a point charge varies with distance, and thus, the flux calculation becomes complicated and inaccurate.
Since the electric field is not constant across the Gaussian surface when it intersects a charge, the total electric flux cannot be accurately calculated. This leads to difficulties in applying Gauss's law effectively.
Therefore, to ensure that the electric field can be treated uniformly and that the total flux can be accurately calculated, a Gaussian surface should not pass through any discrete charge.
A Gaussian surface should not pass through any discrete charge because it complicates the calculation of electric flux due to non-uniform electric fields and makes it difficult to apply Gauss's law effectively.
Topper's Solved these Questions
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - SECTION - B
24 Videos
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - SECTION - C
6 Videos
- SAMPLE PAPER 3BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3EXERCISE - SECNTION -C
6 Videos
- SAMPLE PAPER 6BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section-C
6 Videos
Similar Questions
Regarding a Gaussian surface mark the correct option (or) options. (A) must not pass through any discrete charge (B) must pass through any material medium (C) whether conducting or non- conducting may pass through a continuous charge distribution (D) may pass through a region where no material medium
View SolutionA spherical Gaussian surface surrounds a point charge q. If the charge is moved to another location inside the surface? Then total flux_____________
View Solution
Knowledge Check
Assertion: No net charge can exist in the region where electric field is uniform.
Reason: For any type of Gaussian surface selected within the region of a uniform electric field, the angle between electric field intensity and area normal is 90∘ everywhere. Hence, electric flux linked with the selected Gaussian surface is equal to zero. If the net electric flux is zero for some Gaussian surface then according to Gauss's law the net charge enclosed within the surface must be zero.AIf both assertion and reason are correct and reason is a correct explanation of the assertion .BIf both assertion and reason are correct but reason is not the correct explanation of assertionCIf assertion is correct but reason is incorrectDIf assertion is incorrect but reason is correct.Assertion(A):If Gaussian surface does not enclose any charge then →E at any point on the Gaussian surface must be zero.
Assertion(R ):No net charge is enclosed by Gaussians surface,so net flux passing through the surface is zero.ABoth A and R are true and R is the correct explanation of ABBoth A and R are True but R is not the correct explanation of A.CA is true and R is falseDA is false and R is true.In the situatiioni when the Gaussian surface is so chosen that there are some charges inside and some outside, then regarding the electric field at any point and the electric flux through the Gaussaina surface, which of the following is correct?
AThe field is due to the outside charges and flux depends on the inside chargesBThe field is due to all the charges and flux depends on the inside chargesCThe field is due to all the charges and flux depends on the outside chargesDThe field is due to the inside charges and flux depends on the outside charges
A solid insulating sphere of radius a carries a net positive charge 3Q, uniformly distributed throughout its volume. Concentric with this sphere is a conducting spherical shell with inner radius b and outer radius c and having a net charge -Q, as shown in figure

a. Consider a spherical Gaussian surface of radius rgtc, the net charge enclosed by this surface is .............
b. The direction of the electric field rgtc is .............
c. The electric field at rgtc is ............... .
d. The electric field in the region with radius r, which cgtrgtb, is ...............
e. Consider a spherical Gaussian surface of radius r, where c>r>b, the net charge enclosed by this surface is ................ .
f. Consider a spherical Gaussian surface of radius r, whereb>r>a, the net charge enclosed by this surface is ................. .
g. The electric field in the region b>r>a is ................ .
h. Consider a spherical Gaussian surface of radius r<a. Find an expression for the net charge Q(r) enclosed by this surface as a function of r. Note that the charge inside the surface is less than 3Q.
i. The electric field in the region r<a is ................. .
j. The charge on the inner surface of the conducting shell is .......... .
k. The charge on the outer surface of the conducting shell is .............. .
l. Make a plot of the magnitude of the electric field versus r.
View SolutionA point charge causes an electric flux of −1.0×103Nm2/C to pass through a spherical Gaussian surface of 10.0 cm radius centred on the charge. (a) If the radius of the Gaussian surface were doubled, how much flux would pass through the surface ? (b) What is the is the value of the point charge ?
View SolutionA spherical Gaussian surface encloses, and is concentric with, a charged spherical conductor. If the TNEI over the Gaussian surface is negative, what can you say about the electric field →E at a point on the surface?
View SolutionIf net charge enclosed by a Gaussian surface is zero, then
Assertion :- →E at any point on Gaussian surface is 0.
Reason :- No net charge is enclosed by Gaussian surface, so E = 0
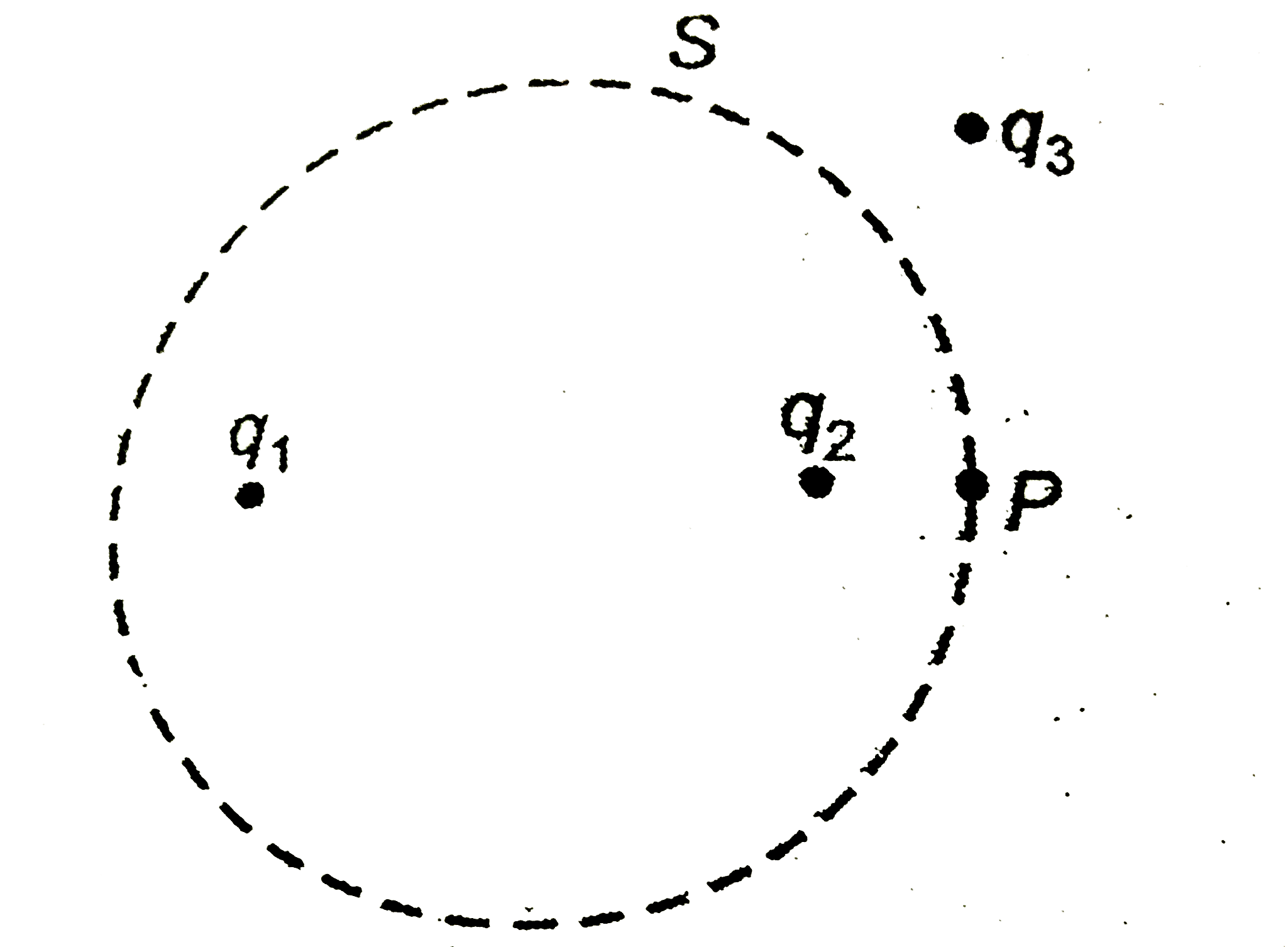
View SolutionThree point charges are placed as shown in the figure. S is a gaussian surface ϕ is the net flux passing through surface A and E be the electric field at point P on the surface, then
View Solution
