From the passage, we can conclude that:
New ventures are not risky.
New ventures are inherently risky.
Financial investment in a new venture is always non-profitable.
Conservative projection of companies' profits is suggested
The correct Answer is:B
New ventures are inherently risky.
Similar Questions
Displacement time graph of a particle moving in a straight line is as shown in figure.
From the graph we can conclude that work done on the block is :
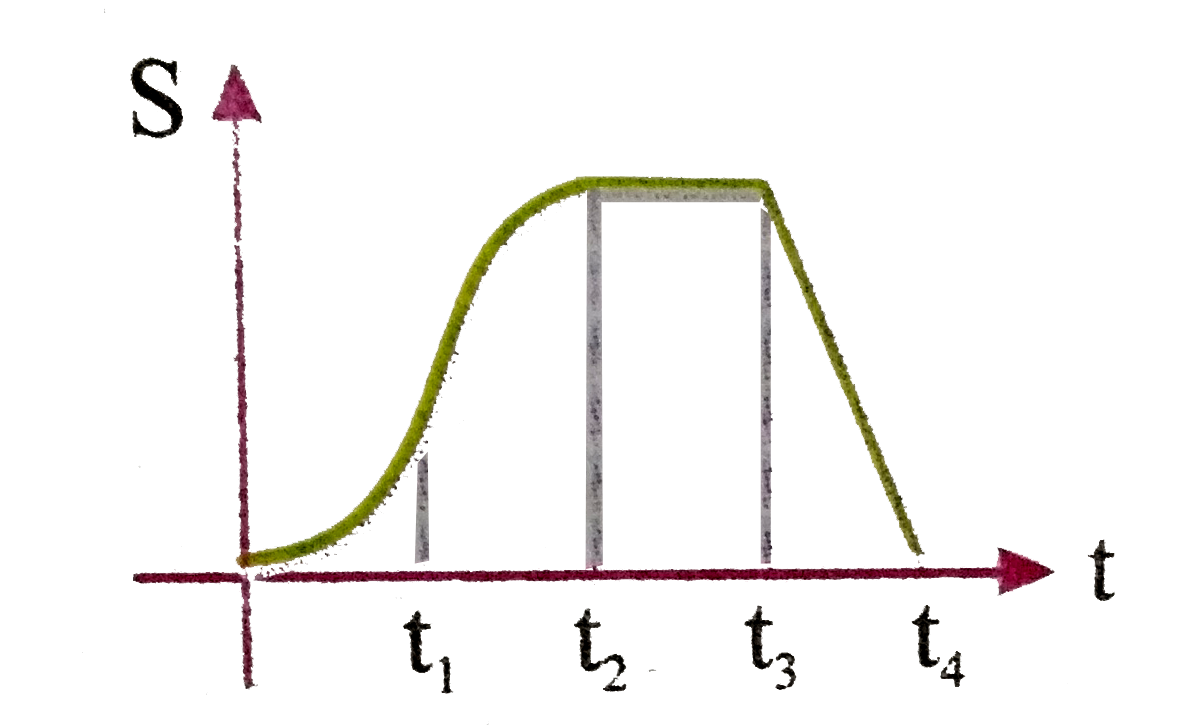 .View Solution
.View SolutionIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
View Solution
Knowledge Check
- Question 1 - Select One

Regarding the nature of the charge, we can conclude that :AThe charge is negativeBThe charge is positiveCThe particle has no chargeDNo conclusion can be made regarding nature of charge - Question 2 - Select One
The graph shows the variation of temperature (T) of one kilogram of a material with the heat (H) supplied to it. At O, the substance is in the solid state. From the graph, we can conclude that
 AT2 is the melting point of the solidBBC represent the change of state from solid to liquidC(H2−H1) represent the latest heat of fusion of the subsatanceD(H3−H1) represent the latest heat of vaporization of the liquid
AT2 is the melting point of the solidBBC represent the change of state from solid to liquidC(H2−H1) represent the latest heat of fusion of the subsatanceD(H3−H1) represent the latest heat of vaporization of the liquid - Question 3 - Select One or More
Displacement time graph of a particle moving in a straight line is as shown in figure.
From the graph we can conclude that work done on the block is :
 .Apositive from 0 to t1Bnegative from t1 to t2Czero from t2 to t3Dnegative from t3 to t4.
.Apositive from 0 to t1Bnegative from t1 to t2Czero from t2 to t3Dnegative from t3 to t4.
Tension in the cable supporting an elevator, is equal to the weight of the elevator. From this, we can conclude that the elevator is going up or down with a
View SolutionTension in the cable supporting an elevator, is equal to the weight of the elevator. From this, we can conclude that the elevator is going up or down with a
View SolutionFor a given surface the Gauss's law is stated as∮→E.d→A=0. From this we can conclude that
View SolutionFor a given surface the Gauss's law is stated as∮→E.d→A=0. From this we can conclude that
View SolutionIn a certain region of space, the electric field is zero. From this, we can conclude that the electric potential in this region is:
View Solution

