Calculate the packing efficiency of bcc structure.

A

0.88

B

0.78

C

0.68

D

0.58

Text Solution

Verified by Experts

The correct Answer is:C

Efficiency of packing in bcc structure : In this case the atom at the centre is in touch with other two atoms which are diagonally arranged. The sphere along the body diagonal are shown with solid boundaries.
In Delta EFD, $b^{2} = a^{2} + a^{2} = 2 a^{2}$
$b = \sqrt{2 a}$

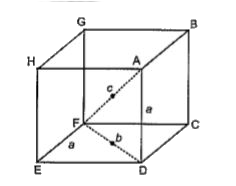
In $Δ A F D, c^{2} = a^{2} + b^{2} = a^{2} + 2 a^{2}$
$= 3 a^{2}$
$c = \sqrt{3 a}$
The length of the body diagonal a is equal to 4r. r being the radius of the sphere (atom). As all the three spheres along the diagonal touch each other.
$a = 4 r$
$∴ a = 4 r = \sqrt{3 a}$
$a = \frac{4 r}{\sqrt{3}} or r = \frac{\sqrt{3}}{4} a$
As already calculated the total number of atoms associated with a bcc unit cell is 2, the volume (v) is therefore
$2 \times \frac{4}{3} π r^{3} = \frac{8}{3} π r^{3}$
Volume of the unit cell $(V) = a^{3}$
$= {(\frac{4 r}{\sqrt{3}})}^{3} = \frac{64 r^{3}}{3 \sqrt{3}}$
Packing efficiency = $\frac{v}{V} = \times 100$
$= \frac{(\frac{8}{3}) π r^{3}}{(\frac{64}{3} \sqrt{3}) \times r^{3}} \times 100 = \frac{\sqrt{3}}{8}$
Therefore, 68% of unit cell is occupied by atoms and the rest 32% is empty space in bcc structure.

|

Updated on:21/07/2023

Class 12 CHEMISTRY SOLID STATE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The packing efficiency in hcp and ccp structures is
A $68 %$
B $74 %$
C $78 %$
D $64 %$

ICSE-THE SOLID STATE-MULTIPLE CHOICE QUESTIONS (Numerical based questions)

Home
Ask Doubt
Profile