A charge Q is placed at the centre of the line joining two charges q and q . The system of the three charges will be in equilibrium if Q is :
+q3
−q3
+q4
−q4
To solve the problem of determining the value of charge Q that will allow the system of three charges Q, q, and q to be in equilibrium, we can follow these steps:
1. Understanding the Configuration:
- We have two identical charges q placed at a distance 2a apart.
- The charge Q is placed at the midpoint of the line joining the two charges q.
2. Identifying Forces:
- The force on charge q due to charge Q is denoted as F1.
- The force on charge q due to the other charge q is denoted as F2.
3. Calculating Forces:
- The force F1 (the force exerted by Q on q) can be calculated using Coulomb's law:
F1=k|Q⋅q|a2
- The force F2 (the force exerted by the other q on q) is:
F2=kq2(2a)2=kq24a2
4. Setting Up the Equilibrium Condition:
- For the system to be in equilibrium, the net force acting on charge q must be zero:
F1−F2=0
- This implies:
F1=F2
5. Substituting the Forces:
- Substituting the expressions for F1 and F2:
k|Q⋅q|a2=kq24a2
6. Simplifying the Equation:
- Canceling k and a2 from both sides:
|Q⋅q|=q24
- This leads to:
|Q|=q4
7. Considering the Sign:
- Since the charge Q must be opposite in sign to the charges q for equilibrium (to attract), we conclude:
Q=−q4
Final Answer:
Thus, the charge Q must be:
Q=−q4
- We have two identical charges q placed at a distance 2a apart.
- The charge Q is placed at the midpoint of the line joining the two charges q.
- The force on charge q due to charge Q is denoted as F1.
- The force on charge q due to the other charge q is denoted as F2.
- The force F1 (the force exerted by Q on q) can be calculated using Coulomb's law:
F1=k|Q⋅q|a2
- The force F2 (the force exerted by the other q on q) is:
F2=kq2(2a)2=kq24a2
- For the system to be in equilibrium, the net force acting on charge q must be zero:
F1−F2=0
- This implies:
F1=F2
- Substituting the expressions for F1 and F2:
k|Q⋅q|a2=kq24a2
- Canceling k and a2 from both sides:
|Q⋅q|=q24
- This leads to:
|Q|=q4
- Since the charge Q must be opposite in sign to the charges q for equilibrium (to attract), we conclude:
Q=−q4
Thus, the charge Q must be:
Q=−q4
Topper's Solved these Questions
- QUESTION PAPER 2022 TERM 1 SET 2BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 2EXERCISE - SECTION B
24 Videos
- QUESTION PAPER 2022 TERM 1 SET 2BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 2EXERCISE - SECTION C
6 Videos
- QUESTION PAPER 2022 TERM 1 SET 1BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2022 TERM 1 SET 1EXERCISE - SECTION-C
6 Videos
- QUESTION PAPER 2023BOOK - XII BOARDS PREVIOUS YEARCHAPTER - QUESTION PAPER 2023EXERCISE - Question
43 Videos
Similar Questions
A charge q is placed at the centre of the line joining two equal charges Q. Show that the system of three charges will be in equilibrium if q=−Q4.
View SolutionA charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
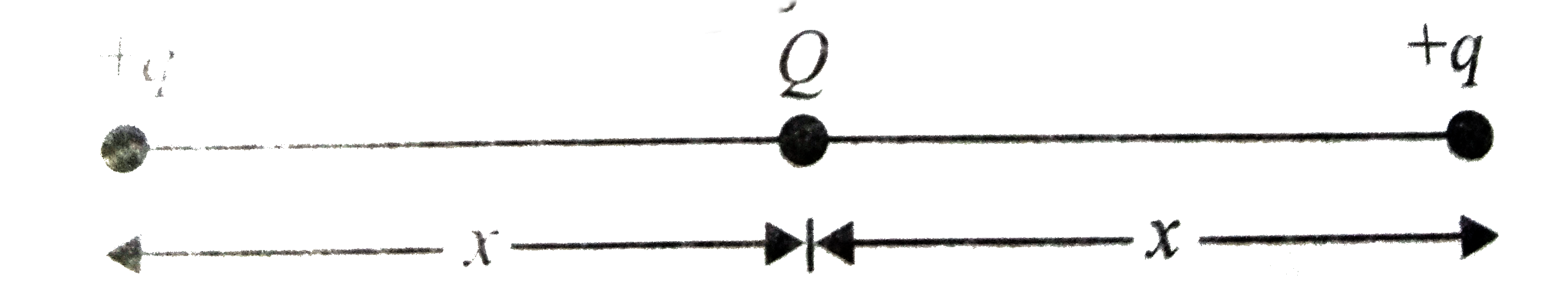
View Solution
Knowledge Check
A charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium , if q is equal to
A−Q2B−Q4C+Q4D+Q2A charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
A(a) −Q2B(b) −Q4C(c) +Q4D(d) +Q2A charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
AQ4B−Q4CQD−Q8
A point charge q is placed at the centre of a line joining two equal charges Q. The whole system is in the state of equilibrium. Can you impose some lower limit on the magnitude of the charge Q?
View SolutionA charge q is placed in the middle of a line joining the two equal and like point charge Q .This charge q will remain in equilibrium if q=Qx .What is x?
View SolutionA charge "q" is placed at the centroid of an equilateral triangle. Three charges having same "],[" charge equal to "Q" are placed at the vertices of the triangle.The system of four charges will be in equilibrium if "q" is equal to
View SolutionTwo equal charges, +Q each, are at a distance r from each other. A third charge is placed on the line joining the above two charges such that all these charges are in equilibrium. Find q, in terms of Q.
View SolutionA charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View Solution
