There are 20 students in a class. The mean value of their scores is 135. On rechecking, two mistakes were found . After correction, the marks of one student was increased by 35 and the marks of the other was decreased by 15.What is the mean value of the marks after correction?
135.5
136
155
134.5
To find the new mean value of the marks after the corrections, we can follow these steps:
Step 1: Calculate the initial total score of the students.
The mean score of the students is given as 135, and there are 20 students.
Total Score=Mean×Number of Students=135×20
Calculating this gives:
Total Score=2700
Step 2: Adjust the total score based on the corrections.
One student's score was increased by 35, and another student's score was decreased by 15.
The net change in the total score can be calculated as:
Net Change=+35−15=+20
Now, we can find the new total score:
New Total Score=Old Total Score+Net Change=2700+20=2720
Step 3: Calculate the new mean.
To find the new mean, we divide the new total score by the number of students:
New Mean=New Total ScoreNumber of Students=272020
Calculating this gives:
New Mean=136
Conclusion
The mean value of the marks after correction is 136.
---
The mean score of the students is given as 135, and there are 20 students.
One student's score was increased by 35, and another student's score was decreased by 15.
To find the new mean, we divide the new total score by the number of students:
The mean value of the marks after correction is 136.
Similar Questions
The mean marks obtained by 300 students in a subject are 60. The mean of top 100 students was found to be 80 and the mean of last 100 students was found to be 50. The mean marks of the remaining 100 students are
View SolutionThe average marks of 18 students in an examination was 60. It was later found that the marks of one student had been wrongly entered as 63 instead of 36. The correct average is:
View Solution
Knowledge Check
The table shows the marks obtained by 25 students in a class test. Find the mean of the marks obtained
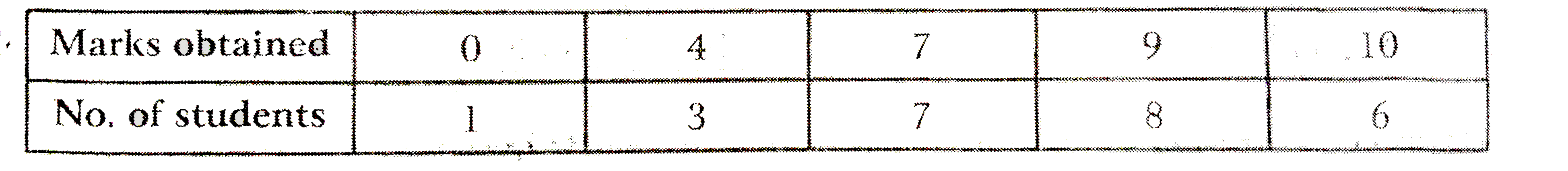 A7.72B7.65C7.4D7
A7.72B7.65C7.4D7The average marks of 30 students are 45 but after checking , there are two mistakes found. After adjustment , if a student got 45 more marks and other student got 15 less marks , then what will be the adjusted average ?
A45B44C47D46Two students appeared for an examination. One student who scored 20 marks more than the other, his score was 60% of the sum of their marks. What was his score?
A80B20C40D60
The average marks of 50 students in an examination was 65. It was later found that the marks of one student had been wrongly entered as 83 instead of 38. The correct average is?
View SolutionThe mean of the marks scored by 40 students was found to be 35. Later on it was discovered that a score of 43 was misread as 34. The correct mean is
View Solution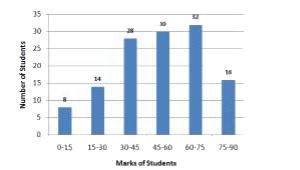
In the given histogram, what is the mean marks of the students. correct to one decimal place?
View Solution
In the given histogram, what is the mean marks of the students. correct to one decimal place?
View SolutionThe average marks of 42 students in an examination, was 64. Later it was found that the marks of three students were misread as 72, 58 and 44 instead of the actual marks 64, 42 and 26 respectively. What is the correct average marks?
View Solution
