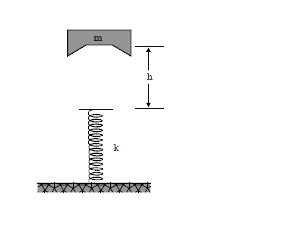
A block of mass 2kg initially at rest is dropped from a height of 1m into a vertical spring having force constant $490 N / m^{- 1}$ . Calculate the maximum distance through which the spring will be compressed?

Text Solution

Verified by Experts

$m = 2 k g, h = 1 m, k = 490 N m^{- 1}$
k is the spring constant.
Let the spring be compressed through a distance x. Then the block falls through a height h= x.
Gain in P.E. of spring = Loss in P.E. of block.
$\frac{1}{2} k x^{2} = m g (h + x)$
$\frac{1}{2} \times 490 \times x^{2} = 2 \times 9.8 \times (1 + x)$
$\frac{245 x^{2}}{19.6} = 1 + x$
$12.5 x^{2} - x - 1 = 0$
$x = \frac{1 \pm \sqrt{1 + 4 \times 12.5}}{2 \times 12.5} = \frac{1 \pm \sqrt{51}}{25}$
$= 0.3256 m$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A block of mass $2 k g$ is propped from a height of $40 c m$ on a spring where force constant is $1960 N m^{- 1}$ The maximum distance thought which the spring compressed by
A $5 c m$
B $15 c m$
C $26 c m$
D $10 c m$
Question 2 - Select One
A block of mass $2 k g$ is propped from a heught of $40 c m$ on a spring where force constant is $1960 N m^{- 1}$ The maximum distance thought which the spring compressed by
A5 cm
B15 cm
C20 cm
D10 cm
Question 3 - Select One

A block of mass m initially at rest is dropped from a height h on to a spring of force constant k . the maximum compression in the spring is x then
A $m g h = \frac{1}{2} k x^{2}$
B $m g (h + x) = \frac{1}{2} k x^{2}$
C $m g h = \frac{1}{2} k {(x + h)}^{2}$
D $m g (h + x) = \frac{1}{2} k {(x + h)}^{2}$

ICSE-DYNAMICS-ADDITIONAL SOLVED PROBLEMS

Home
Ask Doubt
Profile