Check whether the following vectors are orthogonal.
(i) →A=2ˆi+3ˆj and →B=4ˆi−5ˆj
(ii) →C=5ˆi+2ˆj and →D=2ˆi−5ˆj
Text Solution
→A.→B=8−15=−7≠0
Hence →A and →B are not orthogonal to each other.
→C.→D=10−10=0
Hence, →C and →D are orthogonal to each other.
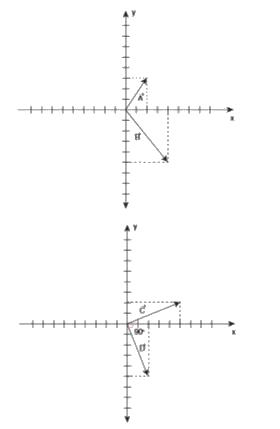
It is also possible to geometrically show that the vectors →C and →D are orthogonal to each other. Th is is shown in the following Figure.
In physics, the work done by a force →F to move an object through a small displacement →dr is defined as,
W=→F.d→r
W=Fdrcosθ
Th e work done is basically a scalar product between the force vector and the displacement vector. Apart from work done, there are other physical quantities which are also defi ned through scalar products.
|
Topper's Solved these Questions
- KINEMATICSBOOK - NCERT TAMILCHAPTER - KINEMATICSEXERCISE - EXERCISE (I. Multi Choice Question)
15 Videos
- KINEMATICSBOOK - NCERT TAMILCHAPTER - KINEMATICSEXERCISE - EXERCISE (IV. Exercises )
20 Videos
- HEAT AND THERMODYNAMICSBOOK - NCERT TAMILCHAPTER - HEAT AND THERMODYNAMICSEXERCISE - EVALUATION (Numerical Problems)
13 Videos
- KINETIC THEORYBOOK - NCERT TAMILCHAPTER - KINETIC THEORYEXERCISE - ADDITIONAL EXERCISE
4 Videos
Similar Questions
Knowledge Check
The three vectors →A=3ˆi−2ˆj+ˆk,→B=ˆi−3ˆj+5ˆk and →C=2ˆi+ˆj−4ˆk form
AAn equilateral triangleBIsosceles triangleCA right angled triangleDNo triangleGiven : →A=2ˆi+3ˆj and →B=5ˆi−6ˆj. The magnitude of →A+→B is
A4 unitsB10 unitsC√58 unitsD√61 unitsSelect CORRECT statement(s) for three vectors →a=−3ˆi+2ˆj−ˆk,→b=ˆi−3ˆj+5ˆk and →c=2ˆi+ˆj−4ˆk
AThe above vectors acna form triangleBComponent of →a along →c is 3C→a makes angle cos−1√27 with y-axis.DA vector having magnitude twice the vector →a and anti parallel to vector →b is √25(−2ˆi+6ˆj−10ˆk)
If →a=(ˆi−2ˆj),→b=(2ˆi−3ˆj) and →c=(2ˆi+3ˆk), find (→a+→b+→c).
View Solution(i) Find the sum of vectors →a=ˆi−2ˆj+ˆk,→b=−2ˆi+4ˆj+5ˆk, and →c=ˆi−ˆj−7ˆk (ii) Find the magnitude of the vector →a=3ˆi−2ˆj+6ˆk.
View SolutionSelect CORRECT statement(s) for three vectors →a=−3ˆi+2ˆj−ˆk,→b=ˆi−3ˆj+5ˆk and →c=2ˆi+ˆj−4ˆk
View SolutionIf →a=2ˆi+3ˆj+ˆk,→b=ˆi−2ˆj+ˆk and →c=−3ˆi+ˆj+2ˆk, then [→a→b→c]=
View SolutionIf →a=2ˆi+3ˆj+ˆk,→b=ˆi−2ˆj+ˆk and →c=−3ˆi+ˆj+2ˆk, then [→a→b→c]=
View Solution
