Three identical vessels A, B and C contain same quantity of liquid. In each vessel, blocks with different densities but same masses are placed as shown in figure. If FA,FBandFC are the total forces acting on the base of vessels A, B and C respectively, then
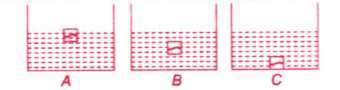
FA=FB=FC
FA<FB<FC
FA=FB<FC
FA>FB>FC
The correct Answer is:A
Force acting on the base of vessels A, B and C is the weight of block and weight of water, which is same for all the three vessels. Hence, FA=FB=FC.
Topper's Solved these Questions
Similar Questions
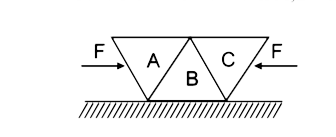
Three triangular blocks A,B and C of equal masses ‘m’ are arranged as shown in figure. Draw F.B.D. of blocks A,B and C. Indicate action– reaction pair between A,B and B,C.
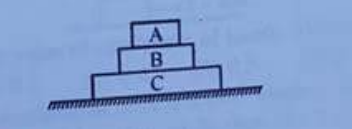
View SolutionThree blocks are placed as shown in figure. Mass of A, B and C are m1,m2,and m3respectively. The force exerted by block 'C' on 'B' is :-
View Solution
Knowledge Check
Three identical vessels A,B and C contain same quantity of liquid. In each vessel balls of different densities but same masses are placed. In vessel A, the ball is partly submerged, in vessel B, the ball is completely submerged but floating and in vessel C, the ball has sunk to the base. If FA,FB and FC are the total forces acting on the base of vessels A,B and C, respectively, then
 AFA=FB=FCBFA<FB<FCCFA=FB<FCDFA<FB<FC
AFA=FB=FCBFA<FB<FCCFA=FB<FCDFA<FB<FCThree vessels A, B and C of different shapes contain water upto the same height in the figure. PA,PBandPC be the pressure exerted by the water at the bottom of the vessels A, B and C respectively. Then
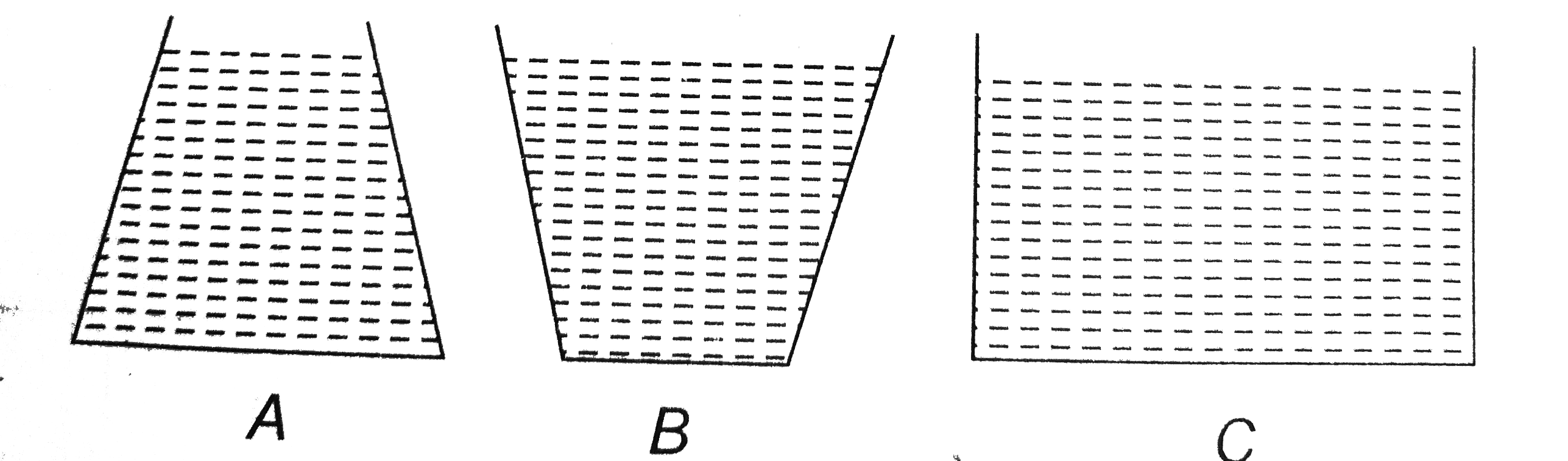 APA>PB>PCBPB>PC>PACPC>PB>PADPA=PB=PC
APA>PB>PCBPB>PC>PACPC>PB>PADPA=PB=PCThree vessels A,B,and C of different shapes contain a water upto the same height as shown in the figure .PA,PB ,and PC be the pressure exerted by the water at the bottom of the vessels A ,B and C respectively . Then
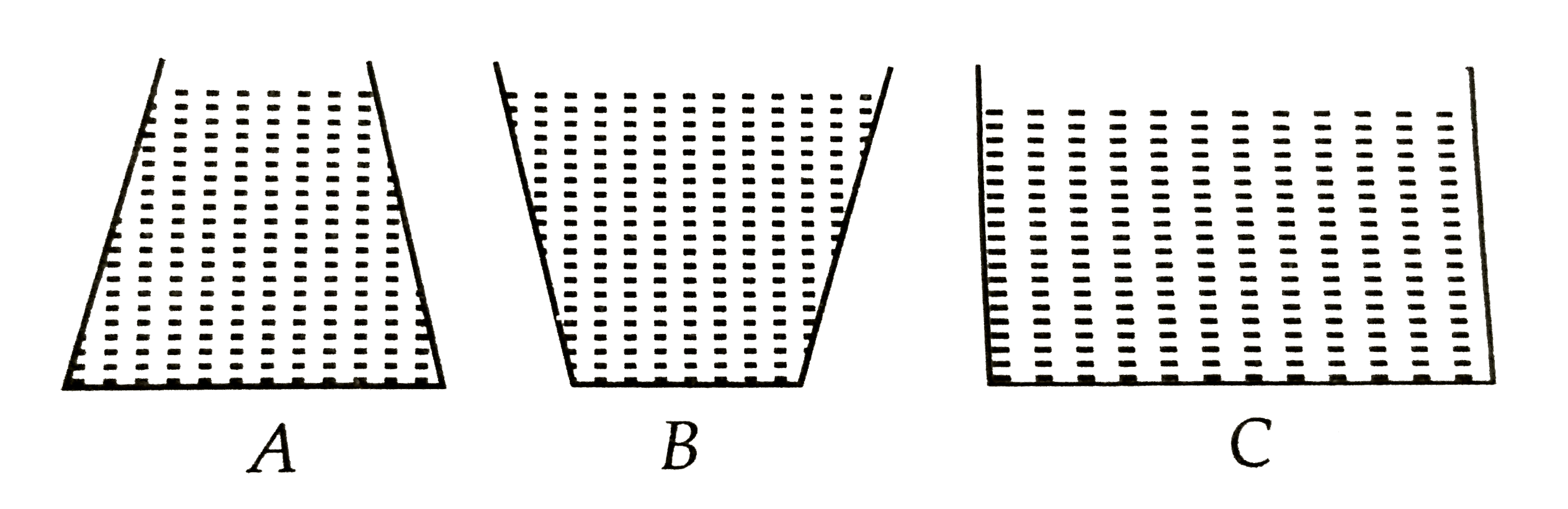 APA>PB>PCBPB>PC>PACPC>PB>PADPA=PB=PC
APA>PB>PCBPB>PC>PACPC>PB>PADPA=PB=PC
Three containers of same base area, same height are filled with three different liquids of same mass as shown in the figure. If F1,F2,F3 are the force exerted by the liquid on the base of the container in case I, and II respectively, then we have the relation:

View SolutionTwo ice cubes of side 10cm, having cavity of volume 20cm3 at centre of cube but filled with different materials A and B respectively. The specific gravity of material B is 1.9 Now these cubes are placed in two different vessels of same base area as shown in figure. The water level before putting blocks in vessels are same Assume that ice melts uniformly from all sides and with same constant rate in both the vessels. (specific gravity of ice=0.9)
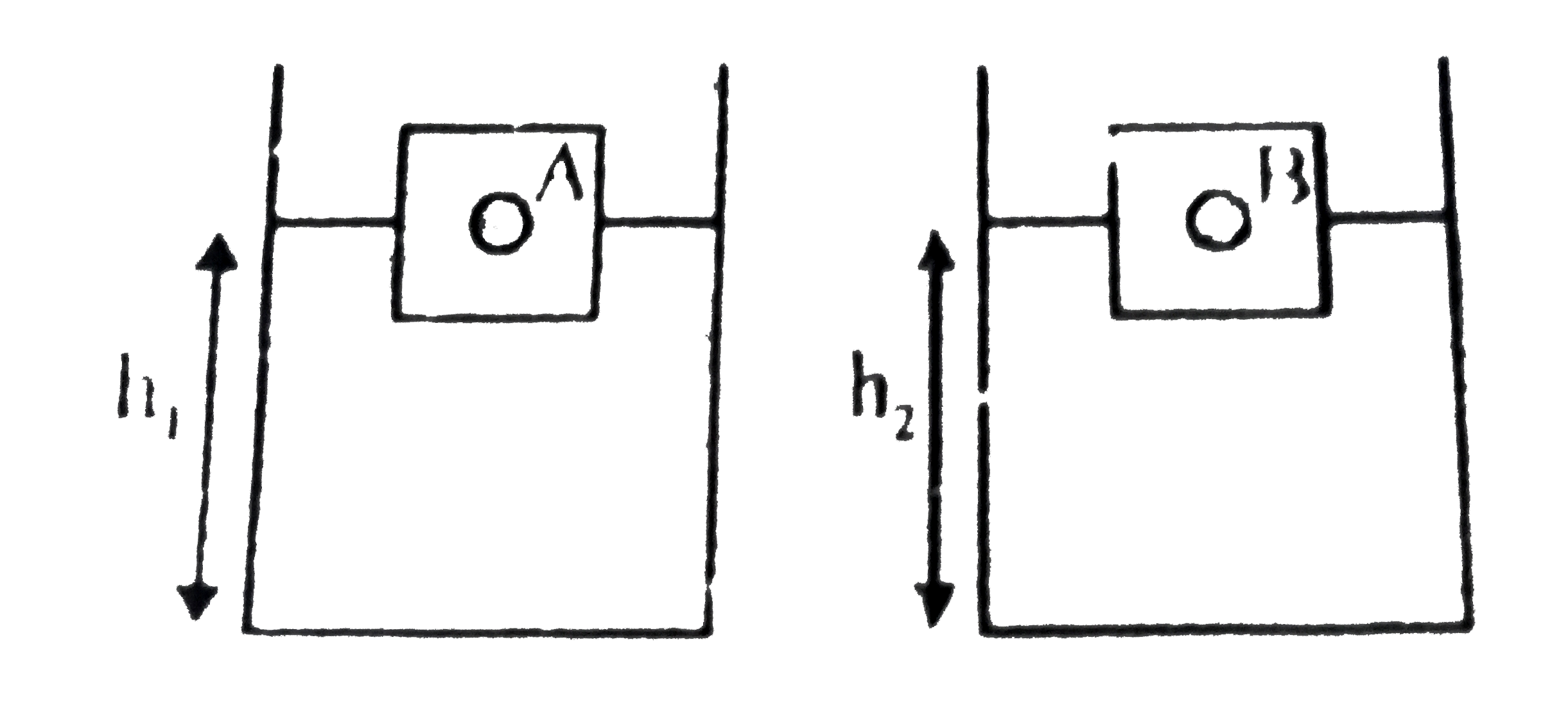 Choose the correct statement:
Choose the correct statement:
View SolutionTwo ice cubes of side 10cm, having cavity of volume 20cm3 at centre of cube but filled with different materials A and B respectively. The specific gravity of material B is 1.9 Now these cubes are placed in two different vessels of same base area as shown in figure. The water level before putting blocks in vessels are same Assume that ice melts uniformly from all sides and with same constant rate in both the vessels. (specific gravity of ice=0.9)
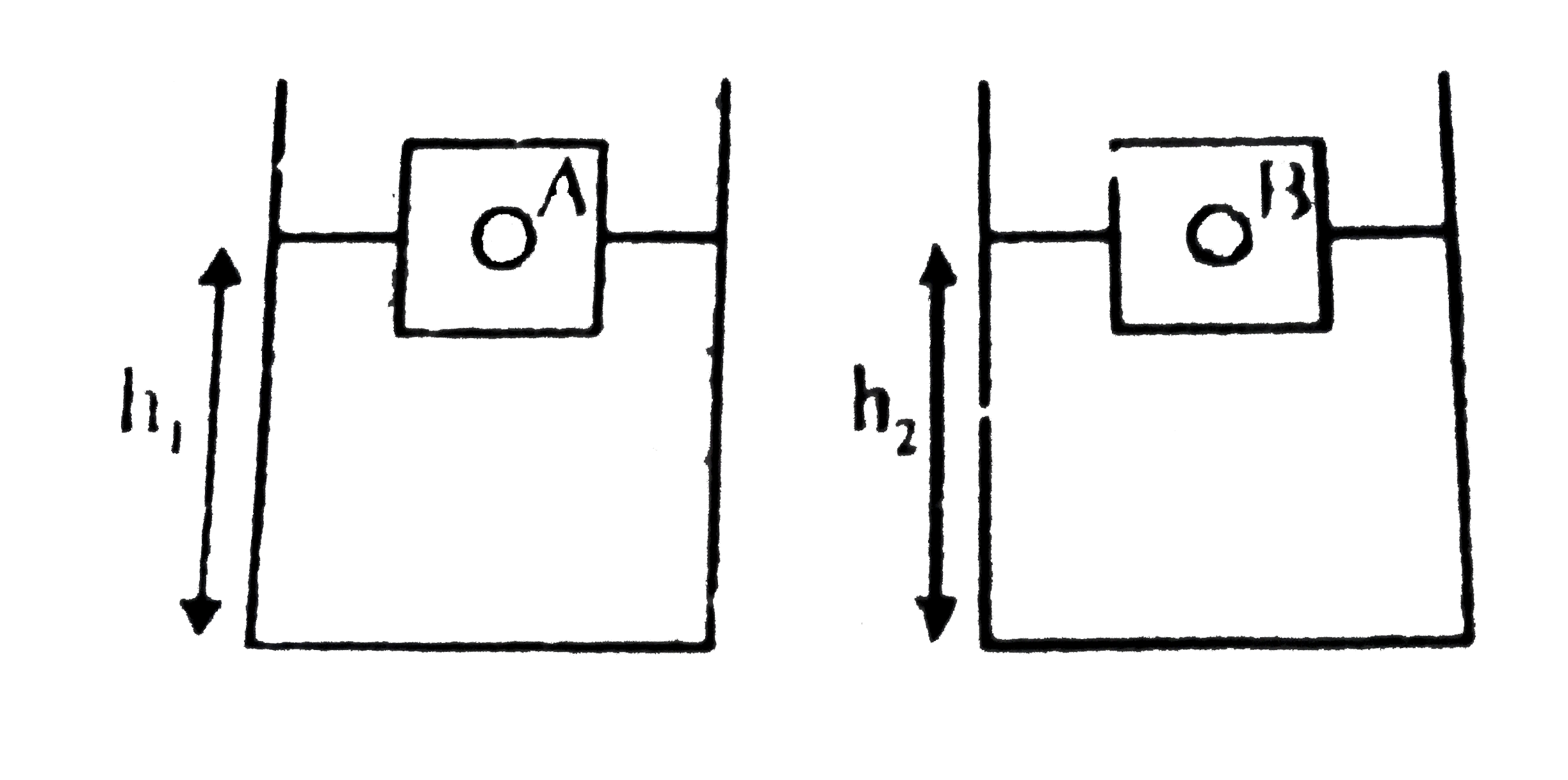 Find the ratio of initial submerged volumes of the blocks containing A and B respectively
Find the ratio of initial submerged volumes of the blocks containing A and B respectively
View SolutionIn the figure, blocks A, B and C of mass m each have acceleration a1, a2 and a3 respectively. F1 and F2 are external forces of magnitudes 2mg and mg respectively, then :
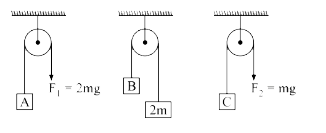
View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities 2ρ and ρ as shown in the figure is
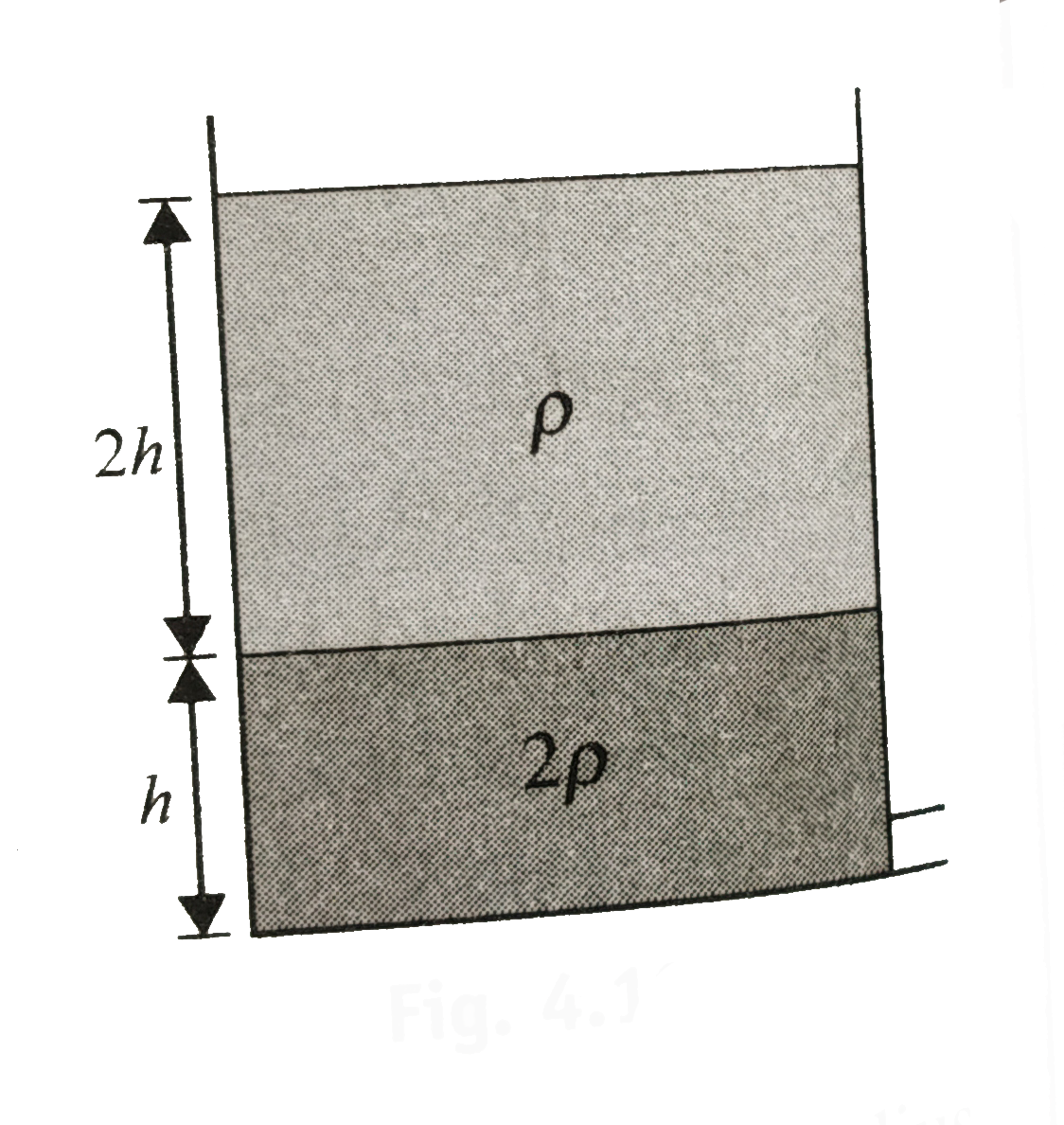
View Solution
