A boy whose eyes are 150 cm above the ground looks at his reflection in a vertical mirror 200 cm away. The top and bottom of the mirror are 220 cm and 120 cm above the ground respectively. What length below his eyes can he see, of himself in the mirror?
60 cm
75 cm
100 cm
120 cm
The correct Answer is:A
To solve the problem step by step, we will analyze the situation involving the boy, his eyes, and the mirror.
Step 1: Understand the Setup
- The boy's eyes are 150 cm above the ground.
- The mirror is located 200 cm away from the boy.
- The top of the mirror is at 220 cm and the bottom of the mirror is at 120 cm above the ground.
Step 2: Determine the Height of the Mirror
- The height of the mirror can be calculated as:
Height of the mirror=Top of the mirror−Bottom of the mirror=220cm−120cm=100cm
Step 3: Identify the Relevant Points
- The boy's eye level is at 150 cm.
- The bottom of the mirror is at 120 cm.
- The distance from the boy's eyes to the bottom of the mirror is:
Distance from eyes to bottom of mirror=150cm−120cm=30cm
Step 4: Reflection and Visibility
- To see his reflection in the mirror, the boy can see the area below his eye level.
- The angle of incidence equals the angle of reflection. Therefore, the distance he can see below his eye level in the mirror will be the same as the distance from his eyes to the bottom of the mirror.
Step 5: Calculate the Length Below His Eyes
- Since the distance from the boy's eyes to the bottom of the mirror is 30 cm, he can see 30 cm below his eyes.
- The total length of the body he can see in the mirror is:
Total length visible=Distance from eyes to bottom of mirror+Distance from eyes to top of mirror
- The distance from his eyes to the top of the mirror is:
Distance from eyes to top of mirror=220cm−150cm=70cm
- Therefore, the total length he can see is:
Total length visible=30cm+70cm=100cm
Step 6: Conclusion
- The boy can see a total length of 60 cm below his eyes in the mirror.
Final Answer
The length below his eyes that he can see in the mirror is 60 cm.
- The boy's eyes are 150 cm above the ground.
- The mirror is located 200 cm away from the boy.
- The top of the mirror is at 220 cm and the bottom of the mirror is at 120 cm above the ground.
- The height of the mirror can be calculated as:
Height of the mirror=Top of the mirror−Bottom of the mirror=220cm−120cm=100cm
- The boy's eye level is at 150 cm.
- The bottom of the mirror is at 120 cm.
- The distance from the boy's eyes to the bottom of the mirror is:
Distance from eyes to bottom of mirror=150cm−120cm=30cm
- To see his reflection in the mirror, the boy can see the area below his eye level.
- The angle of incidence equals the angle of reflection. Therefore, the distance he can see below his eye level in the mirror will be the same as the distance from his eyes to the bottom of the mirror.
- Since the distance from the boy's eyes to the bottom of the mirror is 30 cm, he can see 30 cm below his eyes.
- The total length of the body he can see in the mirror is:
Total length visible=Distance from eyes to bottom of mirror+Distance from eyes to top of mirror
- The distance from his eyes to the top of the mirror is:
Distance from eyes to top of mirror=220cm−150cm=70cm
- Therefore, the total length he can see is:
Total length visible=30cm+70cm=100cm
- The boy can see a total length of 60 cm below his eyes in the mirror.
The length below his eyes that he can see in the mirror is 60 cm.
Topper's Solved these Questions
- NSO QUESTION PAPER 2019 SET BBOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - NSO QUESTION PAPER 2019 SET BEXERCISE - ACHIEVERS SECTION
1 Videos
- NSO QUESTION PAPER 2019 SET ABOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - NSO QUESTION PAPER 2019 SET AEXERCISE - ACHIEVERS SECTION
2 Videos
- NSO QUESTION PAPER 2020 SET 1BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - NSO QUESTION PAPER 2020 SET 1EXERCISE - ACHIEVERS SECTION
2 Videos
Similar Questions
A plane mirror 50cm long, is hung on a vetical wall of a room, with its lower edge 50cm above the ground. A man stands of the mirror at a distance 2m away from the mirror. If his eyes are at a height 1.8m above the ground, then the length (distance between the extreme points of the visible region perpendicular to the mirror ) of the floor visible to him due to refliection from the mirror is x26m . Find the value of x
View SolutionWhat kind of mirror can have a focal length of, -20 cm ?
View Solution
Knowledge Check
A person's eye is at a height of 1.5 m . He stands infront of a 0.3 m long plane mirror whose lower end is 0.8m above the ground. The length of the image he sees of himself is
A1.5 mB1.0 mC0.8 mD0.6 mA person's eye is at a height of 1.5m. He stands infront of a 0.3m long palane mirror which is 0.8m above the ground. The length of the image he sees of himself is:
A1.5mB1.0mC0.8mD0.6mFocal length of objective and eye piece of telescope are 200 cm and 4 cm respectively. What is the length of telescope for normal adjustment?
A196 cmB204 cmC250 cmD225 cm
A boy of height H is standing in front of a mirror, which has been fixed on the ground as shown in figure. What length of his body can the man see in the mirror? The length of the mirror is (H/2).

View SolutionRamesh places a mirror on level ground to determine the height of a pole (with traffic light fired on it). He stands at a certain distance so that he can see the top of the pole reflected from the mirror. Ramesh's eye level is 1.8 m above the ground. The distance of Ramesh and the pole from the mirror are 1.5 m and 2.5 m respectively.
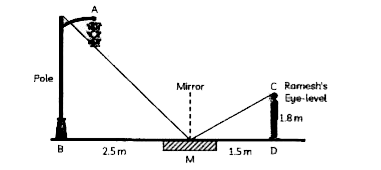
If the distance of Ramesh and the pole from the mirror are 2.5 m and 1.5 m respectively, then the height of the pole is:
View SolutionA ray of light passing through A retraces its path on reflection from a concave mirror. If PA=30cm, what is the focal length of the mirror ?
View SolutionA hair dresser stand with her nose 20 cm in front of a plane mirror for what distance must she focus her eyes in order to see her nose in the mirror?
View Solutionhair dresser stand with her nose 20 cm in front of a plane mirror for what distance must she focus her eyes in order to see her nose in the mirror?
View Solution
